0-1-xtan-1-xdx-

Question Number 77086 by Dah Solu Tion last updated on 03/Jan/20

$$\int_{\mathrm{0}} ^{\mathrm{1}} {xtan}^{−\mathrm{1}} {xdx} \\ $$$$ \\ $$

Commented by Tony Lin last updated on 03/Jan/20

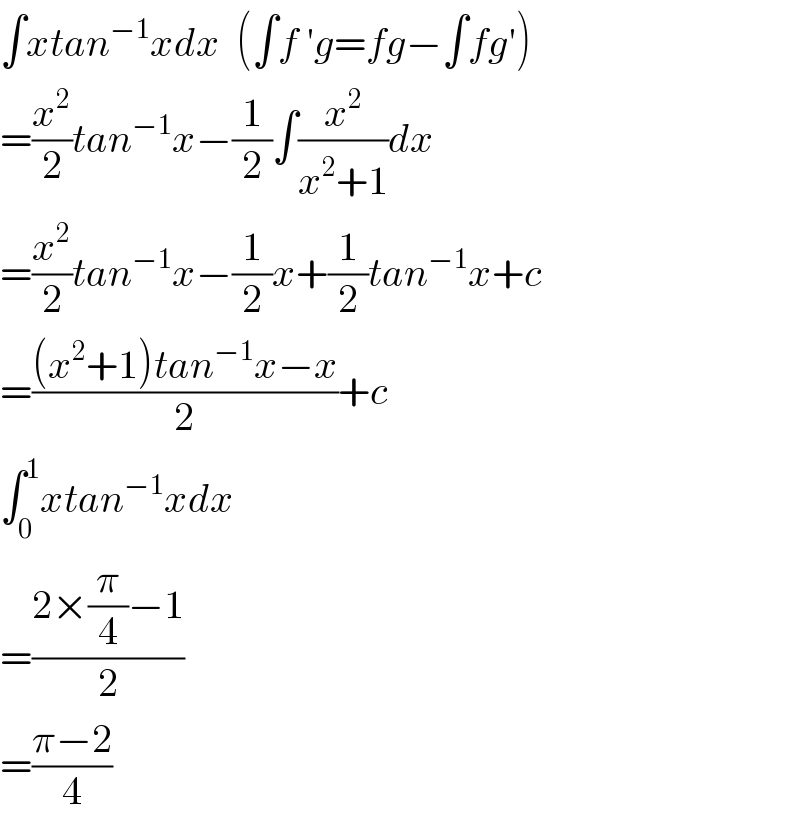
∫xtan^(−1) xdx (∫f ′g=fg−∫fg′) =(x^2 /2)tan^(−1) x−(1/2)∫(x^2 /(x^2 +1))dx =(x^2 /2)tan^(−1) x−(1/2)x+(1/2)tan^(−1) x+c =(((x^2 +1)tan^(−1) x−x)/2)+c ∫_0 ^1 xtan^(−1) xdx =((2×(π/4)−1)/2) =((π−2)/4)

$$\int{xtan}^{−\mathrm{1}} {xdx}\:\:\left(\int{f}\:'{g}={fg}−\int{fg}'\right) \\ $$$$=\frac{{x}^{\mathrm{2}} }{\mathrm{2}}{tan}^{−\mathrm{1}} {x}−\frac{\mathrm{1}}{\mathrm{2}}\int\frac{{x}^{\mathrm{2}} }{{x}^{\mathrm{2}} +\mathrm{1}}{dx} \\ $$$$=\frac{{x}^{\mathrm{2}} }{\mathrm{2}}{tan}^{−\mathrm{1}} {x}−\frac{\mathrm{1}}{\mathrm{2}}{x}+\frac{\mathrm{1}}{\mathrm{2}}{tan}^{−\mathrm{1}} {x}+{c} \\ $$$$=\frac{\left({x}^{\mathrm{2}} +\mathrm{1}\right){tan}^{−\mathrm{1}} {x}−{x}}{\mathrm{2}}+{c} \\ $$$$\int_{\mathrm{0}} ^{\mathrm{1}} {xtan}^{−\mathrm{1}} {xdx} \\ $$$$=\frac{\mathrm{2}×\frac{\pi}{\mathrm{4}}−\mathrm{1}}{\mathrm{2}} \\ $$$$=\frac{\pi−\mathrm{2}}{\mathrm{4}} \\ $$

Commented by turbo msup by abdo last updated on 03/Jan/20

let A =∫_0 ^1 xarctanxdx by parts A =[(x^2 /2) arctanx]_0 ^1 −∫_0 ^1 (x^2 /2)(dx/(1+x^2 )) =(1/2)×(π/4)−(1/2) ∫_0 ^1 ((x^2 +1−1)/(x^2 +1))dx =(π/8)−(1/2)+(1/2)[arctanx]_0 ^1 =(π/8)−(1/2)+ (π/8) =(π/4)−(1/2)

$${let}\:{A}\:=\int_{\mathrm{0}} ^{\mathrm{1}} \:{xarctanxdx}\:{by}\:{parts} \\ $$$${A}\:=\left[\frac{{x}^{\mathrm{2}} }{\mathrm{2}}\:{arctanx}\right]_{\mathrm{0}} ^{\mathrm{1}} −\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{x}^{\mathrm{2}} }{\mathrm{2}}\frac{{dx}}{\mathrm{1}+{x}^{\mathrm{2}} } \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}×\frac{\pi}{\mathrm{4}}−\frac{\mathrm{1}}{\mathrm{2}}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{{x}^{\mathrm{2}} +\mathrm{1}−\mathrm{1}}{{x}^{\mathrm{2}} \:+\mathrm{1}}{dx} \\ $$$$=\frac{\pi}{\mathrm{8}}−\frac{\mathrm{1}}{\mathrm{2}}+\frac{\mathrm{1}}{\mathrm{2}}\left[{arctanx}\right]_{\mathrm{0}} ^{\mathrm{1}} \\ $$$$=\frac{\pi}{\mathrm{8}}−\frac{\mathrm{1}}{\mathrm{2}}+\:\frac{\pi}{\mathrm{8}}\:=\frac{\pi}{\mathrm{4}}−\frac{\mathrm{1}}{\mathrm{2}} \\ $$

Answered by john santu last updated on 03/Jan/20

using integration by part u = tan^(−1) (x) ⇒du = (1/(x^2 +1)) dx dv = x dx ⇒ v = (1/2)x^2 I = u.v − ∫ v du = (1/2)x^2 tan^(−1) (x)−∫ (1/2)((x^2 /(x^2 +1)))dx = (1/2)x^2 tan^(−1) (x) −(1/2)(∫1−sec^2 t)dt , (x=tan t) = (1/2)x^2 tan^(−1) (x)−(1/2)tan^(−1) (x)+x ★ now please substuti x = 1 and x = 0. ★

$${using}\:{integration}\:{by}\:{part}\: \\ $$$${u}\:=\:\mathrm{tan}^{−\mathrm{1}} \left({x}\right)\:\Rightarrow{du}\:=\:\frac{\mathrm{1}}{{x}^{\mathrm{2}} +\mathrm{1}}\:{dx} \\ $$$${dv}\:=\:{x}\:{dx}\:\Rightarrow\:{v}\:=\:\frac{\mathrm{1}}{\mathrm{2}}{x}^{\mathrm{2}} \\ $$$${I}\:=\:{u}.{v}\:−\:\int\:{v}\:{du}\: \\ $$$$=\:\frac{\mathrm{1}}{\mathrm{2}}{x}^{\mathrm{2}} \mathrm{tan}^{−\mathrm{1}} \left({x}\right)−\int\:\frac{\mathrm{1}}{\mathrm{2}}\left(\frac{{x}^{\mathrm{2}} }{{x}^{\mathrm{2}} +\mathrm{1}}\right){dx} \\ $$$$=\:\frac{\mathrm{1}}{\mathrm{2}}{x}^{\mathrm{2}} \mathrm{tan}^{−\mathrm{1}} \left({x}\right)\:−\frac{\mathrm{1}}{\mathrm{2}}\left(\int\mathrm{1}−\mathrm{sec}\:^{\mathrm{2}} {t}\right){dt}\:,\:\left({x}=\mathrm{tan}\:{t}\right) \\ $$$$=\:\frac{\mathrm{1}}{\mathrm{2}}{x}^{\mathrm{2}} \mathrm{tan}^{−\mathrm{1}} \left({x}\right)−\frac{\mathrm{1}}{\mathrm{2}}\mathrm{tan}^{−\mathrm{1}} \left({x}\right)+{x}\:\bigstar \\ $$$${now}\:{please}\:{substuti}\:{x}\:=\:\mathrm{1}\:{and}\:{x}\:=\:\mathrm{0}.\:\bigstar \\ $$

Facebook Tweet Pin

Leave a Reply Cancel reply