Menu Close

0-e-st-sinh-atdt-or-1-s-e-st-sinh-at-0-a-s-2-e-st-coth-at-0-

Tinku Tara 0 Comments
Pin




Question Number 6099 by LMTV last updated on 13/Jun/16
∫_0 ^∞ e^(−st) sinh atdt=? or... −(1/s)[e^(−st) sinh at]_0 ^∞ =? −(a/s^2 )[e^(−st) coth at]_0 ^∞ =?
$$\int_{\mathrm{0}} ^{\infty} {e}^{−{st}} \mathrm{sinh}\:{atdt}=? \\ $$$$\mathrm{or}… \\ $$$$−\frac{\mathrm{1}}{{s}}\left[{e}^{−{st}} \mathrm{sinh}\:{at}\right]_{\mathrm{0}} ^{\infty} =? \\ $$$$−\frac{{a}}{{s}^{\mathrm{2}} }\left[{e}^{−{st}} \mathrm{coth}\:{at}\right]_{\mathrm{0}} ^{\infty} =? \\ $$
Commented by LMTV last updated on 13/Jun/16
sinh ∞=? cosh ∞=?
$$\mathrm{sinh}\:\infty=? \\ $$$$\mathrm{cosh}\:\infty=? \\ $$
Answered by Yozzii last updated on 13/Jun/16
I=∫e^(−st) sinhatdt=∫e^(−st) ((e^(at) −e^(−at) )/2)dt I=(1/2)∫(e^(t(a−s)) −e^(−t(a+s)) )dt I=(1/2)((1/(a−s))e^(t(a−s)) +(1/(a+s))e^(−t(a+s)) )+C ∴J=∫_0 ^∞ e^(−st) sinhatdt=lim_(x→∞) ∫_0 ^x e^(−st) sinhatdt ∫_0 ^∞ e^(−st) sinhatdt=(1/2)lim_(x→∞) (((exp(t(a−s)))/(a−s))+((exp(−t(a+s)))/(a+s)))∣_0 ^x J=(1/2)lim_(x→∞) (((exp(x(a−s)))/(a−s))+((exp(−x(a+s)))/(a+s))+(1/(s−a))−(1/(a+s))) J is convergent if a−s<0 and a+s>0 ⇒a<s and a>−s⇒−s<a<s⇒0≤∣a∣<s which then requires s>0. if either a−s>0 or a+s<0 ⇒a>s or a<−s⇒∣a∣>s, then J diverges since lim_(x→∞) exp(x(a−s))=exp(+∞)=+∞ or also lim_(x→∞) exp(−x(a+s))=exp(+∞)=+∞, depending on the comparison ∣a∣>s. In either case, a≠s so a−s≠0. Also,a≠−s⇒a+s≠0. If indeed 0≤∣a∣<s then J=(1/2)(((exp(−∞))/(a−s))+((exp(−∞))/(a+s))+((a+s−s+a)/(s^2 −a^2 ))) J=(a/(s^2 −a^2 )) or ∫_0 ^∞ e^(−st) sinhatdt=(a/(s^2 −a^2 )) whenever 0≤∣a∣<s.
$${I}=\int{e}^{−{st}} {sinhatdt}=\int{e}^{−{st}} \frac{{e}^{{at}} −{e}^{−{at}} }{\mathrm{2}}{dt} \\ $$$${I}=\frac{\mathrm{1}}{\mathrm{2}}\int\left({e}^{{t}\left({a}−{s}\right)} −{e}^{−{t}\left({a}+{s}\right)} \right){dt} \\ $$$${I}=\frac{\mathrm{1}}{\mathrm{2}}\left(\frac{\mathrm{1}}{{a}−{s}}{e}^{{t}\left({a}−{s}\right)} +\frac{\mathrm{1}}{{a}+{s}}{e}^{−{t}\left({a}+{s}\right)} \right)+{C} \\ $$$$\therefore{J}=\int_{\mathrm{0}} ^{\infty} {e}^{−{st}} {sinhatdt}=\underset{{x}\rightarrow\infty} {\mathrm{lim}}\int_{\mathrm{0}} ^{{x}} {e}^{−{st}} {sinhatdt} \\ $$$$\int_{\mathrm{0}} ^{\infty} {e}^{−{st}} {sinhatdt}=\frac{\mathrm{1}}{\mathrm{2}}\underset{{x}\rightarrow\infty} {\mathrm{lim}}\left(\frac{{exp}\left({t}\left({a}−{s}\right)\right)}{{a}−{s}}+\frac{{exp}\left(−{t}\left({a}+{s}\right)\right)}{{a}+{s}}\right)\mid_{\mathrm{0}} ^{{x}} \\ $$$${J}=\frac{\mathrm{1}}{\mathrm{2}}\underset{{x}\rightarrow\infty} {\mathrm{lim}}\left(\frac{{exp}\left({x}\left({a}−{s}\right)\right)}{{a}−{s}}+\frac{{exp}\left(−{x}\left({a}+{s}\right)\right)}{{a}+{s}}+\frac{\mathrm{1}}{{s}−{a}}−\frac{\mathrm{1}}{{a}+{s}}\right) \\ $$$${J}\:{is}\:{convergent}\:{if}\:{a}−{s}<\mathrm{0}\:{and}\:{a}+{s}>\mathrm{0} \\ $$$$\Rightarrow{a}<{s}\:{and}\:{a}>−{s}\Rightarrow−{s}<{a}<{s}\Rightarrow\mathrm{0}\leqslant\mid{a}\mid<{s}\: \\ $$$${which}\:{then}\:{requires}\:{s}>\mathrm{0}. \\ $$$${if}\:{either}\:{a}−{s}>\mathrm{0}\:{or}\:{a}+{s}<\mathrm{0} \\ $$$$\Rightarrow{a}>{s}\:{or}\:{a}<−{s}\Rightarrow\mid{a}\mid>{s},\:{then}\:{J}\:{diverges} \\ $$$${since}\:\underset{{x}\rightarrow\infty} {\mathrm{lim}}{exp}\left({x}\left({a}−{s}\right)\right)={exp}\left(+\infty\right)=+\infty \\ $$$${or}\:{also}\:\underset{{x}\rightarrow\infty} {\mathrm{lim}}{exp}\left(−{x}\left({a}+{s}\right)\right)={exp}\left(+\infty\right)=+\infty, \\ $$$${depending}\:{on}\:{the}\:{comparison}\:\mid{a}\mid>{s}. \\ $$$${In}\:{either}\:{case},\:{a}\neq{s}\:{so}\:{a}−{s}\neq\mathrm{0}.\:{Also},{a}\neq−{s}\Rightarrow{a}+{s}\neq\mathrm{0}. \\ $$$$ \\ $$$${If}\:{indeed}\:\mathrm{0}\leqslant\mid{a}\mid<{s}\:{then} \\ $$$${J}=\frac{\mathrm{1}}{\mathrm{2}}\left(\frac{{exp}\left(−\infty\right)}{{a}−{s}}+\frac{{exp}\left(−\infty\right)}{{a}+{s}}+\frac{{a}+{s}−{s}+{a}}{{s}^{\mathrm{2}} −{a}^{\mathrm{2}} }\right) \\ $$$${J}=\frac{{a}}{{s}^{\mathrm{2}} −{a}^{\mathrm{2}} } \\ $$$${or}\:\int_{\mathrm{0}} ^{\infty} {e}^{−{st}} {sinhatdt}=\frac{{a}}{{s}^{\mathrm{2}} −{a}^{\mathrm{2}} }\:{whenever}\:\mathrm{0}\leqslant\mid{a}\mid<{s}. \\ $$$$ \\ $$
Answered by 2closedStringsMeet last updated on 13/Jun/16
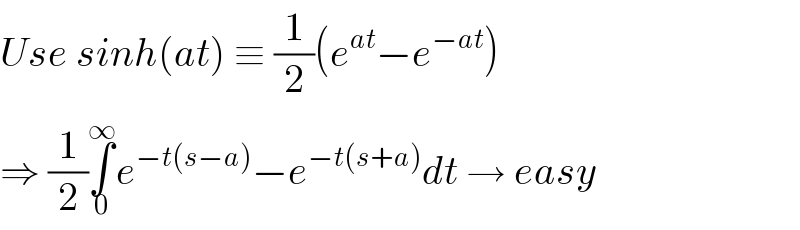
Use sinh(at) ≡ (1/2)(e^(at) −e^(−at) ) ⇒ (1/2)∫_0 ^∞ e^(−t(s−a)) −e^(−t(s+a)) dt → easy
$${Use}\:{sinh}\left({at}\right)\:\equiv\:\frac{\mathrm{1}}{\mathrm{2}}\left({e}^{{at}} −{e}^{−{at}} \right) \\ $$$$\Rightarrow\:\frac{\mathrm{1}}{\mathrm{2}}\underset{\mathrm{0}} {\overset{\infty} {\int}}{e}^{−{t}\left({s}−{a}\right)} −{e}^{−{t}\left({s}+{a}\right)} {dt}\:\rightarrow\:{easy} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *