Question Number 137059 by Dwaipayan Shikari last updated on 29/Mar/21
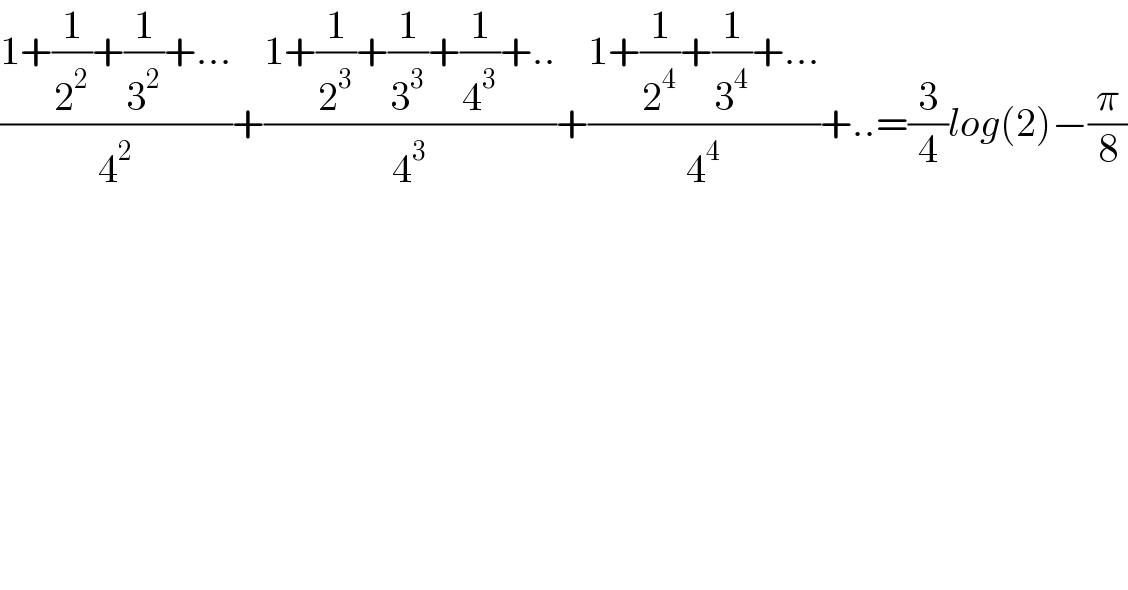
$$\frac{\mathrm{1}+\frac{\mathrm{1}}{\mathrm{2}^{\mathrm{2}} }+\frac{\mathrm{1}}{\mathrm{3}^{\mathrm{2}} }+…}{\mathrm{4}^{\mathrm{2}} }+\frac{\mathrm{1}+\frac{\mathrm{1}}{\mathrm{2}^{\mathrm{3}} }+\frac{\mathrm{1}}{\mathrm{3}^{\mathrm{3}} }+\frac{\mathrm{1}}{\mathrm{4}^{\mathrm{3}} }+..}{\mathrm{4}^{\mathrm{3}} }+\frac{\mathrm{1}+\frac{\mathrm{1}}{\mathrm{2}^{\mathrm{4}} }+\frac{\mathrm{1}}{\mathrm{3}^{\mathrm{4}} }+…}{\mathrm{4}^{\mathrm{4}} }+..=\frac{\mathrm{3}}{\mathrm{4}}{log}\left(\mathrm{2}\right)−\frac{\pi}{\mathrm{8}} \\ $$
