Question Number 5157 by 1771727373 last updated on 24/Apr/16
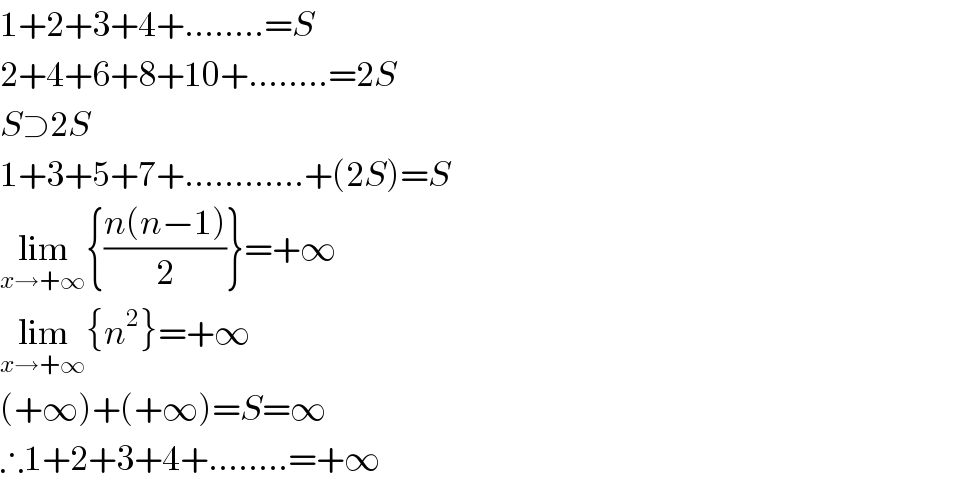
$$\mathrm{1}+\mathrm{2}+\mathrm{3}+\mathrm{4}+……..={S} \\ $$$$\mathrm{2}+\mathrm{4}+\mathrm{6}+\mathrm{8}+\mathrm{10}+……..=\mathrm{2}{S} \\ $$$${S}\supset\mathrm{2}{S} \\ $$$$\mathrm{1}+\mathrm{3}+\mathrm{5}+\mathrm{7}+…………+\left(\mathrm{2}{S}\right)={S} \\ $$$$\underset{{x}\rightarrow+\infty} {\mathrm{lim}}\left\{\frac{{n}\left({n}−\mathrm{1}\right)}{\mathrm{2}}\right\}=+\infty \\ $$$$\underset{{x}\rightarrow+\infty} {\mathrm{lim}}\left\{{n}^{\mathrm{2}} \right\}=+\infty \\ $$$$\left(+\infty\right)+\left(+\infty\right)={S}=\infty \\ $$$$\therefore\mathrm{1}+\mathrm{2}+\mathrm{3}+\mathrm{4}+……..=+\infty \\ $$
Commented by FilupSmith last updated on 24/Apr/16

$$\mathrm{This}\:\mathrm{is}\:\mathrm{both}\:\mathrm{correct}\:\mathrm{and}\:\mathrm{incorrect}. \\ $$$$ \\ $$$$\mathrm{When}\:\mathrm{looking}\:\mathrm{at}\:{S},\:\mathrm{it}\:\mathrm{does}\:\mathrm{diverge}.\:\mathrm{But} \\ $$$$\mathrm{with}\:\mathrm{analytical}\:\mathrm{continuation},\:\mathrm{you}\:\mathrm{can} \\ $$$$\mathrm{show}\:\mathrm{that}\:{S}=−\frac{\mathrm{1}}{\mathrm{12}} \\ $$$$\mathrm{This}\:\mathrm{sequence}\:\mathrm{is}\:\mathrm{also}\:\mathrm{known}\:\mathrm{as}\:\zeta\left(−\mathrm{1}\right). \\ $$$$ \\ $$$$\mathrm{I}\:\mathrm{do}\:\mathrm{not}\:\mathrm{rememberthe}\:\mathrm{proof},\:\mathrm{but}\:\mathrm{I}\:\mathrm{believe} \\ $$$$\mathrm{Yozzii}\:\mathrm{may}\:\mathrm{be}\:\mathrm{able}\:\mathrm{to}\:\mathrm{show}\:\mathrm{you} \\ $$
Commented by Yozzii last updated on 25/Apr/16

$${A}\:{test}\:{for}\:{divergence}\:{of}\:{a}\:{series}\:{given} \\ $$$${by}\:\underset{{i}=\mathrm{1}} {\overset{\infty} {\sum}}{f}\left({i}\right)\:{is}\:\underset{{n}\rightarrow\infty} {\mathrm{lim}}{f}\left({n}\right)\neq\mathrm{0}. \\ $$$${In}\:{this}\:{question}\:{f}\left({n}\right)={n} \\ $$$$\Rightarrow\underset{{n}\rightarrow\infty} {\mathrm{lim}}{f}\left({n}\right)=\underset{{n}\rightarrow\infty} {\mathrm{lim}}{n}=\infty\neq\mathrm{0}. \\ $$$$\therefore\:\mathrm{1}+\mathrm{2}+\mathrm{3}+…\:{is}\:{divergent}. \\ $$$${Since}\:{also}\:\mathrm{1}+\mathrm{2}+\mathrm{3}+…+{n}=\frac{{n}\left({n}+\mathrm{1}\right)}{\mathrm{2}} \\ $$$${and}\:\frac{{n}\left({n}+\mathrm{1}\right)}{\mathrm{2}}\:{is}\:{an}\:{increasing}\:{function} \\ $$$${for}\:{all}\:{n}\geqslant\mathrm{1},\:{then}\:\underset{{n}\rightarrow\infty} {\mathrm{lim}}\frac{{n}\left({n}+\mathrm{1}\right)}{\mathrm{2}}\:{does} \\ $$$${not}\:{exist}\:{and}\:{is}\:{arbitrarily}\:{large} \\ $$$${as}\:{n}\rightarrow\infty. \\ $$
