Question Number 536 by kth last updated on 25/Jan/15
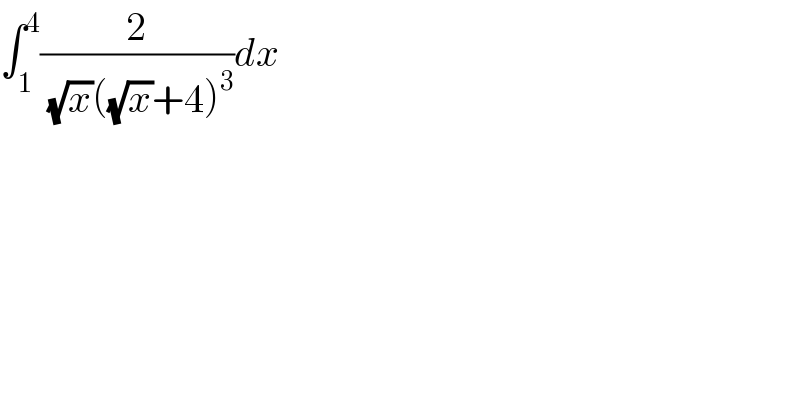
$$\int_{\mathrm{1}} ^{\mathrm{4}} \frac{\mathrm{2}}{\:\sqrt{{x}}\left(\sqrt{{x}}+\mathrm{4}\right)^{\mathrm{3}} }{dx} \\ $$$$ \\ $$
Answered by Vishal last updated on 24/Jan/15
![let (√x)+4=t then (1/( (√x))) dx=2 dt ⇒∫_(5 ) ^6 ((4 dt)/t^3 ) = 4∫_5 ^6 t^(−3) dt= 4[(1/(−2t^2 ))]_5 ^6 ⇒ 4[(1/(−72)) + (1/(50))]= ((−1)/(18)) + (2/(25)) = ((−25)/(450)) + ((36)/(450)) = (4/(50)) = (2/(25))](https://www.tinkutara.com/question/Q538.png)
$${let}\:\sqrt{{x}}+\mathrm{4}={t}\:{then}\:\frac{\mathrm{1}}{\:\sqrt{{x}}}\:{dx}=\mathrm{2}\:{dt} \\ $$$$\Rightarrow\int_{\mathrm{5}\:\:\:} ^{\mathrm{6}} \frac{\mathrm{4}\:{dt}}{{t}^{\mathrm{3}} }\:=\:\mathrm{4}\int_{\mathrm{5}} ^{\mathrm{6}} {t}^{−\mathrm{3}} {dt}=\:\mathrm{4}\left[\frac{\mathrm{1}}{−\mathrm{2}{t}^{\mathrm{2}} }\right]_{\mathrm{5}} ^{\mathrm{6}} \\ $$$$\Rightarrow\:\mathrm{4}\left[\frac{\mathrm{1}}{−\mathrm{72}}\:+\:\frac{\mathrm{1}}{\mathrm{50}}\right]=\:\frac{−\mathrm{1}}{\mathrm{18}}\:+\:\frac{\mathrm{2}}{\mathrm{25}}\:=\:\frac{−\mathrm{25}}{\mathrm{450}}\:+\:\frac{\mathrm{36}}{\mathrm{450}}\:=\:\frac{\mathrm{4}}{\mathrm{50}}\:=\:\frac{\mathrm{2}}{\mathrm{25}} \\ $$
