1-f-x-0-1-x-2t-dt-0-3-f-x-dx-2-f-x-x-2-1-x-dt-t-3-3t-2-3t-f-2019-1-

Question Number 138819 by qaz last updated on 18/Apr/21

(1) :: f (x) = \int_{0}^{1} ∣ x - 2 t ∣ d t, \int_{0}^{3} f (x) d x = ?

- - - - - - - - - - - - - - - - - - -

(2) :: f (x) = x^{2} \cdot \int_{1}^{x} \frac{d t}{t^{3} - 3 t^{2} + 3 t}, f^{(2019)} (1) = ?

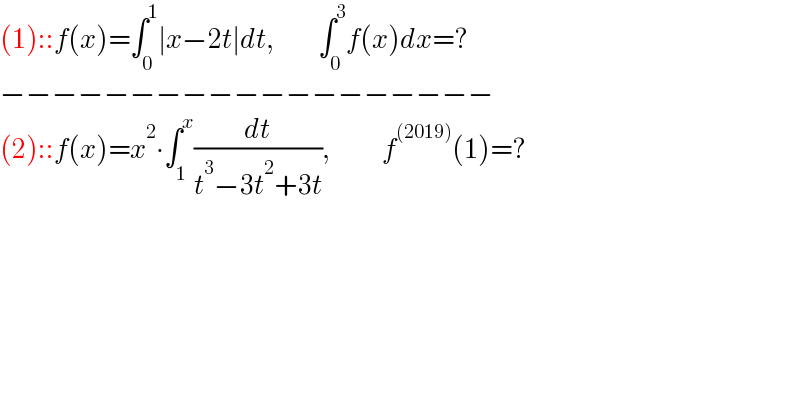
Answered by TheSupreme last updated on 18/Apr/21

\int_{0}^{1} ∣ x - 2 t ∣ d t =

x < 0 \to \int_{0}^{1} ∣ x - 2 t ∣= \int_{0}^{1} - x + 2 t d t = - x t + t^{2} = 1 - x

x > 2 \to \int_{0}^{1} ∣ x - 2 t ∣= \int_{0}^{1} x - 2 t d t = x t - t^{2} = x - 1

0 < x < 2

\int_{0}^{1} ∣ x - 2 t ∣ d t = \int_{0}^{\frac{x}{2}} x - 2 t d t + \int_{\frac{x}{2}}^{1} - x + 2 t d t =

= x t - t^{2} ∣_{0}^{\frac{x}{2}} + (- x t + t^{2}) ∣_{\frac{x}{2}}^{1} = \frac{x^{2}}{2} - \frac{x^{2}}{4} - x + 1 + \frac{x^{2}}{2} - \frac{x^{2}}{4} = \frac{x^{2}}{2} - x + 1

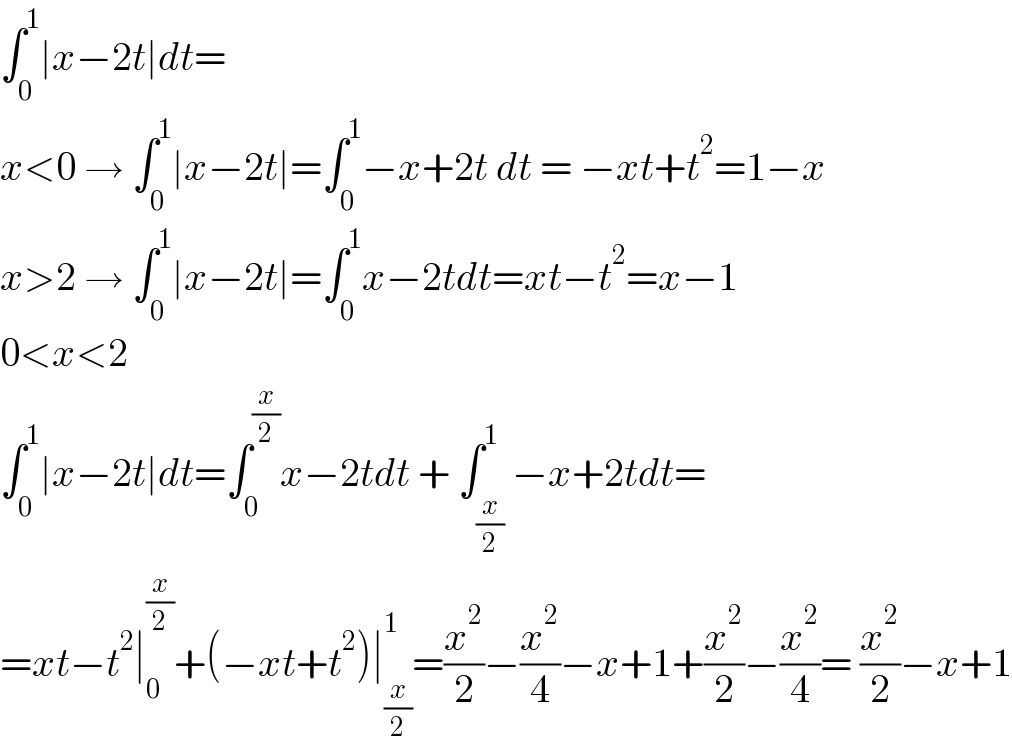
Answered by mathmax by abdo last updated on 18/Apr/21

f (x) = \int_{0}^{1} ∣ x - 2 t ∣ dt =_{2 t = u} \frac{1}{2} \int_{0}^{2} ∣ x - u ∣ du

\Rightarrow 2 f (x) = \int_{0}^{x} ∣ x - u ∣ du + \int_{x}^{2} ∣ x - u ∣ du = \int_{0}^{x} (x - u) du + \int_{x}^{2} (u - x) du

= {[xu - \frac{u^{2}}{2}]}_{u = 0}^{x} + {[\frac{u^{2}}{2} - xu]}_{x}^{2} = x^{2} - \frac{x^{2}}{2} + 2 - 2 x - \frac{x^{2}}{2} + x^{2}

= {2 x}^{2} - x^{2} + 2 - 2 x = x^{2} - 2 x + 2 \Rightarrow f (x) = \frac{x^{2}}{2} - x + 1 \Rightarrow

\int_{0}^{3} f (x) dx = \int_{0}^{3} (\frac{x^{2}}{2} - x + 1) dx = {[\frac{x^{3}}{6} - \frac{x^{2}}{2} + x]}_{0}^{3} = \frac{27}{6} - \frac{9}{2} + 3 = \dots

Answered by mathmax by abdo last updated on 18/Apr/21

f (x) = x^{2} \int_{1}^{x} \frac{dt}{t (t^{2} - 3 t + 3)} let decompose F (t) = \frac{1}{t (t^{2} - 3 t + 3)}

\Rightarrow F (t) = \frac{a}{t} + \frac{bt + c}{t^{2} - 3 t + 3} we have a = \frac{1}{3}

\lim_{t \to + \infty} tF (t) = 0 = a + b \Rightarrow b = - \frac{1}{3}

F (1) = 1 = a + b + c \Rightarrow c = 1 - 0 = 1 \Rightarrow F (x) = \frac{1}{3 t} + \frac{- \frac{t}{3} + 1}{t^{2} - 3 t + 3} \Rightarrow

\int_{1}^{x} F (t) dt = \int_{1}^{x} \frac{dt}{3 t} - \frac{1}{6} \int_{1}^{x} \frac{2 t - 3 - 3}{t^{2} - 3 t + 3} dt

= \frac{1}{3} \log ∣ x ∣ - \frac{1}{6} {[\log (t^{2} - 3 t + 3)]}_{1}^{x} + \frac{3}{6} \int_{1}^{x} \frac{dt}{t^{2} - 3 t + 3}

= \frac{1}{3} \log ∣ x ∣ - \frac{1}{6} \log (x^{2} - 3 x + 3) + \frac{3}{6} \int_{1}^{x} \frac{dt}{t^{2} - 3 t + 3}

\int_{1}^{x} \frac{dt}{t^{2} - 3 t + 3} = \int_{1}^{x} \frac{dt}{t^{2} - 2 \frac{3}{2} t + \frac{9}{4} + 3 - \frac{9}{4}}

= \int_{1}^{x} \frac{dt}{{(t - \frac{3}{2})}^{2} + \frac{3}{4}} =_{t - \frac{3}{2} = \frac{\sqrt{3}}{2} y} \frac{4}{3} \int_{- \frac{1}{\sqrt{3}}}^{\frac{2 x - 3}{\sqrt{3}}} \frac{1}{y^{2} + 1} . \frac{\sqrt{3}}{2} dy

= \frac{2}{\sqrt{3}} {[arctany]}_{- \frac{1}{\sqrt{3}}}^{\frac{2 x - 3}{\sqrt{3}}} = \frac{2}{\sqrt{3}} (\arctan (\frac{2 x - 3}{\sqrt{3}}) + \arctan (\frac{1}{\sqrt{3}}))

\Rightarrow \int_{1}^{x} F (x) dx = \frac{1}{3} \log ∣ x ∣ - \frac{1}{6} \log (x^{2} - 3 x + 3)

+ \frac{1}{\sqrt{3}} (\arctan (\frac{2 x - 3}{\sqrt{3}}) + \frac{π}{6}) \Rightarrow

f (x) = \frac{1}{3} x^{2} \log ∣ x ∣ - \frac{x^{2}}{6} \log (x^{2} - 3 x + 3) + \frac{x^{2}}{\sqrt{3}} (\arctan (\frac{2 x - 3}{\sqrt{3}}) + \frac{π}{6})

rest to calculate f^{(n)} (x) \dots . be continued \dots .

Commented by qaz last updated on 19/Apr/21

{i t}^{'} s j u s t a f i l l - i n - t h e - b l a n k s e x e r c i s e,

i d o n t t h i n k o f t h a t s o c o m p l i c a t e .

Answered by ajfour last updated on 19/Apr/21

f (x) = 2 \int_{0}^{1} \sqrt{{(t - \frac{x}{2})}^{2}} d x

= (t - \frac{x}{2}) \sqrt{{(t - \frac{x}{2})}^{2}} ∣_{0}^{1}

= (1 - \frac{x}{2}) \sqrt{{(1 - \frac{x}{2})}^{2}} + \frac{x}{2} \sqrt{\frac{x^{2}}{4}}

\int_{0}^{3} f (x) d x = \int_{0}^{2} {{(1 - \frac{x}{2})}^{2} d x

- \int_{2}^{3} {{(1 - \frac{x}{2})}^{2} d x + \int_{0}^{3} \frac{x^{2}}{4} d x

= \frac{2}{3} {(\frac{x}{2} - 1)}^{3} ∣_{0}^{2} - \frac{2}{3} {(\frac{x}{2} - 1)}^{3} ∣_{2}^{3}

+ \frac{x^{3}}{12} ∣_{0}^{3}

= \frac{2}{3} - \frac{1}{12} + \frac{9}{4} = \frac{17}{6}

Answered by ajfour last updated on 19/Apr/21

\frac{d f}{d x} = \frac{x^{2}}{x^{3} - 3 x^{2} + 3 x} + \frac{2 f}{x}

x^{2} (\frac{d f (x)}{d x}) - 2 x f (x) = \frac{x^{3}}{x^{2} - 3 x + 3}

d (\frac{f (x)}{x^{2}}) = \frac{1}{x (x^{2} - 3 x + 3)}

\frac{3}{3 x (x^{2} - 3 x + 3)} = \frac{1}{3 x} + \frac{A x + B}{x^{2} - 3 x + 3}

\Rightarrow x^{2} - 3 x + 3 + 3 {A x}^{2} + 3 B x = 3

\Rightarrow A = - \frac{1}{3}, B = 1 = \frac{3}{3}

f (x) = x^{2} {\frac{\ln x}{3} - \frac{1}{3} \int \frac{(x - 3) d x}{x^{2} - 3 x + 3} + k}

f^{1} (x) = \frac{2 x \ln x}{3} + \frac{x}{3} - \frac{x^{2} (x - 3)}{3 (x^{2} - 3 x + 3)}

+ 2 x (\frac{f (x)}{x^{2}} - \frac{\ln x}{3})

f^{1} (x) = \frac{x}{3} - \frac{x^{2} (x - 3)}{3 (x^{2} - 3 x + 3)} + \frac{2 f (x)}{x}

{x f}^{1} (x) = \frac{x^{2}}{3} - \frac{x^{2} (x^{2} - 3 x)}{3 (x^{2} - 3 x + 3)} + 2 f (x)

f^{1} (x) + {x f}^{2} (x) = \frac{2 x (x^{2} - 3 x + 3) - x^{2} (2 x - 3)}{{(x^{2} - 3 x + 3)}^{2}} + 2 f^{1} (x)

{x f}^{2} (x) = - \frac{3 x^{2}}{{(x^{2} - 3 x + 3)}^{2}} + f^{1} (x)

x {(x^{2} - 3 x + 3)}^{2} f^{2} (x) + 3 x^{2}

= {(x^{2} - 3 x + 3)}^{2} f^{1} (x)

{{(x^{2} - 3 x + 3)}^{2} + 2 x (2 x - 3) (x^{2} - 3 x + 3)} f^{2} (x)

+ x {(x^{2} - 3 x + 3)}^{2} f^{3} (x) + 6 x

= 2 (x^{2} - 3 x + 3) (2 x - 3) f^{1} (x)

+ {(x^{2} - 3 x + 3)}^{2} f^{2} (x)

\Rightarrow 2 x (2 x - 3) (x^{2} - 3 x + 3) f^{2} (x)

+ x {(x^{2} - 3 x + 3)}^{2} f^{3} (x) + 6

= 2 (x^{2} - 3 x + 3) (2 x - 3) f^{1} (x)

\Rightarrow

2 (2 x - 3) (3 x^{2}) = 6 (x^{2} - 3 x + 3)

+ x {(x^{2} - 3 x + 3)}^{2} f^{3} (x)

f^{3} (x) = \frac{6 x^{2} (2 x - 3) - 6 (x^{2} - 3 x + 3)}{x {(x^{2} - 3 x + 3)}^{2}}

= \frac{6 (2 x^{3} - 4 x^{2} + 3 x - 3)}{x {(x^{2} - 3 x + 3)}^{2}}

l e t x = t + h

f^{3} (t) = \frac{6 (2 t^{3} + 6 {h t}^{2} + 6 h^{2} t + h^{3} - 4 t^{2} - 8 h t - 4 h^{2} + 3 t + 3 h - 3)}{(t + h) {(t^{2} + 2 h t + h^{2} - 3 t - 3 h + 3)}^{2}}

l e t - 2 h^{3} + 6 h^{3} - 6 h^{3} + h^{3} - 4 h^{2} + 8 h^{2} - 4 h^{2} - 3 h + 3 h - 3 = 0

\Rightarrow - h^{3} - 3 = 0

h = - 3^{1 / 3}

\dots

Facebook Tweet Pin