Menu Close

1-sin-4-x-cos-4-x-sin-2-x-cos-2-x-dx-

Tinku Tara
Pin




Question Number 336 by Vishal Bhardwaj last updated on 25/Jan/15
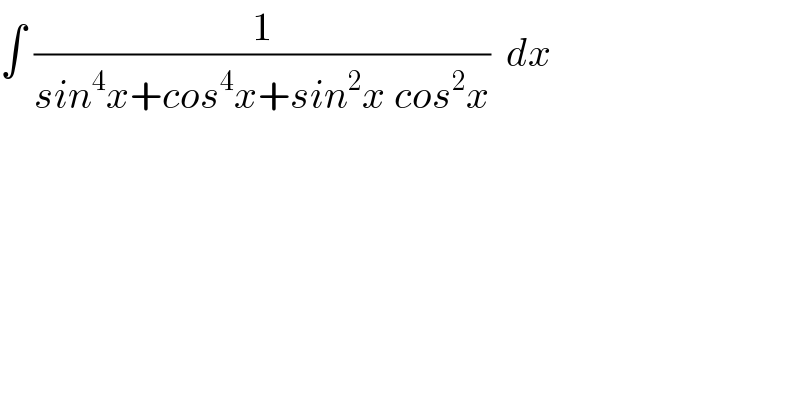
∫ (1/(sin^4 x+cos^4 x+sin^2 x cos^2 x)) dx
$$\int\:\frac{\mathrm{1}}{{sin}^{\mathrm{4}} {x}+{cos}^{\mathrm{4}} {x}+{sin}^{\mathrm{2}} {x}\:{cos}^{\mathrm{2}} {x}}\:\:{dx} \\ $$
Commented by 123456 last updated on 22/Dec/14
f(x)=(1/(sin^4 x+cos^4 x+sin^2 x cos^2 x)) =(1/(sin^4 x+cos^4 x+2sin^2 x cos^2 x−sin^2 x cos^2 x)) =(1/((sin^2 x+cos^2 x)^2 −sin^2 x cos^2 x)) =(1/(1−sin^2 x cos^2 x))
$${f}\left({x}\right)=\frac{\mathrm{1}}{\mathrm{sin}^{\mathrm{4}} {x}+\mathrm{cos}^{\mathrm{4}} {x}+\mathrm{sin}^{\mathrm{2}} {x}\:\mathrm{cos}^{\mathrm{2}} {x}} \\ $$$$=\frac{\mathrm{1}}{\mathrm{sin}^{\mathrm{4}} {x}+\mathrm{cos}^{\mathrm{4}} {x}+\mathrm{2sin}^{\mathrm{2}} {x}\:\mathrm{cos}^{\mathrm{2}} {x}−\mathrm{sin}^{\mathrm{2}} {x}\:\mathrm{cos}^{\mathrm{2}} {x}} \\ $$$$=\frac{\mathrm{1}}{\left(\mathrm{sin}^{\mathrm{2}} {x}+\mathrm{cos}^{\mathrm{2}} {x}\right)^{\mathrm{2}} −\mathrm{sin}^{\mathrm{2}} {x}\:\mathrm{cos}^{\mathrm{2}} {x}} \\ $$$$=\frac{\mathrm{1}}{\mathrm{1}−\mathrm{sin}^{\mathrm{2}} {x}\:\mathrm{cos}^{\mathrm{2}} {x}} \\ $$
Commented by 123456 last updated on 23/Dec/14
((tan^(−1) [((√3)/2)tan(2x)])/( (√3)))
$$\frac{\mathrm{tan}^{−\mathrm{1}} \left[\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\mathrm{tan}\left(\mathrm{2}{x}\right)\right]}{\:\sqrt{\mathrm{3}}} \\ $$
Answered by prakash jain last updated on 27/Dec/14
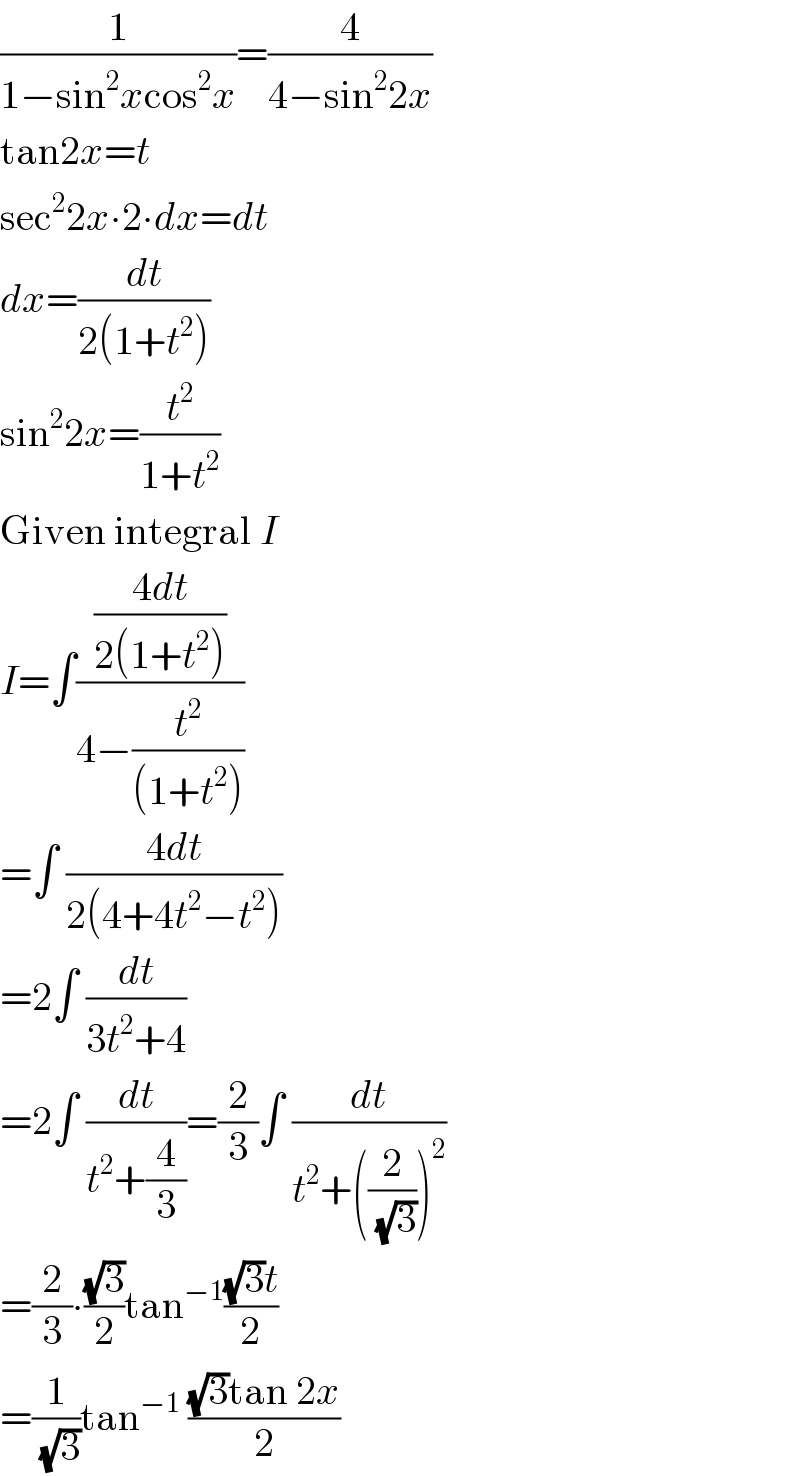
(1/(1−sin^2 xcos^2 x))=(4/(4−sin^2 2x)) tan2x=t sec^2 2x∙2∙dx=dt dx=(dt/(2(1+t^2 ))) sin^2 2x=(t^2 /(1+t^2 )) Given integral I I=∫(((4dt)/(2(1+t^2 )))/(4−(t^2 /((1+t^2 ))))) =∫ ((4dt)/(2(4+4t^2 −t^2 ))) =2∫ (dt/(3t^2 +4)) =2∫ (dt/(t^2 +(4/3)))=(2/3)∫ (dt/(t^2 +((2/( (√3))))^2 )) =(2/3)∙((√3)/2)tan^(−1) (((√3)t)/2) =(1/( (√3)))tan^(−1) (((√3)tan 2x)/2)
$$\frac{\mathrm{1}}{\mathrm{1}−\mathrm{sin}^{\mathrm{2}} {x}\mathrm{cos}^{\mathrm{2}} {x}}=\frac{\mathrm{4}}{\mathrm{4}−\mathrm{sin}^{\mathrm{2}} \mathrm{2}{x}} \\ $$$$\mathrm{tan2}{x}={t} \\ $$$$\mathrm{sec}^{\mathrm{2}} \mathrm{2}{x}\centerdot\mathrm{2}\centerdot{dx}={dt} \\ $$$${dx}=\frac{{dt}}{\mathrm{2}\left(\mathrm{1}+{t}^{\mathrm{2}} \right)} \\ $$$$\mathrm{sin}^{\mathrm{2}} \mathrm{2}{x}=\frac{{t}^{\mathrm{2}} }{\mathrm{1}+{t}^{\mathrm{2}} } \\ $$$$\mathrm{Given}\:\mathrm{integral}\:{I} \\ $$$${I}=\int\frac{\frac{\mathrm{4}{dt}}{\mathrm{2}\left(\mathrm{1}+{t}^{\mathrm{2}} \right)}}{\mathrm{4}−\frac{{t}^{\mathrm{2}} }{\left(\mathrm{1}+{t}^{\mathrm{2}} \right)}} \\ $$$$=\int\:\frac{\mathrm{4}{dt}}{\mathrm{2}\left(\mathrm{4}+\mathrm{4}{t}^{\mathrm{2}} −{t}^{\mathrm{2}} \right)} \\ $$$$=\mathrm{2}\int\:\frac{{dt}}{\mathrm{3}{t}^{\mathrm{2}} +\mathrm{4}} \\ $$$$=\mathrm{2}\int\:\frac{{dt}}{{t}^{\mathrm{2}} +\frac{\mathrm{4}}{\mathrm{3}}}=\frac{\mathrm{2}}{\mathrm{3}}\int\:\frac{{dt}}{{t}^{\mathrm{2}} +\left(\frac{\mathrm{2}}{\:\sqrt{\mathrm{3}}}\right)^{\mathrm{2}} } \\ $$$$=\frac{\mathrm{2}}{\mathrm{3}}\centerdot\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\mathrm{tan}^{−\mathrm{1}} \frac{\sqrt{\mathrm{3}}{t}}{\mathrm{2}} \\ $$$$=\frac{\mathrm{1}}{\:\sqrt{\mathrm{3}}}\mathrm{tan}^{−\mathrm{1}} \:\frac{\sqrt{\mathrm{3}}\mathrm{tan}\:\mathrm{2}{x}}{\mathrm{2}} \\ $$

Pin