Menu Close

1-x-2-x-2-9-dx-

Tinku Tara 0 Comments
Pin




Question Number 136313 by aurpeyz last updated on 20/Mar/21
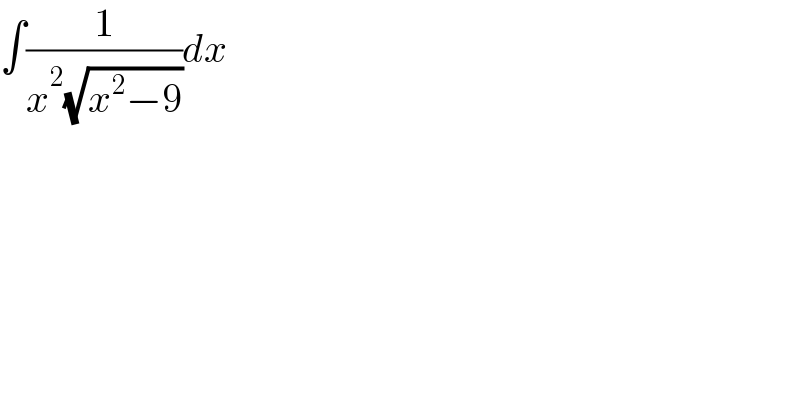
∫(1/(x^2 (√(x^2 −9))))dx
$$\int\frac{\mathrm{1}}{{x}^{\mathrm{2}} \sqrt{{x}^{\mathrm{2}} −\mathrm{9}}}{dx} \\ $$
Answered by liberty last updated on 20/Mar/21
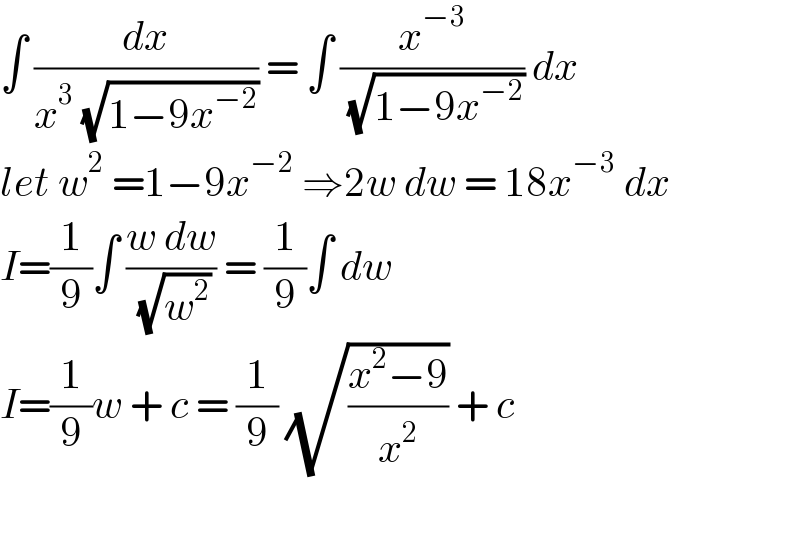
∫ (dx/(x^3 (√(1−9x^(−2) )))) = ∫ (x^(−3) /( (√(1−9x^(−2) )))) dx let w^2 =1−9x^(−2) ⇒2w dw = 18x^(−3) dx I=(1/9)∫ ((w dw)/( (√w^2 ))) = (1/9)∫ dw I=(1/9)w + c = (1/9) (√((x^2 −9)/x^2 )) + c
$$\int\:\frac{{dx}}{{x}^{\mathrm{3}} \:\sqrt{\mathrm{1}−\mathrm{9}{x}^{−\mathrm{2}} }}\:=\:\int\:\frac{{x}^{−\mathrm{3}} }{\:\sqrt{\mathrm{1}−\mathrm{9}{x}^{−\mathrm{2}} }}\:{dx}\: \\ $$$${let}\:{w}^{\mathrm{2}} \:=\mathrm{1}−\mathrm{9}{x}^{−\mathrm{2}} \:\Rightarrow\mathrm{2}{w}\:{dw}\:=\:\mathrm{18}{x}^{−\mathrm{3}} \:{dx} \\ $$$${I}=\frac{\mathrm{1}}{\mathrm{9}}\int\:\frac{{w}\:{dw}}{\:\sqrt{{w}^{\mathrm{2}} }}\:=\:\frac{\mathrm{1}}{\mathrm{9}}\int\:{dw}\: \\ $$$${I}=\frac{\mathrm{1}}{\mathrm{9}}{w}\:+\:{c}\:=\:\frac{\mathrm{1}}{\mathrm{9}}\:\sqrt{\frac{{x}^{\mathrm{2}} −\mathrm{9}}{{x}^{\mathrm{2}} }}\:+\:{c} \\ $$$$ \\ $$
Answered by Olaf last updated on 20/Mar/21
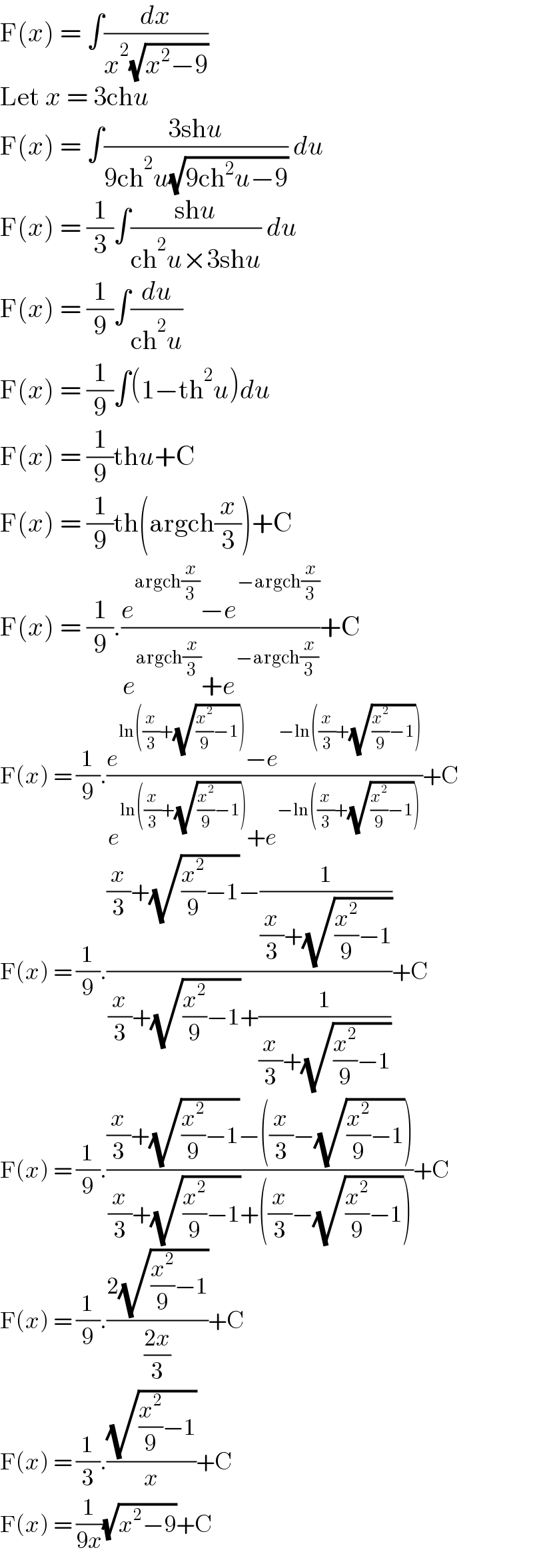
F(x) = ∫(dx/(x^2 (√(x^2 −9)))) Let x = 3chu F(x) = ∫((3shu)/(9ch^2 u(√(9ch^2 u−9)))) du F(x) = (1/3)∫((shu)/(ch^2 u×3shu)) du F(x) = (1/9)∫(du/(ch^2 u)) F(x) = (1/9)∫(1−th^2 u)du F(x) = (1/9)thu+C F(x) = (1/9)th(argch(x/3))+C F(x) = (1/9).((e^(argch(x/3)) −e^(−argch(x/3)) )/(e^(argch(x/3)) +e^(−argch(x/3)) ))+C F(x) = (1/9).((e^(ln((x/3)+(√((x^2 /9)−1)))) −e^(−ln((x/3)+(√((x^2 /9)−1)))) )/(e^(ln((x/3)+(√((x^2 /9)−1)))) +e^(−ln((x/3)+(√((x^2 /9)−1)))) ))+C F(x) = (1/9).(((x/3)+(√((x^2 /9)−1))−(1/((x/3)+(√((x^2 /9)−1)))))/((x/3)+(√((x^2 /9)−1))+(1/((x/3)+(√((x^2 /9)−1))))))+C F(x) = (1/9).(((x/3)+(√((x^2 /9)−1))−((x/3)−(√((x^2 /9)−1))))/((x/3)+(√((x^2 /9)−1))+((x/3)−(√((x^2 /9)−1)))))+C F(x) = (1/9).((2(√((x^2 /9)−1)))/((2x)/3))+C F(x) = (1/3).((√((x^2 /9)−1))/x)+C F(x) = (1/(9x))(√(x^2 −9))+C
$$\mathrm{F}\left({x}\right)\:=\:\int\frac{{dx}}{{x}^{\mathrm{2}} \sqrt{{x}^{\mathrm{2}} −\mathrm{9}}} \\ $$$$\mathrm{Let}\:{x}\:=\:\mathrm{3ch}{u} \\ $$$$\mathrm{F}\left({x}\right)\:=\:\int\frac{\mathrm{3sh}{u}}{\mathrm{9ch}^{\mathrm{2}} {u}\sqrt{\mathrm{9ch}^{\mathrm{2}} {u}−\mathrm{9}}}\:{du} \\ $$$$\mathrm{F}\left({x}\right)\:=\:\frac{\mathrm{1}}{\mathrm{3}}\int\frac{\mathrm{sh}{u}}{\mathrm{ch}^{\mathrm{2}} {u}×\mathrm{3sh}{u}}\:{du} \\ $$$$\mathrm{F}\left({x}\right)\:=\:\frac{\mathrm{1}}{\mathrm{9}}\int\frac{{du}}{\mathrm{ch}^{\mathrm{2}} {u}}\: \\ $$$$\mathrm{F}\left({x}\right)\:=\:\frac{\mathrm{1}}{\mathrm{9}}\int\left(\mathrm{1}−\mathrm{th}^{\mathrm{2}} {u}\right){du} \\ $$$$\mathrm{F}\left({x}\right)\:=\:\frac{\mathrm{1}}{\mathrm{9}}\mathrm{th}{u}+\mathrm{C} \\ $$$$\mathrm{F}\left({x}\right)\:=\:\frac{\mathrm{1}}{\mathrm{9}}\mathrm{th}\left(\mathrm{argch}\frac{{x}}{\mathrm{3}}\right)+\mathrm{C} \\ $$$$\mathrm{F}\left({x}\right)\:=\:\frac{\mathrm{1}}{\mathrm{9}}.\frac{{e}^{\mathrm{argch}\frac{{x}}{\mathrm{3}}} −{e}^{−\mathrm{argch}\frac{{x}}{\mathrm{3}}} }{{e}^{\mathrm{argch}\frac{{x}}{\mathrm{3}}} +{e}^{−\mathrm{argch}\frac{{x}}{\mathrm{3}}} }+\mathrm{C} \\ $$$$\mathrm{F}\left({x}\right)\:=\:\frac{\mathrm{1}}{\mathrm{9}}.\frac{{e}^{\mathrm{ln}\left(\frac{{x}}{\mathrm{3}}+\sqrt{\frac{{x}^{\mathrm{2}} }{\mathrm{9}}−\mathrm{1}}\right)} −{e}^{−\mathrm{ln}\left(\frac{{x}}{\mathrm{3}}+\sqrt{\frac{{x}^{\mathrm{2}} }{\mathrm{9}}−\mathrm{1}}\right)} }{{e}^{\mathrm{ln}\left(\frac{{x}}{\mathrm{3}}+\sqrt{\frac{{x}^{\mathrm{2}} }{\mathrm{9}}−\mathrm{1}}\right)} +{e}^{−\mathrm{ln}\left(\frac{{x}}{\mathrm{3}}+\sqrt{\frac{{x}^{\mathrm{2}} }{\mathrm{9}}−\mathrm{1}}\right)} }+\mathrm{C} \\ $$$$\mathrm{F}\left({x}\right)\:=\:\frac{\mathrm{1}}{\mathrm{9}}.\frac{\frac{{x}}{\mathrm{3}}+\sqrt{\frac{{x}^{\mathrm{2}} }{\mathrm{9}}−\mathrm{1}}−\frac{\mathrm{1}}{\frac{{x}}{\mathrm{3}}+\sqrt{\frac{{x}^{\mathrm{2}} }{\mathrm{9}}−\mathrm{1}}}}{\frac{{x}}{\mathrm{3}}+\sqrt{\frac{{x}^{\mathrm{2}} }{\mathrm{9}}−\mathrm{1}}+\frac{\mathrm{1}}{\frac{{x}}{\mathrm{3}}+\sqrt{\frac{{x}^{\mathrm{2}} }{\mathrm{9}}−\mathrm{1}}}}+\mathrm{C} \\ $$$$\mathrm{F}\left({x}\right)\:=\:\frac{\mathrm{1}}{\mathrm{9}}.\frac{\frac{{x}}{\mathrm{3}}+\sqrt{\frac{{x}^{\mathrm{2}} }{\mathrm{9}}−\mathrm{1}}−\left(\frac{{x}}{\mathrm{3}}−\sqrt{\frac{{x}^{\mathrm{2}} }{\mathrm{9}}−\mathrm{1}}\right)}{\frac{{x}}{\mathrm{3}}+\sqrt{\frac{{x}^{\mathrm{2}} }{\mathrm{9}}−\mathrm{1}}+\left(\frac{{x}}{\mathrm{3}}−\sqrt{\frac{{x}^{\mathrm{2}} }{\mathrm{9}}−\mathrm{1}}\right)}+\mathrm{C} \\ $$$$\mathrm{F}\left({x}\right)\:=\:\frac{\mathrm{1}}{\mathrm{9}}.\frac{\mathrm{2}\sqrt{\frac{{x}^{\mathrm{2}} }{\mathrm{9}}−\mathrm{1}}}{\frac{\mathrm{2}{x}}{\mathrm{3}}}+\mathrm{C} \\ $$$$\mathrm{F}\left({x}\right)\:=\:\frac{\mathrm{1}}{\mathrm{3}}.\frac{\sqrt{\frac{{x}^{\mathrm{2}} }{\mathrm{9}}−\mathrm{1}}}{{x}}+\mathrm{C} \\ $$$$\mathrm{F}\left({x}\right)\:=\:\frac{\mathrm{1}}{\mathrm{9}{x}}\sqrt{{x}^{\mathrm{2}} −\mathrm{9}}+\mathrm{C} \\ $$
Answered by mathmax by abdo last updated on 20/Mar/21
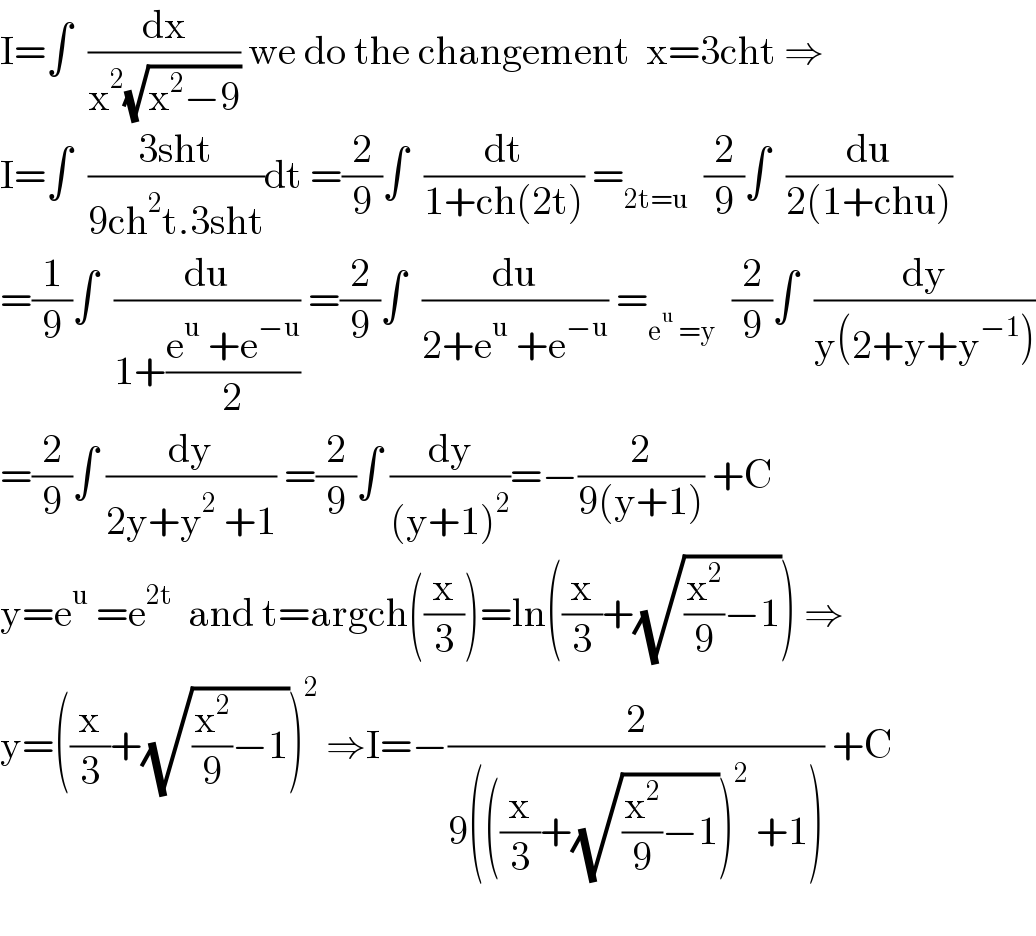
I=∫ (dx/(x^2 (√(x^2 −9)))) we do the changement x=3cht ⇒ I=∫ ((3sht)/(9ch^2 t.3sht))dt =(2/9)∫ (dt/(1+ch(2t))) =_(2t=u) (2/9)∫ (du/(2(1+chu))) =(1/9)∫ (du/(1+((e^u +e^(−u) )/2))) =(2/9)∫ (du/(2+e^u +e^(−u) )) =_(e^u =y) (2/9)∫ (dy/(y(2+y+y^(−1) ))) =(2/9)∫ (dy/(2y+y^2 +1)) =(2/9)∫ (dy/((y+1)^2 ))=−(2/(9(y+1))) +C y=e^u =e^(2t) and t=argch((x/3))=ln((x/3)+(√((x^2 /9)−1))) ⇒ y=((x/3)+(√((x^2 /9)−1)))^2 ⇒I=−(2/(9(((x/3)+(√((x^2 /9)−1)))^2 +1))) +C
$$\mathrm{I}=\int\:\:\frac{\mathrm{dx}}{\mathrm{x}^{\mathrm{2}} \sqrt{\mathrm{x}^{\mathrm{2}} −\mathrm{9}}}\:\mathrm{we}\:\mathrm{do}\:\mathrm{the}\:\mathrm{changement}\:\:\mathrm{x}=\mathrm{3cht}\:\Rightarrow \\ $$$$\mathrm{I}=\int\:\:\frac{\mathrm{3sht}}{\mathrm{9ch}^{\mathrm{2}} \mathrm{t}.\mathrm{3sht}}\mathrm{dt}\:=\frac{\mathrm{2}}{\mathrm{9}}\int\:\:\frac{\mathrm{dt}}{\mathrm{1}+\mathrm{ch}\left(\mathrm{2t}\right)}\:=_{\mathrm{2t}=\mathrm{u}} \:\:\frac{\mathrm{2}}{\mathrm{9}}\int\:\:\frac{\mathrm{du}}{\mathrm{2}\left(\mathrm{1}+\mathrm{chu}\right)} \\ $$$$=\frac{\mathrm{1}}{\mathrm{9}}\int\:\:\frac{\mathrm{du}}{\mathrm{1}+\frac{\mathrm{e}^{\mathrm{u}} \:+\mathrm{e}^{−\mathrm{u}} }{\mathrm{2}}}\:=\frac{\mathrm{2}}{\mathrm{9}}\int\:\:\frac{\mathrm{du}}{\mathrm{2}+\mathrm{e}^{\mathrm{u}} \:+\mathrm{e}^{−\mathrm{u}} }\:=_{\mathrm{e}^{\mathrm{u}} \:=\mathrm{y}} \:\:\frac{\mathrm{2}}{\mathrm{9}}\int\:\:\frac{\mathrm{dy}}{\mathrm{y}\left(\mathrm{2}+\mathrm{y}+\mathrm{y}^{−\mathrm{1}} \right)} \\ $$$$=\frac{\mathrm{2}}{\mathrm{9}}\int\:\frac{\mathrm{dy}}{\mathrm{2y}+\mathrm{y}^{\mathrm{2}} \:+\mathrm{1}}\:=\frac{\mathrm{2}}{\mathrm{9}}\int\:\frac{\mathrm{dy}}{\left(\mathrm{y}+\mathrm{1}\right)^{\mathrm{2}} }=−\frac{\mathrm{2}}{\mathrm{9}\left(\mathrm{y}+\mathrm{1}\right)}\:+\mathrm{C} \\ $$$$\mathrm{y}=\mathrm{e}^{\mathrm{u}} \:=\mathrm{e}^{\mathrm{2t}} \:\:\mathrm{and}\:\mathrm{t}=\mathrm{argch}\left(\frac{\mathrm{x}}{\mathrm{3}}\right)=\mathrm{ln}\left(\frac{\mathrm{x}}{\mathrm{3}}+\sqrt{\frac{\mathrm{x}^{\mathrm{2}} }{\mathrm{9}}−\mathrm{1}}\right)\:\Rightarrow \\ $$$$\mathrm{y}=\left(\frac{\mathrm{x}}{\mathrm{3}}+\sqrt{\frac{\mathrm{x}^{\mathrm{2}} }{\mathrm{9}}−\mathrm{1}}\right)^{\mathrm{2}} \:\Rightarrow\mathrm{I}=−\frac{\mathrm{2}}{\mathrm{9}\left(\left(\frac{\mathrm{x}}{\mathrm{3}}+\sqrt{\frac{\mathrm{x}^{\mathrm{2}} }{\mathrm{9}}−\mathrm{1}}\right)^{\mathrm{2}} \:+\mathrm{1}\right)}\:+\mathrm{C} \\ $$$$ \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *