Menu Close

10-25-28-125-82-625-

Tinku Tara 0 Comments
Pin




Question Number 143184 by bramlexs22 last updated on 11/Jun/21
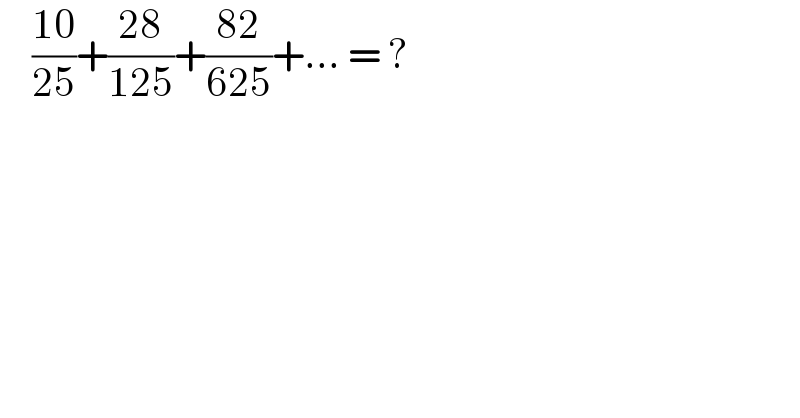
((10)/(25))+((28)/(125))+((82)/(625))+... = ?
$$\:\:\:\:\frac{\mathrm{10}}{\mathrm{25}}+\frac{\mathrm{28}}{\mathrm{125}}+\frac{\mathrm{82}}{\mathrm{625}}+…\:=\:? \\ $$
Answered by Canebulok last updated on 11/Jun/21
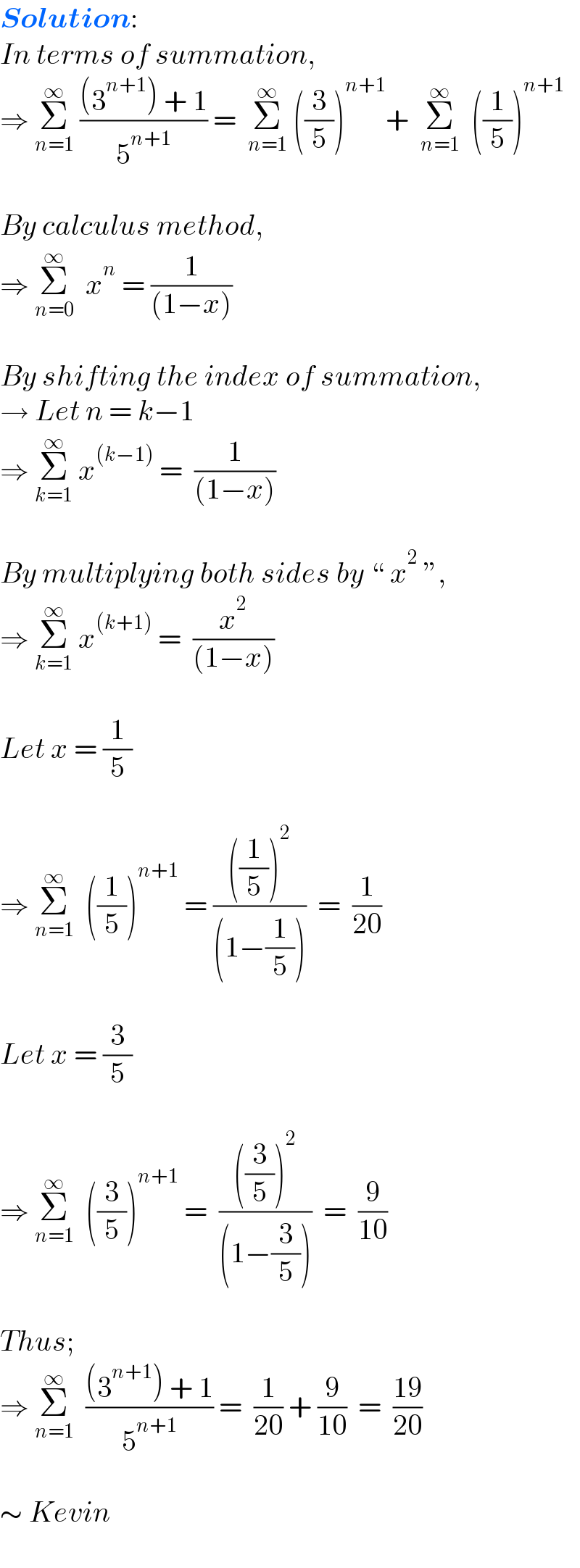
Solution: In terms of summation, ⇒ Σ_(n=1) ^∞ (((3^(n+1) ) + 1)/5^(n+1) ) = Σ_(n=1) ^∞ ((3/5))^(n+1) + Σ_(n=1) ^∞ ((1/5))^(n+1) By calculus method, ⇒ Σ_(n=0) ^∞ x^n = (1/((1−x))) By shifting the index of summation, → Let n = k−1 ⇒ Σ_(k=1) ^∞ x^((k−1)) = (1/((1−x))) By multiplying both sides by “ x^2 ”, ⇒ Σ_(k=1) ^∞ x^((k+1)) = (x^2 /((1−x))) Let x = (1/5) ⇒ Σ_(n=1) ^∞ ((1/5))^(n+1) = ((((1/5))^2 )/((1−(1/5)))) = (1/(20)) Let x = (3/5) ⇒ Σ_(n=1) ^∞ ((3/5))^(n+1) = ((((3/5))^2 )/((1−(3/5)))) = (9/(10)) Thus; ⇒ Σ_(n=1) ^∞ (((3^(n+1) ) + 1)/5^(n+1) ) = (1/(20)) + (9/(10)) = ((19)/(20)) ∼ Kevin
$$\boldsymbol{{Solution}}: \\ $$$${In}\:{terms}\:{of}\:{summation}, \\ $$$$\Rightarrow\:\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\:\frac{\left(\mathrm{3}^{{n}+\mathrm{1}} \right)\:+\:\mathrm{1}}{\mathrm{5}^{{n}+\mathrm{1}} }\:=\:\:\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\:\left(\frac{\mathrm{3}}{\mathrm{5}}\right)^{{n}+\mathrm{1}} +\:\:\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\:\:\left(\frac{\mathrm{1}}{\mathrm{5}}\right)^{{n}+\mathrm{1}} \: \\ $$$$\: \\ $$$${By}\:{calculus}\:{method}, \\ $$$$\Rightarrow\:\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}\:\:{x}^{{n}} \:=\:\frac{\mathrm{1}}{\left(\mathrm{1}−{x}\right)} \\ $$$$\: \\ $$$${By}\:{shifting}\:{the}\:{index}\:{of}\:{summation}, \\ $$$$\rightarrow\:{Let}\:{n}\:=\:{k}−\mathrm{1} \\ $$$$\Rightarrow\:\underset{{k}=\mathrm{1}} {\overset{\infty} {\sum}}\:{x}^{\left({k}−\mathrm{1}\right)} \:=\:\:\frac{\mathrm{1}}{\left(\mathrm{1}−{x}\right)} \\ $$$$\: \\ $$$${By}\:{multiplying}\:{both}\:{sides}\:{by}\:“\:{x}^{\mathrm{2}} \:'', \\ $$$$\Rightarrow\:\underset{{k}=\mathrm{1}} {\overset{\infty} {\sum}}\:{x}^{\left({k}+\mathrm{1}\right)} \:=\:\:\frac{{x}^{\mathrm{2}} }{\left(\mathrm{1}−{x}\right)} \\ $$$$\: \\ $$$${Let}\:{x}\:=\:\frac{\mathrm{1}}{\mathrm{5}} \\ $$$$\: \\ $$$$\Rightarrow\:\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\:\:\left(\frac{\mathrm{1}}{\mathrm{5}}\right)^{{n}+\mathrm{1}} \:=\:\frac{\left(\frac{\mathrm{1}}{\mathrm{5}}\right)^{\mathrm{2}} }{\left(\mathrm{1}−\frac{\mathrm{1}}{\mathrm{5}}\right)}\:\:=\:\:\frac{\mathrm{1}}{\mathrm{20}} \\ $$$$\: \\ $$$${Let}\:{x}\:=\:\frac{\mathrm{3}}{\mathrm{5}} \\ $$$$\: \\ $$$$\Rightarrow\:\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\:\:\left(\frac{\mathrm{3}}{\mathrm{5}}\right)^{{n}+\mathrm{1}} \:=\:\:\frac{\left(\frac{\mathrm{3}}{\mathrm{5}}\right)^{\mathrm{2}} }{\left(\mathrm{1}−\frac{\mathrm{3}}{\mathrm{5}}\right)}\:\:=\:\:\frac{\mathrm{9}}{\mathrm{10}} \\ $$$$\: \\ $$$${Thus}; \\ $$$$\Rightarrow\:\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\:\:\frac{\left(\mathrm{3}^{{n}+\mathrm{1}} \right)\:+\:\mathrm{1}}{\mathrm{5}^{{n}+\mathrm{1}} }\:=\:\:\frac{\mathrm{1}}{\mathrm{20}}\:+\:\frac{\mathrm{9}}{\mathrm{10}}\:\:=\:\:\frac{\mathrm{19}}{\mathrm{20}} \\ $$$$\: \\ $$$$\sim\:{Kevin} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *