Question Number 12402 by sin (x) last updated on 21/Apr/17
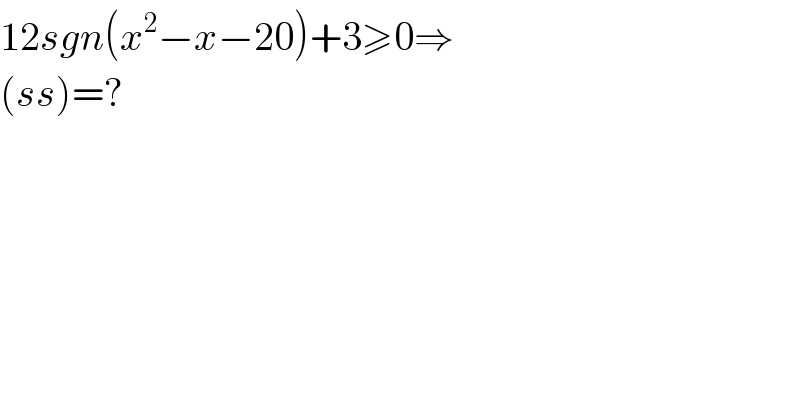
$$\mathrm{12}{sgn}\left({x}^{\mathrm{2}} −{x}−\mathrm{20}\right)+\mathrm{3}\geqslant\mathrm{0}\Rightarrow \\ $$$$\left({ss}\right)=? \\ $$
Commented by FilupS last updated on 21/Apr/17
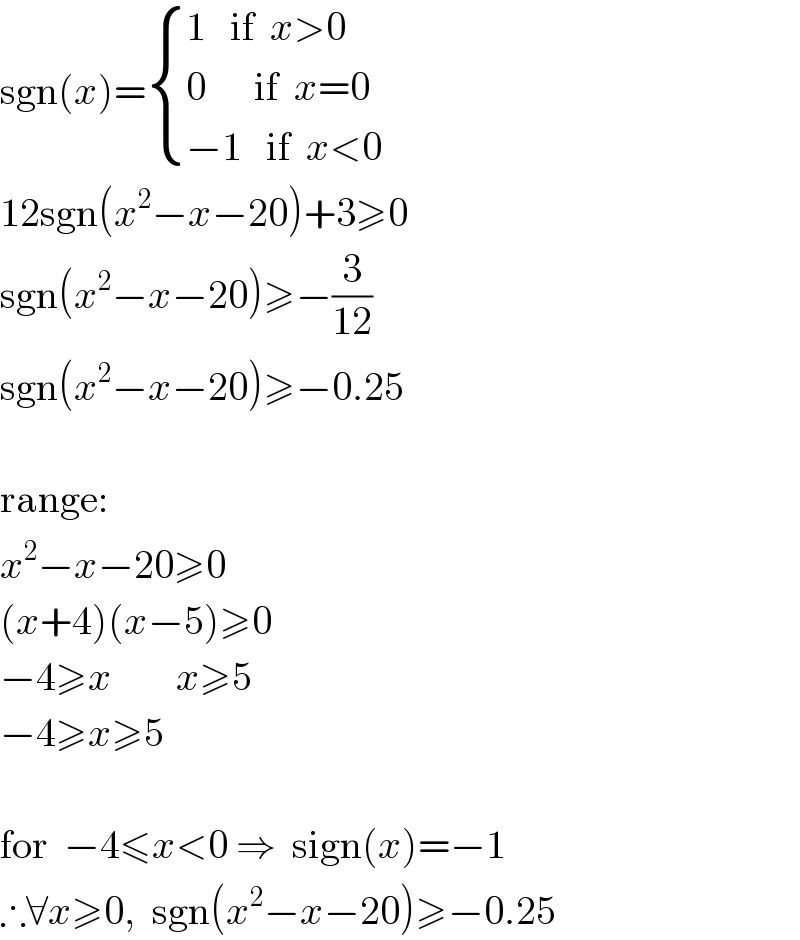
$$\mathrm{sgn}\left({x}\right)=\begin{cases}{\mathrm{1}\:\:\:\mathrm{if}\:\:{x}>\mathrm{0}}\\{\mathrm{0}\:\:\:\:\:\:\mathrm{if}\:\:{x}=\mathrm{0}}\\{−\mathrm{1}\:\:\:\mathrm{if}\:\:{x}<\mathrm{0}}\end{cases} \\ $$$$\mathrm{12sgn}\left({x}^{\mathrm{2}} −{x}−\mathrm{20}\right)+\mathrm{3}\geqslant\mathrm{0} \\ $$$$\mathrm{sgn}\left({x}^{\mathrm{2}} −{x}−\mathrm{20}\right)\geqslant−\frac{\mathrm{3}}{\mathrm{12}} \\ $$$$\mathrm{sgn}\left({x}^{\mathrm{2}} −{x}−\mathrm{20}\right)\geqslant−\mathrm{0}.\mathrm{25} \\ $$$$\: \\ $$$$\mathrm{range}: \\ $$$${x}^{\mathrm{2}} −{x}−\mathrm{20}\geqslant\mathrm{0} \\ $$$$\left({x}+\mathrm{4}\right)\left({x}−\mathrm{5}\right)\geqslant\mathrm{0} \\ $$$$−\mathrm{4}\geqslant{x}\:\:\:\:\:\:\:\:{x}\geqslant\mathrm{5} \\ $$$$−\mathrm{4}\geqslant{x}\geqslant\mathrm{5} \\ $$$$\: \\ $$$$\mathrm{for}\:\:−\mathrm{4}\leqslant{x}<\mathrm{0}\:\Rightarrow\:\:\mathrm{sign}\left({x}\right)=−\mathrm{1} \\ $$$$\therefore\forall{x}\geqslant\mathrm{0},\:\:\mathrm{sgn}\left({x}^{\mathrm{2}} −{x}−\mathrm{20}\right)\geqslant−\mathrm{0}.\mathrm{25} \\ $$
Commented by FilupS last updated on 21/Apr/17

$$\mathrm{I}\:\mathrm{have}\:\mathrm{never}\:\mathrm{done}\:\mathrm{a}\:\mathrm{question}\:\mathrm{like}\:\mathrm{this}. \\ $$$$\mathrm{I}\:\mathrm{hope}\:\mathrm{I}\:\mathrm{answered}\:\mathrm{this}\:\mathrm{correcty} \\ $$
