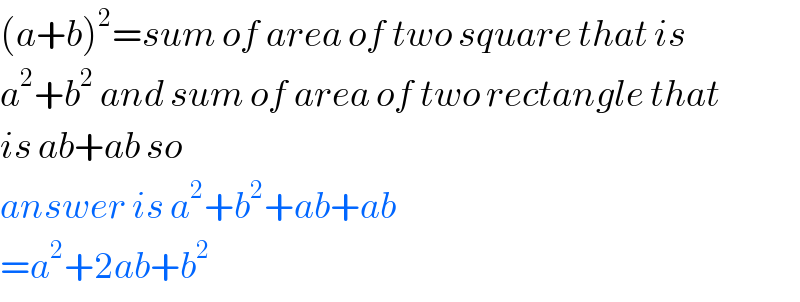2-2-2-2-

Question Number 72595 by zillnehu@gmail.com last updated on 30/Oct/19

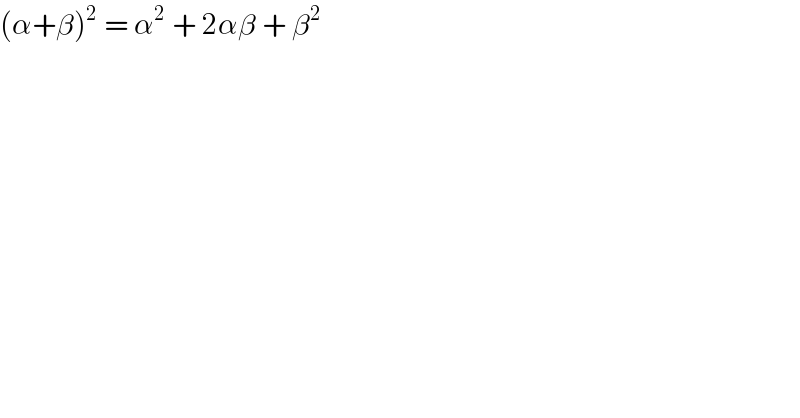
{(α + β)}^{2} = α^{2} + 2 α β + β^{2}

Commented by ajfour last updated on 30/Oct/19

a c c o r d i n g t o n e w r e s e a r c h . .

Commented by MJS last updated on 30/Oct/19

one day we might even find that 1 + 1 = 2

Commented by Learner-123 last updated on 30/Oct/19

if we replace, beta by -beta we may discover one more equation which will say 1-1=0 ��

Answered by Tanmay chaudhury last updated on 30/Oct/19

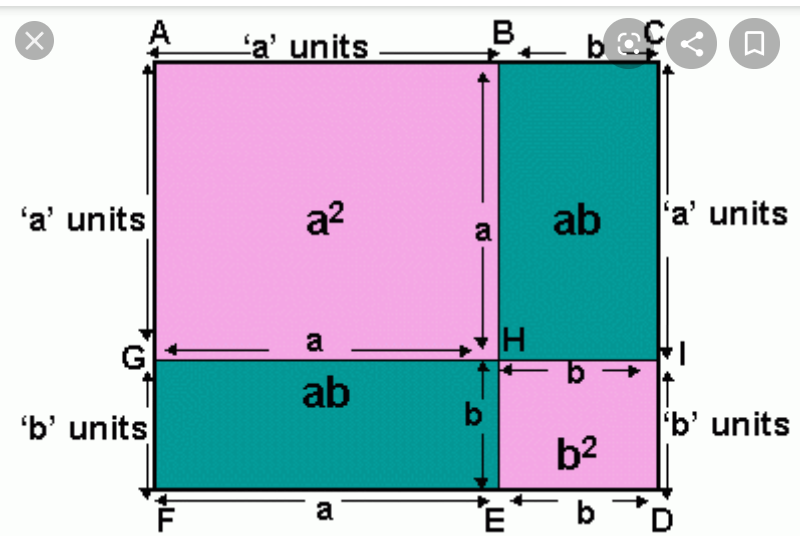
Commented by Tanmay chaudhury last updated on 30/Oct/19

{(a + b)}^{2} = s u m o f a r e a o f t w o s q u a r e t h a t i s

a^{2} + b^{2} a n d s u m o f a r e a o f t w o r e c t a n g l e t h a t

i s a b + a b s o

a n s w e r i s a^{2} + b^{2} + a b + a b

= a^{2} + 2 a b + b^{2}