Question Number 139427 by Fikret last updated on 26/Apr/21
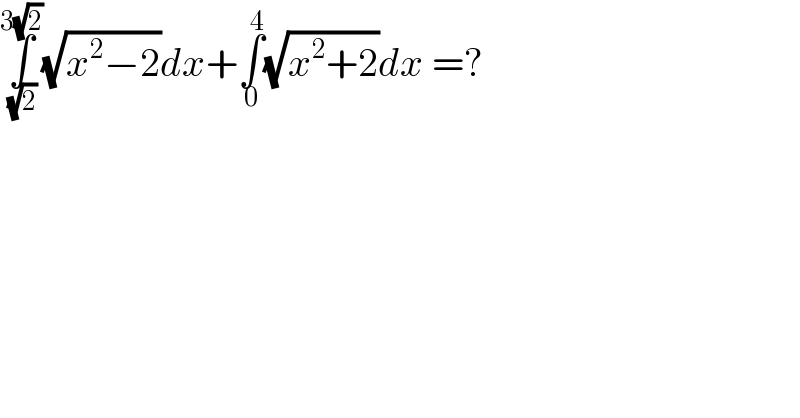
$$\underset{\:\sqrt{\mathrm{2}}} {\overset{\mathrm{3}\sqrt{\mathrm{2}}} {\int}}\sqrt{{x}^{\mathrm{2}} −\mathrm{2}}{dx}+\underset{\mathrm{0}} {\overset{\mathrm{4}} {\int}}\sqrt{{x}^{\mathrm{2}} +\mathrm{2}}{dx}\:=? \\ $$
Answered by MJS_new last updated on 27/Apr/21
![∫(√(x^2 −2))dx= [t=((x+(√(x^2 −2)))/( (√2))) → dx=((√(2(x^2 −2)))/(x+(√(x^2 −2))))dt] =∫((t/2)−(1/t)+(1/(2t^3 )))dt= =((t^4 −1)/(4t^2 ))−ln t = =(1/2)x(√(x^2 −2))−ln (x+(√(x^2 −2))) +C_1 ∫(√(x^2 +2))dx= [u=((x+(√(x^2 +2)))/( (√2))) → dx=((√(2(x^2 +2)))/(x+(√(x^2 +2))))du] =∫((u/2)+(1/u)+(1/(2u^3 )))dt= =((u^4 −1)/(4u^2 ))+ln u = =(1/2)x(√(x^2 +2))−ln (x+(√(x^2 +2))) +C_2 ⇒ answer is 12(√2)](https://www.tinkutara.com/question/Q139430.png)
$$\int\sqrt{{x}^{\mathrm{2}} −\mathrm{2}}{dx}= \\ $$$$\:\:\:\:\:\left[{t}=\frac{{x}+\sqrt{{x}^{\mathrm{2}} −\mathrm{2}}}{\:\sqrt{\mathrm{2}}}\:\rightarrow\:{dx}=\frac{\sqrt{\mathrm{2}\left({x}^{\mathrm{2}} −\mathrm{2}\right)}}{{x}+\sqrt{{x}^{\mathrm{2}} −\mathrm{2}}}{dt}\right] \\ $$$$=\int\left(\frac{{t}}{\mathrm{2}}−\frac{\mathrm{1}}{{t}}+\frac{\mathrm{1}}{\mathrm{2}{t}^{\mathrm{3}} }\right){dt}= \\ $$$$=\frac{{t}^{\mathrm{4}} −\mathrm{1}}{\mathrm{4}{t}^{\mathrm{2}} }−\mathrm{ln}\:{t}\:= \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}{x}\sqrt{{x}^{\mathrm{2}} −\mathrm{2}}−\mathrm{ln}\:\left({x}+\sqrt{{x}^{\mathrm{2}} −\mathrm{2}}\right)\:+{C}_{\mathrm{1}} \\ $$$$\int\sqrt{{x}^{\mathrm{2}} +\mathrm{2}}{dx}= \\ $$$$\:\:\:\:\:\left[{u}=\frac{{x}+\sqrt{{x}^{\mathrm{2}} +\mathrm{2}}}{\:\sqrt{\mathrm{2}}}\:\rightarrow\:{dx}=\frac{\sqrt{\mathrm{2}\left({x}^{\mathrm{2}} +\mathrm{2}\right)}}{{x}+\sqrt{{x}^{\mathrm{2}} +\mathrm{2}}}{du}\right] \\ $$$$=\int\left(\frac{{u}}{\mathrm{2}}+\frac{\mathrm{1}}{{u}}+\frac{\mathrm{1}}{\mathrm{2}{u}^{\mathrm{3}} }\right){dt}= \\ $$$$=\frac{{u}^{\mathrm{4}} −\mathrm{1}}{\mathrm{4}{u}^{\mathrm{2}} }+\mathrm{ln}\:{u}\:= \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}{x}\sqrt{{x}^{\mathrm{2}} +\mathrm{2}}−\mathrm{ln}\:\left({x}+\sqrt{{x}^{\mathrm{2}} +\mathrm{2}}\right)\:+{C}_{\mathrm{2}} \\ $$$$\Rightarrow\:\mathrm{answer}\:\mathrm{is}\:\mathrm{12}\sqrt{\mathrm{2}} \\ $$
Answered by mathmax by abdo last updated on 27/Apr/21
![I = H +K H =∫_(√2) ^(3(√2)) (√(x^2 −2))dx =_(x=(√2)cht→t=argch((x/( (√2))))) ∫_(argch(1)) ^(argch(3)) (√2)sht .(√2)sht dt =2 ∫_0 ^(ln(3+2(√2))) ((ch(2t)−1)/2)dt=[((sh(2t))/2)]_0 ^(ln(3+2(√2))) −ln(3+2(√2)) =(1/2)[((e^(2t) −e^(−2t) )/2)]_0 ^(ln(3+2(√2))) −ln(3+2(√2)) =(1/4){(3+2(√2))^2 −(3+2(√2))^(−2) } −ln(3+2(√2)) K =∫_0 ^4 (√(x^2 +2))dx =_(x=(√2)sh(t)→t=argsh((x/( (√2))))) ∫_0 ^(argsh((4/( (√2))))) (√2)ch(t)(√2)ch(t) =2 ∫_0 ^(ln((4/( (√2)))+3)) ((1+ch(2t))/2)dt =ln((4/( (√2)))+3)+[(1/2)sh(2t)]_0 ^(ln(3+2(√2))) =ln(3+2(√2))+(1/4){(3+2(√2))^2 −(3+2(√2))^(−2) } ⇒ I =(1/2){(3+2(√2))^2 −(3+2(√2))^(−2) }−ln(3+2(√2))](https://www.tinkutara.com/question/Q139431.png)
$$\mathrm{I}\:=\:\mathrm{H}\:+\mathrm{K} \\ $$$$\mathrm{H}\:=\int_{\sqrt{\mathrm{2}}} ^{\mathrm{3}\sqrt{\mathrm{2}}} \sqrt{\mathrm{x}^{\mathrm{2}} −\mathrm{2}}\mathrm{dx}\:=_{\mathrm{x}=\sqrt{\mathrm{2}}\mathrm{cht}\rightarrow\mathrm{t}=\mathrm{argch}\left(\frac{\mathrm{x}}{\:\sqrt{\mathrm{2}}}\right)} \:\:\int_{\mathrm{argch}\left(\mathrm{1}\right)} ^{\mathrm{argch}\left(\mathrm{3}\right)} \sqrt{\mathrm{2}}\mathrm{sht}\:.\sqrt{\mathrm{2}}\mathrm{sht}\:\mathrm{dt} \\ $$$$=\mathrm{2}\:\int_{\mathrm{0}} ^{\mathrm{ln}\left(\mathrm{3}+\mathrm{2}\sqrt{\mathrm{2}}\right)} \frac{\mathrm{ch}\left(\mathrm{2t}\right)−\mathrm{1}}{\mathrm{2}}\mathrm{dt}=\left[\frac{\mathrm{sh}\left(\mathrm{2t}\right)}{\mathrm{2}}\right]_{\mathrm{0}} ^{\mathrm{ln}\left(\mathrm{3}+\mathrm{2}\sqrt{\mathrm{2}}\right)} \:−\mathrm{ln}\left(\mathrm{3}+\mathrm{2}\sqrt{\mathrm{2}}\right) \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\left[\frac{\mathrm{e}^{\mathrm{2t}} −\mathrm{e}^{−\mathrm{2t}} }{\mathrm{2}}\right]_{\mathrm{0}} ^{\mathrm{ln}\left(\mathrm{3}+\mathrm{2}\sqrt{\mathrm{2}}\right)} −\mathrm{ln}\left(\mathrm{3}+\mathrm{2}\sqrt{\mathrm{2}}\right)\:=\frac{\mathrm{1}}{\mathrm{4}}\left\{\left(\mathrm{3}+\mathrm{2}\sqrt{\mathrm{2}}\right)^{\mathrm{2}} −\left(\mathrm{3}+\mathrm{2}\sqrt{\mathrm{2}}\right)^{−\mathrm{2}} \right\} \\ $$$$−\mathrm{ln}\left(\mathrm{3}+\mathrm{2}\sqrt{\mathrm{2}}\right) \\ $$$$\mathrm{K}\:=\int_{\mathrm{0}} ^{\mathrm{4}} \:\sqrt{\mathrm{x}^{\mathrm{2}} +\mathrm{2}}\mathrm{dx}\:=_{\mathrm{x}=\sqrt{\mathrm{2}}\mathrm{sh}\left(\mathrm{t}\right)\rightarrow\mathrm{t}=\mathrm{argsh}\left(\frac{\mathrm{x}}{\:\sqrt{\mathrm{2}}}\right)} \:\:\int_{\mathrm{0}} ^{\mathrm{argsh}\left(\frac{\mathrm{4}}{\:\sqrt{\mathrm{2}}}\right)} \sqrt{\mathrm{2}}\mathrm{ch}\left(\mathrm{t}\right)\sqrt{\mathrm{2}}\mathrm{ch}\left(\mathrm{t}\right) \\ $$$$=\mathrm{2}\:\int_{\mathrm{0}} ^{\mathrm{ln}\left(\frac{\mathrm{4}}{\:\sqrt{\mathrm{2}}}+\mathrm{3}\right)} \:\frac{\mathrm{1}+\mathrm{ch}\left(\mathrm{2t}\right)}{\mathrm{2}}\mathrm{dt}\:=\mathrm{ln}\left(\frac{\mathrm{4}}{\:\sqrt{\mathrm{2}}}+\mathrm{3}\right)+\left[\frac{\mathrm{1}}{\mathrm{2}}\mathrm{sh}\left(\mathrm{2t}\right)\right]_{\mathrm{0}} ^{\mathrm{ln}\left(\mathrm{3}+\mathrm{2}\sqrt{\mathrm{2}}\right)} \\ $$$$=\mathrm{ln}\left(\mathrm{3}+\mathrm{2}\sqrt{\mathrm{2}}\right)+\frac{\mathrm{1}}{\mathrm{4}}\left\{\left(\mathrm{3}+\mathrm{2}\sqrt{\mathrm{2}}\right)^{\mathrm{2}} −\left(\mathrm{3}+\mathrm{2}\sqrt{\mathrm{2}}\right)^{−\mathrm{2}} \right\}\:\Rightarrow \\ $$$$\mathrm{I}\:=\frac{\mathrm{1}}{\mathrm{2}}\left\{\left(\mathrm{3}+\mathrm{2}\sqrt{\mathrm{2}}\right)^{\mathrm{2}} −\left(\mathrm{3}+\mathrm{2}\sqrt{\mathrm{2}}\right)^{−\mathrm{2}} \right\}−\mathrm{ln}\left(\mathrm{3}+\mathrm{2}\sqrt{\mathrm{2}}\right) \\ $$
