Question Number 7030 by Tawakalitu. last updated on 07/Aug/16
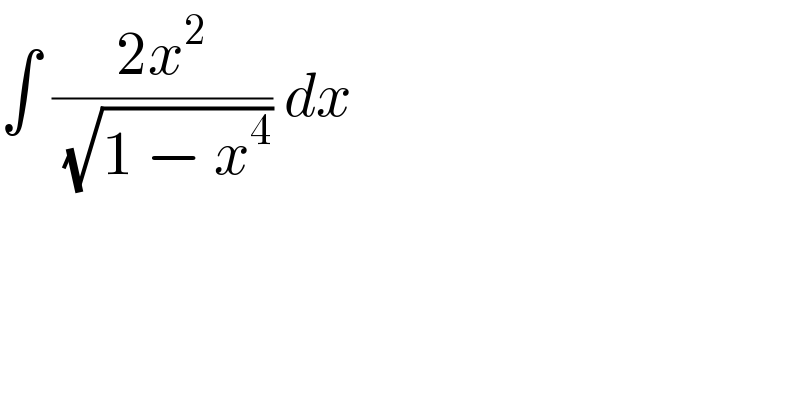
$$\int\:\frac{\mathrm{2}{x}^{\mathrm{2}} }{\:\sqrt{\mathrm{1}\:−\:{x}^{\mathrm{4}} }}\:{dx}\: \\ $$
Answered by uchechukwu okorie favour last updated on 10/Aug/16
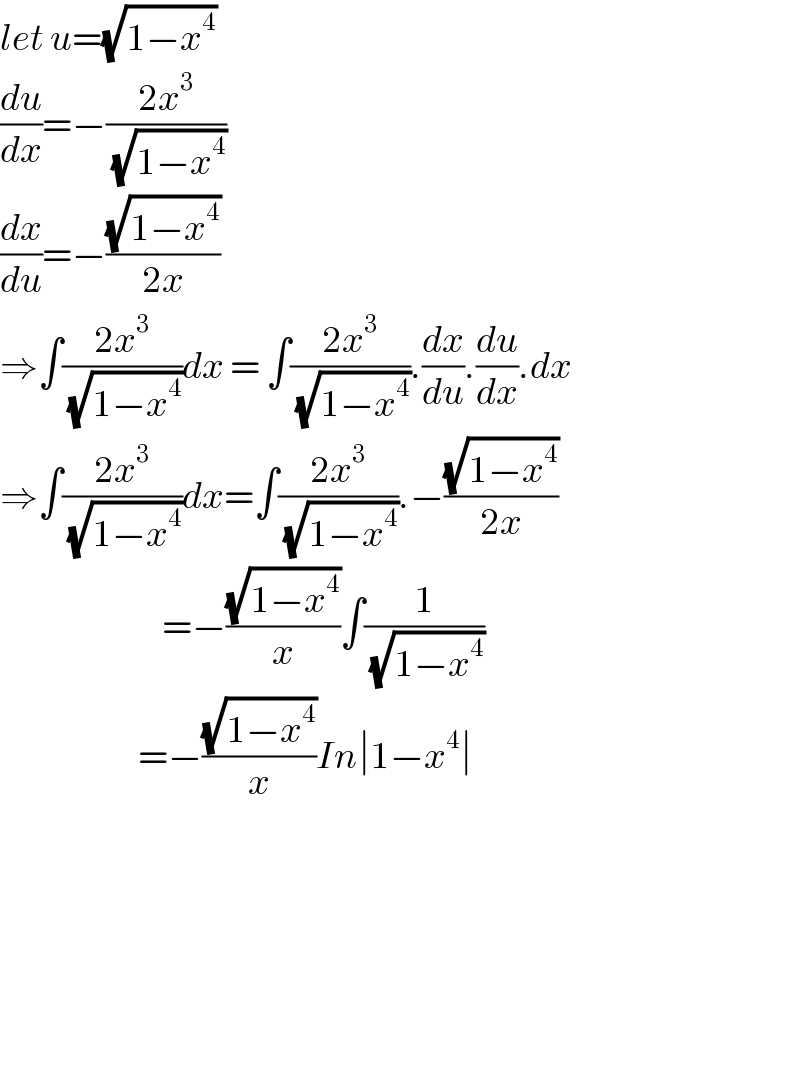
$${let}\:{u}=\sqrt{\mathrm{1}−{x}^{\mathrm{4}} } \\ $$$$\frac{{du}}{{dx}}=−\frac{\mathrm{2}{x}^{\mathrm{3}} }{\:\sqrt{\mathrm{1}−{x}^{\mathrm{4}} }} \\ $$$$\frac{{dx}}{{du}}=−\frac{\sqrt{\mathrm{1}−{x}^{\mathrm{4}} }}{\mathrm{2}{x}} \\ $$$$\Rightarrow\int\frac{\mathrm{2}{x}^{\mathrm{3}} }{\:\sqrt{\mathrm{1}−{x}^{\mathrm{4}} }}{dx}\:=\:\int\frac{\mathrm{2}{x}^{\mathrm{3}} }{\:\sqrt{\mathrm{1}−{x}^{\mathrm{4}} }}.\frac{{dx}}{{du}}.\frac{{du}}{{dx}}.{dx} \\ $$$$\Rightarrow\int\frac{\mathrm{2}{x}^{\mathrm{3}} }{\:\sqrt{\mathrm{1}−{x}^{\mathrm{4}} }}{dx}=\int\frac{\mathrm{2}{x}^{\mathrm{3}} }{\:\sqrt{\mathrm{1}−{x}^{\mathrm{4}} }}.−\frac{\sqrt{\mathrm{1}−{x}^{\mathrm{4}} }}{\mathrm{2}{x}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=−\frac{\sqrt{\mathrm{1}−{x}^{\mathrm{4}} }}{{x}}\int\frac{\mathrm{1}}{\:\sqrt{\mathrm{1}−{x}^{\mathrm{4}} }} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=−\frac{\sqrt{\mathrm{1}−{x}^{\mathrm{4}} }}{{x}}{In}\mid\mathrm{1}−{x}^{\mathrm{4}} \mid \\ $$$$ \\ $$$$ \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\: \\ $$$$ \\ $$$$ \\ $$
