Question Number 10213 by konen last updated on 30/Jan/17
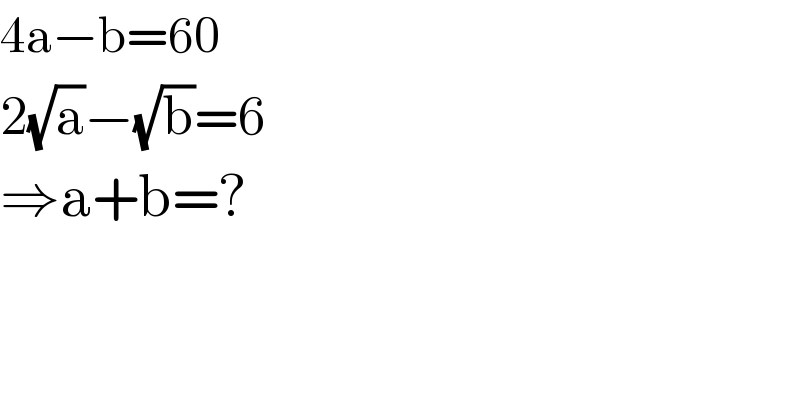
$$\mathrm{4a}−\mathrm{b}=\mathrm{60} \\ $$$$\mathrm{2}\sqrt{\mathrm{a}}−\sqrt{\mathrm{b}}=\mathrm{6} \\ $$$$\Rightarrow\mathrm{a}+\mathrm{b}=? \\ $$
Answered by nume1114 last updated on 30/Jan/17

$$\:\:\:\:\:\left(\mathrm{2}\sqrt{{a}}−\sqrt{{b}}\right)\left(\mathrm{2}\sqrt{{a}}+\sqrt{{b}}\right)=\mathrm{4}{a}−{b} \\ $$$$\Rightarrow\mathrm{6}\left(\mathrm{2}\sqrt{{a}}+\sqrt{{b}}\right)=\mathrm{60} \\ $$$$\Rightarrow\mathrm{2}\sqrt{{a}}+\sqrt{{b}}=\mathrm{10} \\ $$$$\mathrm{4}\sqrt{{a}}=\left(\mathrm{2}\sqrt{{a}}+\sqrt{{b}}\right)+\left(\mathrm{2}\sqrt{{a}}−\sqrt{{b}}\right) \\ $$$$\:\:\:\:\:\:\:=\mathrm{10}+\mathrm{6}=\mathrm{16} \\ $$$$\Rightarrow{a}=\mathrm{16} \\ $$$$\mathrm{2}\sqrt{{b}}=\left(\mathrm{2}\sqrt{{a}}+\sqrt{{b}}\right)−\left(\mathrm{2}\sqrt{{a}}−\sqrt{{b}}\right) \\ $$$$\:\:\:\:\:\:\:=\mathrm{10}−\mathrm{6}=\mathrm{4} \\ $$$$\Rightarrow{b}=\mathrm{4} \\ $$$$\Rightarrow{a}+{b}=\mathrm{16}+\mathrm{4}=\mathrm{20} \\ $$
Answered by arge last updated on 03/Feb/17
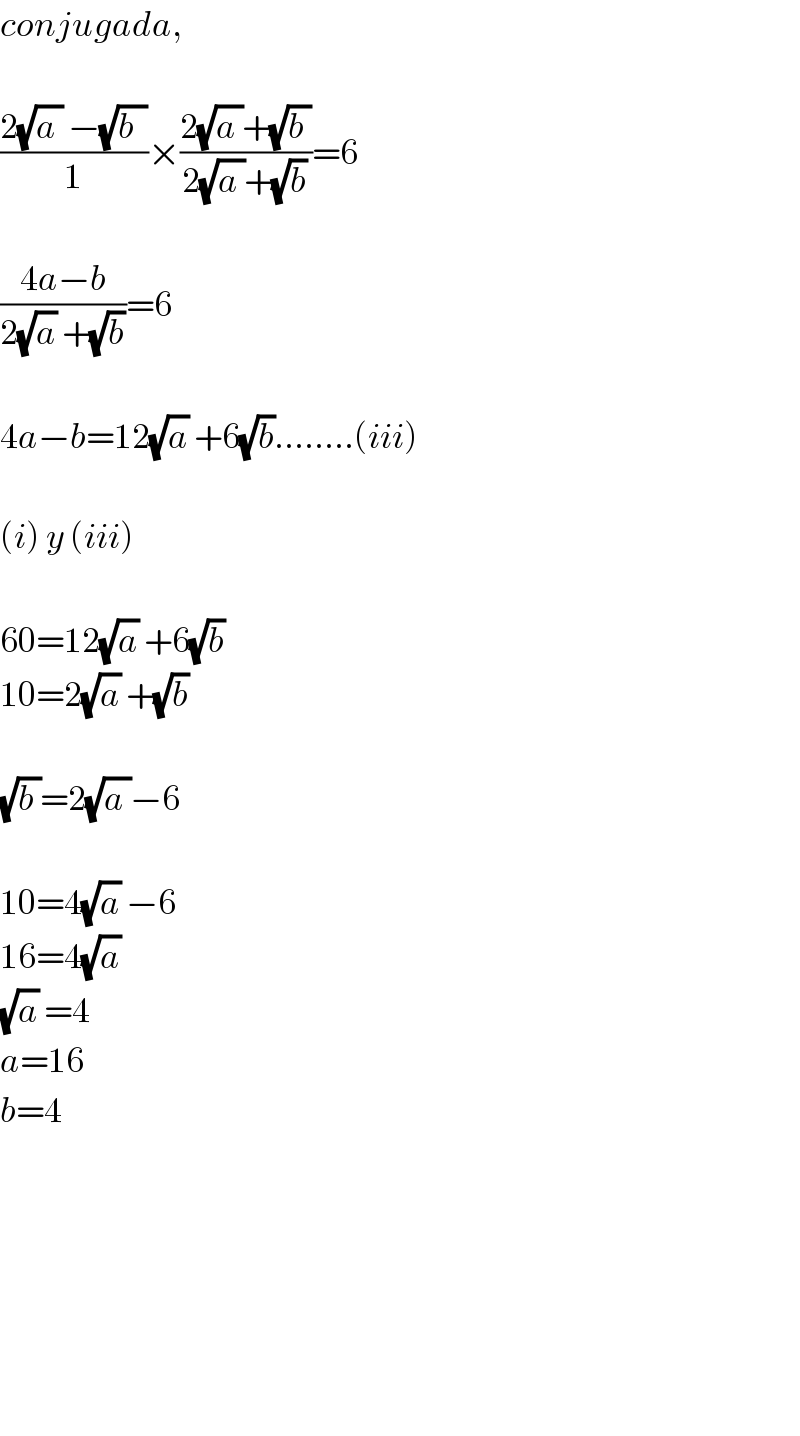
$${conjugada}, \\ $$$$ \\ $$$$\frac{\mathrm{2}\sqrt{{a}\:}\:−\sqrt{{b}\:\:}}{\mathrm{1}}×\frac{\mathrm{2}\sqrt{{a}\:}+\sqrt{{b}\:}}{\mathrm{2}\sqrt{{a}\:}+\sqrt{{b}}}=\mathrm{6} \\ $$$$ \\ $$$$\frac{\mathrm{4}{a}−{b}}{\mathrm{2}\sqrt{{a}}\:+\sqrt{{b}}}=\mathrm{6} \\ $$$$ \\ $$$$\mathrm{4}{a}−{b}=\mathrm{12}\sqrt{{a}}\:+\mathrm{6}\sqrt{{b}}……..\left({iii}\right) \\ $$$$ \\ $$$$\left({i}\right)\:{y}\:\left({iii}\right) \\ $$$$ \\ $$$$\mathrm{60}=\mathrm{12}\sqrt{{a}}\:+\mathrm{6}\sqrt{{b}} \\ $$$$\mathrm{10}=\mathrm{2}\sqrt{{a}}\:+\sqrt{{b}} \\ $$$$ \\ $$$$\sqrt{{b}\:}=\mathrm{2}\sqrt{{a}\:}−\mathrm{6} \\ $$$$ \\ $$$$\mathrm{10}=\mathrm{4}\sqrt{{a}}\:−\mathrm{6} \\ $$$$\mathrm{16}=\mathrm{4}\sqrt{{a}} \\ $$$$\sqrt{{a}}\:=\mathrm{4} \\ $$$${a}=\mathrm{16} \\ $$$${b}=\mathrm{4} \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$
