Question Number 75186 by Hassen_Timol last updated on 08/Dec/19

$$\mathrm{7}{n}\:\equiv\:\mathrm{1}\:\left(\mathrm{mod}\:\mathrm{5}\right) \\ $$$$\mathrm{What}\:\mathrm{is}\:\mathrm{the}\:\mathrm{general}\:\mathrm{form}\:\mathrm{of}\:{n}\:? \\ $$
Commented by mr W last updated on 08/Dec/19

$$\mathrm{7}{n}=\mathrm{5}{m}+\mathrm{1} \\ $$$$\Rightarrow{n}=\mathrm{5}{k}+\mathrm{3} \\ $$
Commented by Hassen_Timol last updated on 08/Dec/19
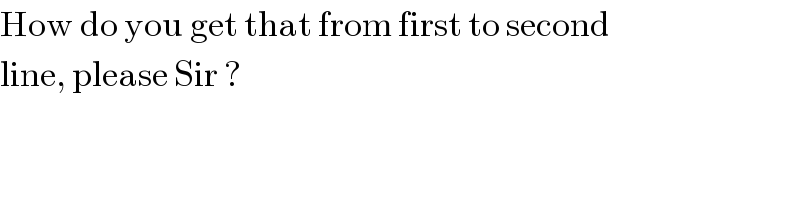
$$\mathrm{How}\:\mathrm{do}\:\mathrm{you}\:\mathrm{get}\:\mathrm{that}\:\mathrm{from}\:\mathrm{first}\:\mathrm{to}\:\mathrm{second} \\ $$$$\mathrm{line},\:\mathrm{please}\:\mathrm{Sir}\:? \\ $$
Commented by mr W last updated on 08/Dec/19

$${see}\:{my}\:{comment}\:{inQ}\mathrm{19198}\:{or}\:{here}: \\ $$
Commented by mr W last updated on 08/Dec/19

Commented by Hassen_Timol last updated on 08/Dec/19

$$\mathrm{Thank}\:\mathrm{you}\:\mathrm{sir},\:\mathrm{may}\:\mathrm{god}\:\mathrm{bless}\:\mathrm{you} \\ $$
Commented by mr W last updated on 08/Dec/19

$${see}\:{also}\:{Q}\mathrm{66832}\:{for}\:{other}\:{methods} \\ $$
Commented by jagannath.02 last updated on 08/Dec/19
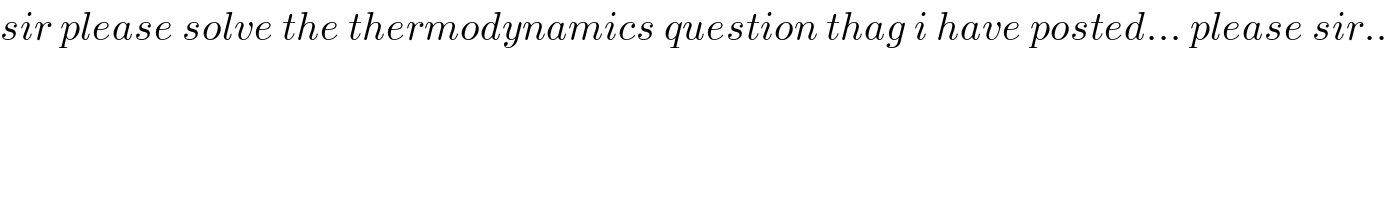
$${sir}\:{please}\:{solve}\:{the}\:{thermodynamics}\:{question}\:{thag}\:{i}\:{have}\:{posted}…\:{please}\:{sir}.. \\ $$
Answered by Rio Michael last updated on 08/Dec/19

$$\mathrm{7}{n}\:\equiv\:\mathrm{1}\left({mod}\:\mathrm{5}\right) \\ $$$${by}\:{trial}\:{and}\:{error}\:{we}\:{look}\:{for}\: \\ $$$${a}\:{number}\:{n}\:{which}\:{when}\:{multiplied}\:{to}\: \\ $$$$\mathrm{7}\:{gives}\:{a}\:{remainder}\:{of}\:\mathrm{1}\:{when}\:{divided}\:{by}\:\mathrm{5}. \\ $$$$\:{so}\: \\ $$$${n}=\mathrm{1}\:{does}\:{not}\:{work} \\ $$$${n}\:=\:\mathrm{2}\:{does}\:{not}\:{work} \\ $$$${n}\:=\:\mathrm{3}\:\Rightarrow\:\mathrm{7}\left(\mathrm{3}\right)\:=\:\mathrm{21}\: \\ $$$$\:\mathrm{21}\:=\:\mathrm{4}\left(\mathrm{5}\right)\:+\:\mathrm{1}\:{remainder}\:{of}\:\mathrm{1}\:{hence}\:{it}\:{works} \\ $$$$\therefore\:\:{n}\:=\:\mathrm{3}\:{and}\:{we}\:{write}\:\:{n}\:\equiv\:\mathrm{3}\left({mod}\:\mathrm{5}\right) \\ $$$${hence}\:{n}\:=\:\mathrm{5}{k}\:+\:\mathrm{3},\:\:{k}\in\mathbb{Z}\: \\ $$
Commented by Hassen_Timol last updated on 09/Dec/19

$$\mathrm{Thank}\:\mathrm{you}\:\mathrm{Sir} \\ $$
