A-regular-hexagon-has-sides-of-lenght-8-cm-Find-the-perpendicular-distance-between-two-opposite-faces-

Question Number 9603 by tawakalitu last updated on 20/Dec/16

A regular hexagon has sides of lenght 8 cm .

Find the perpendicular distance between two

opposite faces .

Answered by mrW last updated on 20/Dec/16

2 \times \frac{8}{2} \times \sqrt{3} = 8 \sqrt{3} cm

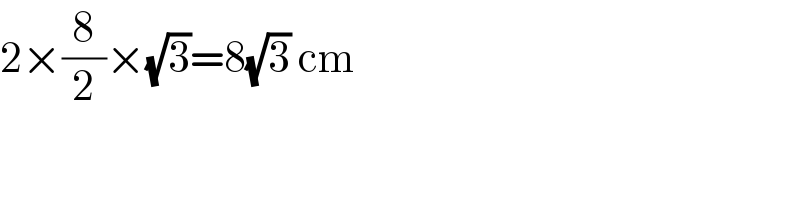
Commented by tawakalitu last updated on 20/Dec/16

God bless you sir .

Answered by sandy_suhendra last updated on 20/Dec/16

Commented by sandy_suhendra last updated on 20/Dec/16

OC = \sqrt{8^{2} - 4^{2}} = \sqrt{48} = 4 \sqrt{3}

distance between two opposite faces = 2 OC = 8 \sqrt{3}

Commented by tawakalitu last updated on 20/Dec/16

God bless you sir .