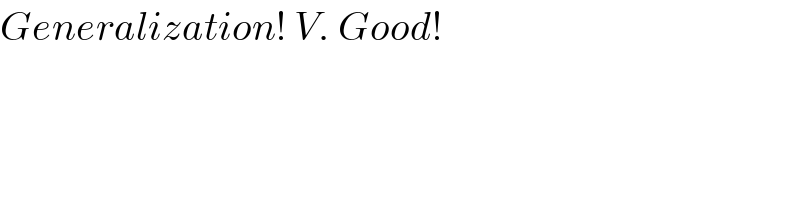Are-A-B-A-B-and-A-B-completely-equivalent-Simplify-A-B-A-B-to-A-B-using-set-operations-and-their-properties-

Question Number 1698 by Rasheed Ahmad last updated on 01/Sep/15

∙ A r e A \cup B = A \cap B a n d A = B

c o m p l e t e l y e q u i v a l e n t ?

∙ S i m p l i f y A \cup B = A \cap B t o A = B

u s i n g s e t o p e r a t i o n s a n d t h e i r

p r o p e r t i e s .

Answered by 123456 last updated on 01/Sep/15

if A \cup B = A \cap B them A = B

we have that A \cup B = A \cap B them \forall x, x \in A \cup B, x \in A \cap B

them supose that A \neq B, without loss

of generality suppose tbat ∣ A ∣>∣ B ∣

x \in A, x \notin B

x \in A \cup B, x \notin A \cap B (contradition)

hence A = B

and if A = B, the

A \cup B = A \cup A = A

A \cap B = A \cap A = A

A \cup B = A \cap B

so

A \cup B = A \cap B \Leftrightarrow A = B

Answered by 123456 last updated on 01/Sep/15

A \cup B = A \cap B

(A \cup B) \cup A = (A \cap B) \cup A

A \cup B = A

(A \cup B) \cup B = (A \cap B) \cup B

A \cup B = B

A = B

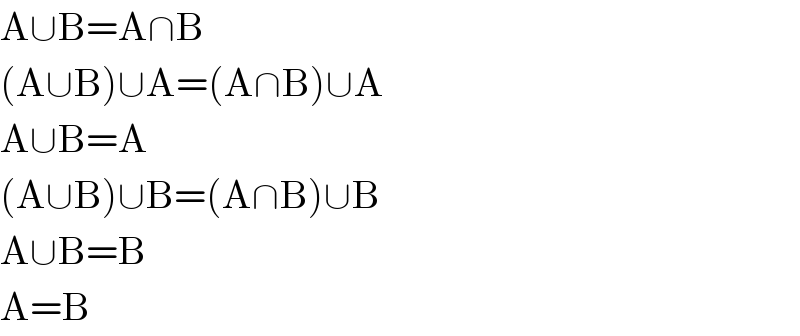
Commented by 123456 last updated on 01/Sep/15

(A \cup B) \cap A = (A \cap B) \cap A

A = A \cap B

(A \cup B) \cap B = (A \cap B) \cap B

B = A \cap B

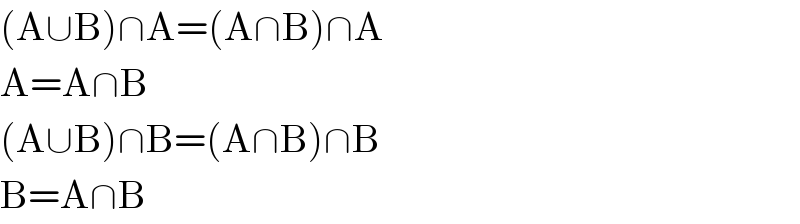
Commented by Rasheed Ahmad last updated on 01/Sep/15

G^{o o} {DD}_{eductio} N_{!}^{!}

Commented by 123456 last updated on 02/Sep/15

also we can extend it to

\underset{i = 1}{\overset{n}{\cup}} A_{i} = \underset{i = 1}{\overset{n}{\cap}} A_{i} \Leftrightarrow A_{i} = A_{j}, i \in {1, \dots, n}, j \in {1, \dots, n}

n \in N^{*}

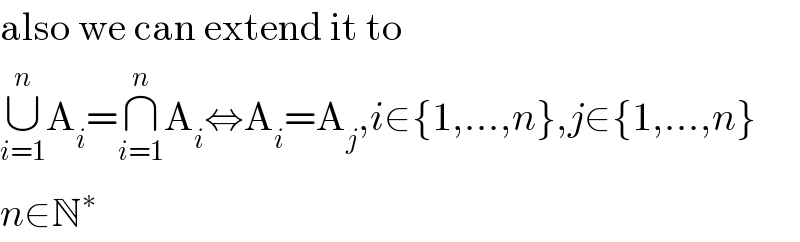
Commented by Rasheed Soomro last updated on 03/Sep/15

G e n e r a l i z a t i o n! V . G o o d!