Menu Close

calculate-0-arctan-2cosx-3-x-2-dx-

Tinku Tara 0 Comments
Pin




Question Number 73338 by mathmax by abdo last updated on 10/Nov/19
calculate ∫_0 ^∞ ((arctan(2cosx))/(3+x^2 ))dx
$${calculate}\:\int_{\mathrm{0}} ^{\infty} \:\:\frac{{arctan}\left(\mathrm{2}{cosx}\right)}{\mathrm{3}+{x}^{\mathrm{2}} }{dx} \\ $$
Commented by mathmax by abdo last updated on 11/Nov/19
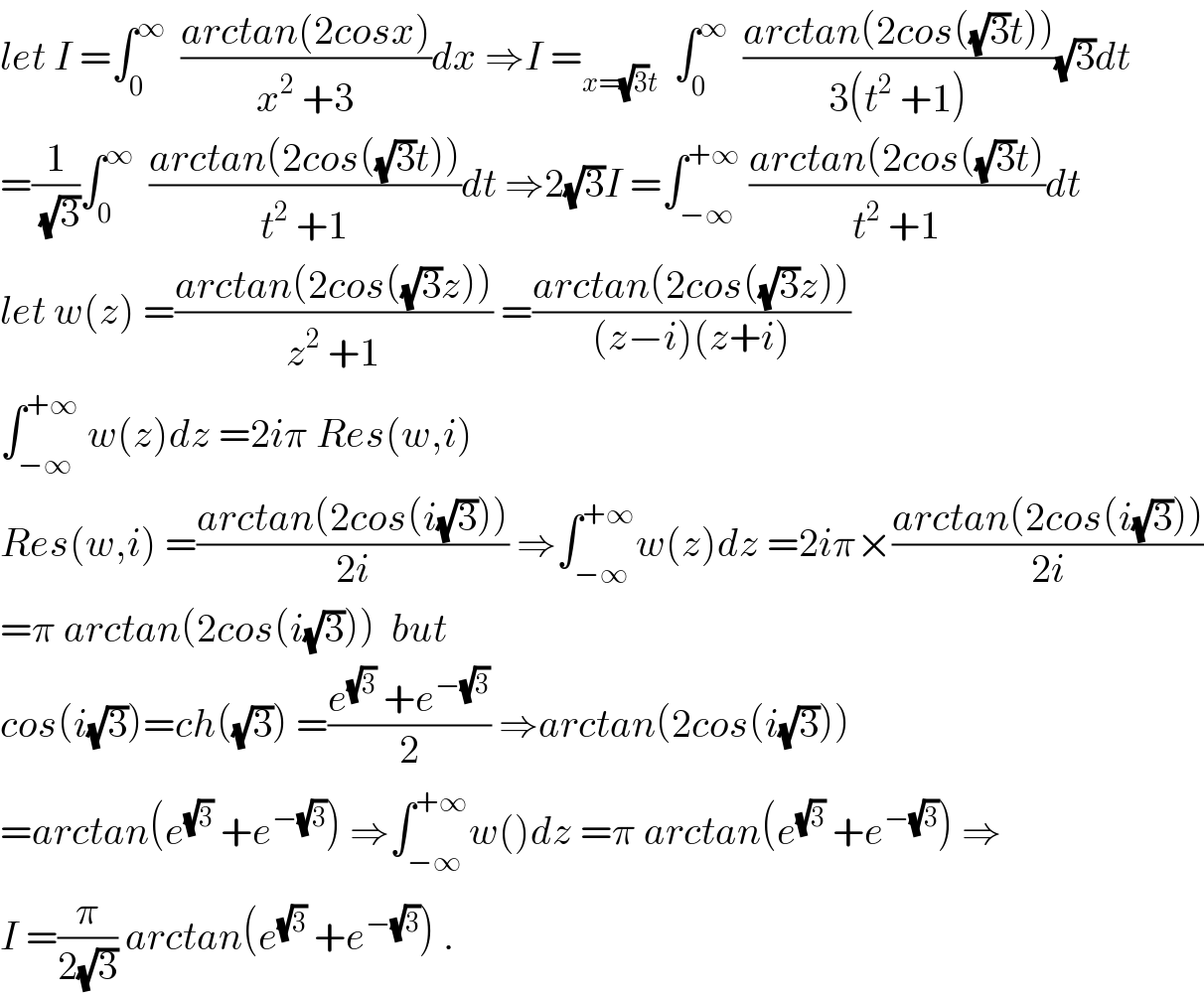
let I =∫_0 ^∞ ((arctan(2cosx))/(x^2 +3))dx ⇒I =_(x=(√3)t) ∫_0 ^∞ ((arctan(2cos((√3)t)))/(3(t^2 +1)))(√3)dt =(1/( (√3)))∫_0 ^∞ ((arctan(2cos((√3)t)))/(t^2 +1))dt ⇒2(√3)I =∫_(−∞) ^(+∞) ((arctan(2cos((√3)t))/(t^2 +1))dt let w(z) =((arctan(2cos((√3)z)))/(z^2 +1)) =((arctan(2cos((√3)z)))/((z−i)(z+i))) ∫_(−∞) ^(+∞) w(z)dz =2iπ Res(w,i) Res(w,i) =((arctan(2cos(i(√3))))/(2i)) ⇒∫_(−∞) ^(+∞) w(z)dz =2iπ×((arctan(2cos(i(√3))))/(2i)) =π arctan(2cos(i(√3))) but cos(i(√3))=ch((√3)) =((e^(√3) +e^(−(√3)) )/2) ⇒arctan(2cos(i(√3))) =arctan(e^(√3) +e^(−(√3)) ) ⇒∫_(−∞) ^(+∞) w()dz =π arctan(e^(√3) +e^(−(√3)) ) ⇒ I =(π/(2(√3))) arctan(e^(√3) +e^(−(√3)) ) .
$${let}\:{I}\:=\int_{\mathrm{0}} ^{\infty} \:\:\frac{{arctan}\left(\mathrm{2}{cosx}\right)}{{x}^{\mathrm{2}} \:+\mathrm{3}}{dx}\:\Rightarrow{I}\:=_{{x}=\sqrt{\mathrm{3}}{t}} \:\:\int_{\mathrm{0}} ^{\infty} \:\:\frac{{arctan}\left(\mathrm{2}{cos}\left(\sqrt{\mathrm{3}}{t}\right)\right)}{\mathrm{3}\left({t}^{\mathrm{2}} \:+\mathrm{1}\right)}\sqrt{\mathrm{3}}{dt} \\ $$$$=\frac{\mathrm{1}}{\:\sqrt{\mathrm{3}}}\int_{\mathrm{0}} ^{\infty} \:\:\frac{{arctan}\left(\mathrm{2}{cos}\left(\sqrt{\mathrm{3}}{t}\right)\right)}{{t}^{\mathrm{2}} \:+\mathrm{1}}{dt}\:\Rightarrow\mathrm{2}\sqrt{\mathrm{3}}{I}\:=\int_{−\infty} ^{+\infty} \:\frac{{arctan}\left(\mathrm{2}{cos}\left(\sqrt{\mathrm{3}}{t}\right)\right.}{{t}^{\mathrm{2}} \:+\mathrm{1}}{dt} \\ $$$${let}\:{w}\left({z}\right)\:=\frac{{arctan}\left(\mathrm{2}{cos}\left(\sqrt{\mathrm{3}}{z}\right)\right)}{{z}^{\mathrm{2}} \:+\mathrm{1}}\:=\frac{{arctan}\left(\mathrm{2}{cos}\left(\sqrt{\mathrm{3}}{z}\right)\right)}{\left({z}−{i}\right)\left({z}+{i}\right)} \\ $$$$\int_{−\infty} ^{+\infty} \:{w}\left({z}\right){dz}\:=\mathrm{2}{i}\pi\:{Res}\left({w},{i}\right) \\ $$$${Res}\left({w},{i}\right)\:=\frac{{arctan}\left(\mathrm{2}{cos}\left({i}\sqrt{\mathrm{3}}\right)\right)}{\mathrm{2}{i}}\:\Rightarrow\int_{−\infty} ^{+\infty} {w}\left({z}\right){dz}\:=\mathrm{2}{i}\pi×\frac{{arctan}\left(\mathrm{2}{cos}\left({i}\sqrt{\mathrm{3}}\right)\right)}{\mathrm{2}{i}} \\ $$$$=\pi\:{arctan}\left(\mathrm{2}{cos}\left({i}\sqrt{\mathrm{3}}\right)\right)\:\:{but} \\ $$$${cos}\left({i}\sqrt{\mathrm{3}}\right)={ch}\left(\sqrt{\mathrm{3}}\right)\:=\frac{{e}^{\sqrt{\mathrm{3}}} \:+{e}^{−\sqrt{\mathrm{3}}} }{\mathrm{2}}\:\Rightarrow{arctan}\left(\mathrm{2}{cos}\left({i}\sqrt{\mathrm{3}}\right)\right) \\ $$$$={arctan}\left({e}^{\sqrt{\mathrm{3}}} \:+{e}^{−\sqrt{\mathrm{3}}} \right)\:\Rightarrow\int_{−\infty} ^{+\infty} {w}\left(\right){dz}\:=\pi\:{arctan}\left({e}^{\sqrt{\mathrm{3}}} \:+{e}^{−\sqrt{\mathrm{3}}} \right)\:\Rightarrow \\ $$$${I}\:=\frac{\pi}{\mathrm{2}\sqrt{\mathrm{3}}}\:{arctan}\left({e}^{\sqrt{\mathrm{3}}} \:+{e}^{−\sqrt{\mathrm{3}}} \right)\:. \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *