Question Number 66695 by mathmax by abdo last updated on 18/Aug/19
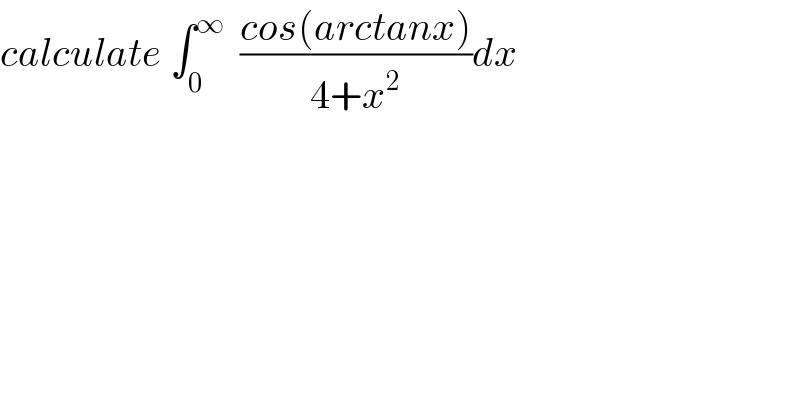
$${calculate}\:\int_{\mathrm{0}} ^{\infty} \:\:\frac{{cos}\left({arctanx}\right)}{\mathrm{4}+{x}^{\mathrm{2}} }{dx} \\ $$
Commented by ~ À ® @ 237 ~ last updated on 18/Aug/19
![Let named it J As 1+tan^2 t=(1/(cos^2 t)) we have cos(arctanx)=(1/( (√(1+x^2 )) )) Now J=∫_0 ^∞ (dx/((4+x^2 )(√(1+x^2 )))) let state x=shu ⇒ du=(dx/( (√(1+x^2 )) )) J= ∫_0 ^∞ (( du)/(4+sh^2 u)) = ∫_0 ^∞ ((1/(ch^2 u))/((4/(ch^2 u)) + th^2 u)) du As (1/(ch^2 u)) =1−th^2 u and (d/du) (thu)=(1/(ch^2 u)) we have J= ∫_0 ^∞ ((d(thu))/(4 −3th^2 u)) = (1/4). ∫_0 ^∞ ((d(thu))/(1−((((√3) )/2) thu)^2 ))=(1/(2(√3))) .∫_0 ^∞ (( d(((√3)/2) thu))/(1−(((√3)/2) thu)^2 )) =(1/(2(√3))) [argth(((√3)/2) thu)]_0 ^∞ = (1/(2(√3))) argth(((√3)/2)) finally J= (1/(2(√3))) . (1/2)ln(((1+((√3)/2))/(1−((√3)/2))))=(1/(4(√3) )). ln(((4+2(√3))/(4−2(√3))) )=((ln(1+(√3)))/( (√3))) −((ln2)/(2(√3)))](https://www.tinkutara.com/question/Q66713.png)
$$\:{Let}\:{named}\:{it}\:{J}\: \\ $$$${As}\:\mathrm{1}+{tan}^{\mathrm{2}} {t}=\frac{\mathrm{1}}{{cos}^{\mathrm{2}} {t}}\:\:{we}\:{have}\:\:{cos}\left({arctanx}\right)=\frac{\mathrm{1}}{\:\sqrt{\mathrm{1}+{x}^{\mathrm{2}} }\:} \\ $$$${Now}\:\:{J}=\int_{\mathrm{0}} ^{\infty} \:\:\frac{{dx}}{\left(\mathrm{4}+{x}^{\mathrm{2}} \right)\sqrt{\mathrm{1}+{x}^{\mathrm{2}} }}\: \\ $$$${let}\:{state}\:{x}={shu}\:\Rightarrow\:{du}=\frac{{dx}}{\:\sqrt{\mathrm{1}+{x}^{\mathrm{2}} }\:} \\ $$$${J}=\:\int_{\mathrm{0}} ^{\infty} \frac{\:{du}}{\mathrm{4}+{sh}^{\mathrm{2}} {u}}\:=\:\int_{\mathrm{0}} ^{\infty} \:\frac{\frac{\mathrm{1}}{{ch}^{\mathrm{2}} {u}}}{\frac{\mathrm{4}}{{ch}^{\mathrm{2}} {u}}\:+\:{th}^{\mathrm{2}} {u}}\:{du}\: \\ $$$${As}\:\:\:\frac{\mathrm{1}}{{ch}^{\mathrm{2}} {u}}\:=\mathrm{1}−{th}^{\mathrm{2}} {u}\:\:{and}\:\:\frac{{d}}{{du}}\:\left({thu}\right)=\frac{\mathrm{1}}{{ch}^{\mathrm{2}} {u}}\:\:\:\:\:{we}\:{have} \\ $$$${J}=\:\int_{\mathrm{0}} ^{\infty} \:\frac{{d}\left({thu}\right)}{\mathrm{4}\:−\mathrm{3}{th}^{\mathrm{2}} {u}}\:=\:\frac{\mathrm{1}}{\mathrm{4}}.\:\int_{\mathrm{0}} ^{\infty} \:\frac{{d}\left({thu}\right)}{\mathrm{1}−\left(\frac{\sqrt{\mathrm{3}}\:}{\mathrm{2}}\:{thu}\right)^{\mathrm{2}} }=\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{3}}}\:.\int_{\mathrm{0}} ^{\infty} \:\frac{\:{d}\left(\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\:{thu}\right)}{\mathrm{1}−\left(\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\:{thu}\right)^{\mathrm{2}} }\:=\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{3}}}\:\left[{argth}\left(\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\:{thu}\right)\right]_{\mathrm{0}} ^{\infty} =\:\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{3}}}\:{argth}\left(\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\right) \\ $$$${finally} \\ $$$${J}=\:\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{3}}}\:.\:\frac{\mathrm{1}}{\mathrm{2}}{ln}\left(\frac{\mathrm{1}+\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}}{\mathrm{1}−\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}}\right)=\frac{\mathrm{1}}{\mathrm{4}\sqrt{\mathrm{3}}\:}.\:{ln}\left(\frac{\mathrm{4}+\mathrm{2}\sqrt{\mathrm{3}}}{\mathrm{4}−\mathrm{2}\sqrt{\mathrm{3}}}\:\right)=\frac{{ln}\left(\mathrm{1}+\sqrt{\mathrm{3}}\right)}{\:\sqrt{\mathrm{3}}}\:−\frac{{ln}\mathrm{2}}{\mathrm{2}\sqrt{\mathrm{3}}}\: \\ $$
Commented by mathmax by abdo last updated on 19/Aug/19

$${thanks}\:{sir}. \\ $$
Commented by mathmax by abdo last updated on 19/Aug/19
![let I=∫_0 ^∞ ((cos(arctanx))/(4+x^2 ))dx we know that cos(arctanx)=(1/( (√(1+x^2 )))) ⇒ I =∫_0 ^∞ (dx/((x^2 +4)(√(1+x^2 )))) changement x=sht give I =∫_0 ^∞ ((ch(t))/((sh^2 t +4)ch(t)))dt =∫_0 ^∞ (dt/(4+((ch(2t)−1)/2))) =∫_0 ^∞ ((2dt)/(8+ch(2t)−1)) =∫_0 ^∞ ((2dt)/(7+ch(2t))) =∫_0 ^∞ ((2dt)/(7+((e^(2t) +e^(−2t) )/2))) =∫_0 ^∞ ((4dt)/(14+e^(2t) +e^(−2t) )) =_(e^(2t) =u) ∫_1 ^(+∞) (4/(14 +u+u^(−1) )) (du/(2u)) = ∫_1 ^(+∞) ((2du)/(14u +u^2 +1)) =∫_1 ^(+∞) ((2du)/(u^2 +14u +1)) u^2 +14u+1=0→Δ^′ =7^2 −1 =48 ⇒u_1 =−7+(√(48))=−7+4(√3) u_2 =−7−4(√3) ⇒I =∫_1 ^(+∞) ((2du)/((u−u_1 )(u−u_2 ))) =(2/(8(√3)))∫_1 ^(+∞) {(1/(u−u_1 ))−(1/(u−u_2 ))}du =(1/(4(√3)))[ln∣((u−u_1 )/(u−u_2 ))∣]_1 ^(+∞) =(1/(4(√3))){−ln∣((1−u_1 )/(1−u_2 ))∣} =(1/(4(√3))){ln∣((1+7+4(√3))/(1+7−4(√3)))∣} =(1/(4(√3)))ln∣((8+4(√3))/(8−4(√3)))∣ =(1/(4(√3)))ln(((2+(√3))/(2−(√3)))) .](https://www.tinkutara.com/question/Q66721.png)
$${let}\:{I}=\int_{\mathrm{0}} ^{\infty} \:\:\frac{{cos}\left({arctanx}\right)}{\mathrm{4}+{x}^{\mathrm{2}} }{dx}\:\:{we}\:{know}\:{that}\:{cos}\left({arctanx}\right)=\frac{\mathrm{1}}{\:\sqrt{\mathrm{1}+{x}^{\mathrm{2}} }} \\ $$$$\Rightarrow\:{I}\:=\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{{dx}}{\left({x}^{\mathrm{2}} \:+\mathrm{4}\right)\sqrt{\mathrm{1}+{x}^{\mathrm{2}} }}\:\:{changement}\:\:{x}={sht}\:{give} \\ $$$${I}\:=\int_{\mathrm{0}} ^{\infty} \:\frac{{ch}\left({t}\right)}{\left({sh}^{\mathrm{2}} {t}\:+\mathrm{4}\right){ch}\left({t}\right)}{dt}\:=\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{{dt}}{\mathrm{4}+\frac{{ch}\left(\mathrm{2}{t}\right)−\mathrm{1}}{\mathrm{2}}}\:=\int_{\mathrm{0}} ^{\infty} \:\frac{\mathrm{2}{dt}}{\mathrm{8}+{ch}\left(\mathrm{2}{t}\right)−\mathrm{1}} \\ $$$$=\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{\mathrm{2}{dt}}{\mathrm{7}+{ch}\left(\mathrm{2}{t}\right)}\:=\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{\mathrm{2}{dt}}{\mathrm{7}+\frac{{e}^{\mathrm{2}{t}} \:+{e}^{−\mathrm{2}{t}} }{\mathrm{2}}}\:=\int_{\mathrm{0}} ^{\infty} \:\:\frac{\mathrm{4}{dt}}{\mathrm{14}+{e}^{\mathrm{2}{t}} \:+{e}^{−\mathrm{2}{t}} } \\ $$$$=_{{e}^{\mathrm{2}{t}} ={u}} \:\:\:\int_{\mathrm{1}} ^{+\infty} \:\:\:\:\frac{\mathrm{4}}{\mathrm{14}\:+{u}+{u}^{−\mathrm{1}} }\:\frac{{du}}{\mathrm{2}{u}}\:=\:\int_{\mathrm{1}} ^{+\infty} \:\:\frac{\mathrm{2}{du}}{\mathrm{14}{u}\:+{u}^{\mathrm{2}} \:+\mathrm{1}} \\ $$$$=\int_{\mathrm{1}} ^{+\infty} \:\frac{\mathrm{2}{du}}{{u}^{\mathrm{2}} \:+\mathrm{14}{u}\:+\mathrm{1}} \\ $$$${u}^{\mathrm{2}} \:+\mathrm{14}{u}+\mathrm{1}=\mathrm{0}\rightarrow\Delta^{'} =\mathrm{7}^{\mathrm{2}} −\mathrm{1}\:=\mathrm{48}\:\Rightarrow{u}_{\mathrm{1}} =−\mathrm{7}+\sqrt{\mathrm{48}}=−\mathrm{7}+\mathrm{4}\sqrt{\mathrm{3}} \\ $$$${u}_{\mathrm{2}} =−\mathrm{7}−\mathrm{4}\sqrt{\mathrm{3}}\:\Rightarrow{I}\:=\int_{\mathrm{1}} ^{+\infty} \:\:\frac{\mathrm{2}{du}}{\left({u}−{u}_{\mathrm{1}} \right)\left({u}−{u}_{\mathrm{2}} \right)} \\ $$$$=\frac{\mathrm{2}}{\mathrm{8}\sqrt{\mathrm{3}}}\int_{\mathrm{1}} ^{+\infty} \:\left\{\frac{\mathrm{1}}{{u}−{u}_{\mathrm{1}} }−\frac{\mathrm{1}}{{u}−{u}_{\mathrm{2}} }\right\}{du}\:=\frac{\mathrm{1}}{\mathrm{4}\sqrt{\mathrm{3}}}\left[{ln}\mid\frac{{u}−{u}_{\mathrm{1}} }{{u}−{u}_{\mathrm{2}} }\mid\right]_{\mathrm{1}} ^{+\infty} \\ $$$$=\frac{\mathrm{1}}{\mathrm{4}\sqrt{\mathrm{3}}}\left\{−{ln}\mid\frac{\mathrm{1}−{u}_{\mathrm{1}} }{\mathrm{1}−{u}_{\mathrm{2}} }\mid\right\}\:=\frac{\mathrm{1}}{\mathrm{4}\sqrt{\mathrm{3}}}\left\{{ln}\mid\frac{\mathrm{1}+\mathrm{7}+\mathrm{4}\sqrt{\mathrm{3}}}{\mathrm{1}+\mathrm{7}−\mathrm{4}\sqrt{\mathrm{3}}}\mid\right\}\:=\frac{\mathrm{1}}{\mathrm{4}\sqrt{\mathrm{3}}}{ln}\mid\frac{\mathrm{8}+\mathrm{4}\sqrt{\mathrm{3}}}{\mathrm{8}−\mathrm{4}\sqrt{\mathrm{3}}}\mid \\ $$$$=\frac{\mathrm{1}}{\mathrm{4}\sqrt{\mathrm{3}}}{ln}\left(\frac{\mathrm{2}+\sqrt{\mathrm{3}}}{\mathrm{2}−\sqrt{\mathrm{3}}}\right)\:. \\ $$
Answered by mind is power last updated on 18/Aug/19
![cos(arctg(x))=cos(x)=(1/( (√(1+tg^2 (x)))))==>(1/( (√((1+x^2 )))))=cos(arctg(x)).x≥0 =∫_0 ^(+∞) (dx/( (√((1+x^2 )))(4+x^2 ))) x=sh(t) dx=ch(t) ==>∫_0 ^(+∞) ((ch(t)dt)/(ch(t)(4+sh^2 (t)))) withe e^t =w=≥dt=(1/w)dw ==>∫_1 ^(+∞) (dw/(w(4+(w^2 /4)+(1/(4w^2 ))−1)))=∫_(−∞) ^(+∞) ((4wdw)/((w^4 +12w^2 +1)))=∫((4w)/((w^2 +6−(√(35)))(w^2 +6+(√(35))))) ((4w)/((w^2 +6−(√(35)))(w^2 +6+(√(35)))))=(2/( (√(35))))((w/(w^2 +6−(√(35))))−(w/(w^2 +6+(√(35))))) ==>∫((4wdw)/(w^4 −12w^2 +1))=(1/( (√(35))))ln(((w^2 +6−(√(35)))/(w^2 +6+(√(35)))))+c ==>∫_1 ^(+∞) ((4wdw)/(w^4 −12w^2 +1))=lim_(x→∞) [(2/( (√(35))))ln (((w^2 +6−(√(35)))/(w^2 +6+(√(35)))))]_1 ^x =lim_(x→+∞) (2/( (√(35))))ln(((x^2 +6−(√(35)))/(x^2 +6+(√(35)))))+(2/( (√(35))))ln(((7+(√(35)))/(7−(√(35)))))=(2/( (√(35))))ln(((7+(√(35)))/(7−(√(35)))))](https://www.tinkutara.com/question/Q66706.png)
$${cos}\left({arctg}\left({x}\right)\right)={cos}\left({x}\right)=\frac{\mathrm{1}}{\:\sqrt{\mathrm{1}+{tg}^{\mathrm{2}} \left({x}\right)}}==>\frac{\mathrm{1}}{\:\sqrt{\left(\mathrm{1}+{x}^{\mathrm{2}} \right)}}={cos}\left({arctg}\left({x}\right)\right).{x}\geqslant\mathrm{0} \\ $$$$=\int_{\mathrm{0}} ^{+\infty} \frac{{dx}}{\:\sqrt{\left(\mathrm{1}+{x}^{\mathrm{2}} \right)}\left(\mathrm{4}+{x}^{\mathrm{2}} \right)} \\ $$$${x}={sh}\left({t}\right) \\ $$$${dx}={ch}\left({t}\right) \\ $$$$==>\int_{\mathrm{0}} ^{+\infty} \frac{{ch}\left({t}\right){dt}}{{ch}\left({t}\right)\left(\mathrm{4}+{sh}^{\mathrm{2}} \left({t}\right)\right)} \\ $$$${withe}\:{e}^{{t}} ={w}=\geqslant{dt}=\frac{\mathrm{1}}{{w}}{dw} \\ $$$$==>\int_{\mathrm{1}} ^{+\infty} \frac{{dw}}{{w}\left(\mathrm{4}+\frac{{w}^{\mathrm{2}} }{\mathrm{4}}+\frac{\mathrm{1}}{\mathrm{4}{w}^{\mathrm{2}} }−\mathrm{1}\right)}=\int_{−\infty} ^{+\infty} \frac{\mathrm{4}{wdw}}{\left({w}^{\mathrm{4}} +\mathrm{12}{w}^{\mathrm{2}} +\mathrm{1}\right)}=\int\frac{\mathrm{4}{w}}{\left({w}^{\mathrm{2}} +\mathrm{6}−\sqrt{\mathrm{35}}\right)\left({w}^{\mathrm{2}} +\mathrm{6}+\sqrt{\mathrm{35}}\right)} \\ $$$$\frac{\mathrm{4}{w}}{\left({w}^{\mathrm{2}} +\mathrm{6}−\sqrt{\left.\mathrm{35}\right)}\left({w}^{\mathrm{2}} +\mathrm{6}+\sqrt{\mathrm{35}}\right)\right.}=\frac{\mathrm{2}}{\:\sqrt{\mathrm{35}}}\left(\frac{{w}}{{w}^{\mathrm{2}} +\mathrm{6}−\sqrt{\mathrm{35}}}−\frac{{w}}{{w}^{\mathrm{2}} +\mathrm{6}+\sqrt{\mathrm{35}}}\right) \\ $$$$==>\int\frac{\mathrm{4}{wdw}}{{w}^{\mathrm{4}} −\mathrm{12}{w}^{\mathrm{2}} +\mathrm{1}}=\frac{\mathrm{1}}{\:\sqrt{\mathrm{35}}}{ln}\left(\frac{{w}^{\mathrm{2}} +\mathrm{6}−\sqrt{\mathrm{35}}}{{w}^{\mathrm{2}} +\mathrm{6}+\sqrt{\mathrm{35}}}\right)+{c} \\ $$$$==>\int_{\mathrm{1}} ^{+\infty} \frac{\mathrm{4}{wdw}}{{w}^{\mathrm{4}} −\mathrm{12}{w}^{\mathrm{2}} +\mathrm{1}}={li}\underset{{x}\rightarrow\infty} {{m}}\left[\frac{\mathrm{2}}{\:\sqrt{\mathrm{35}}}\mathrm{ln}\:\left(\frac{{w}^{\mathrm{2}} +\mathrm{6}−\sqrt{\mathrm{35}}}{{w}^{\mathrm{2}} +\mathrm{6}+\sqrt{\mathrm{35}}}\right)\right]_{\mathrm{1}} ^{{x}} \\ $$$$={lim}_{{x}\rightarrow+\infty} \frac{\mathrm{2}}{\:\sqrt{\mathrm{35}}}{ln}\left(\frac{{x}^{\mathrm{2}} +\mathrm{6}−\sqrt{\mathrm{35}}}{{x}^{\mathrm{2}} +\mathrm{6}+\sqrt{\mathrm{35}}}\right)+\frac{\mathrm{2}}{\:\sqrt{\mathrm{35}}}{ln}\left(\frac{\mathrm{7}+\sqrt{\mathrm{35}}}{\mathrm{7}−\sqrt{\mathrm{35}}}\right)=\frac{\mathrm{2}}{\:\sqrt{\mathrm{35}}}{ln}\left(\frac{\mathrm{7}+\sqrt{\mathrm{35}}}{\mathrm{7}−\sqrt{\mathrm{35}}}\right) \\ $$$$ \\ $$$$ \\ $$
Commented by mathmax by abdo last updated on 19/Aug/19

$${thanks}\:{sir}. \\ $$
