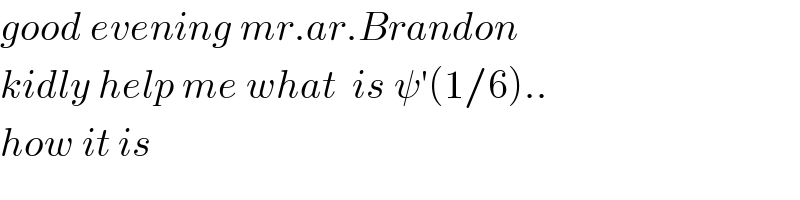calculate-0-logx-x-4-x-2-1-dx-

Question Number 136036 by mathmax by abdo last updated on 18/Mar/21

calculate \int_{0}^{\infty} \frac{logx}{x^{4} + x^{2} + 1} dx

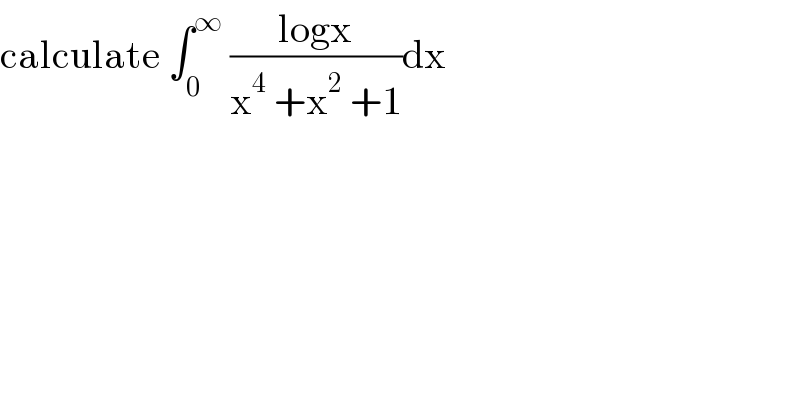
Commented by Ajetunmobi last updated on 18/Mar/21

hi sir how can we chat privately ?

Commented by Ajetunmobi last updated on 18/Mar/21

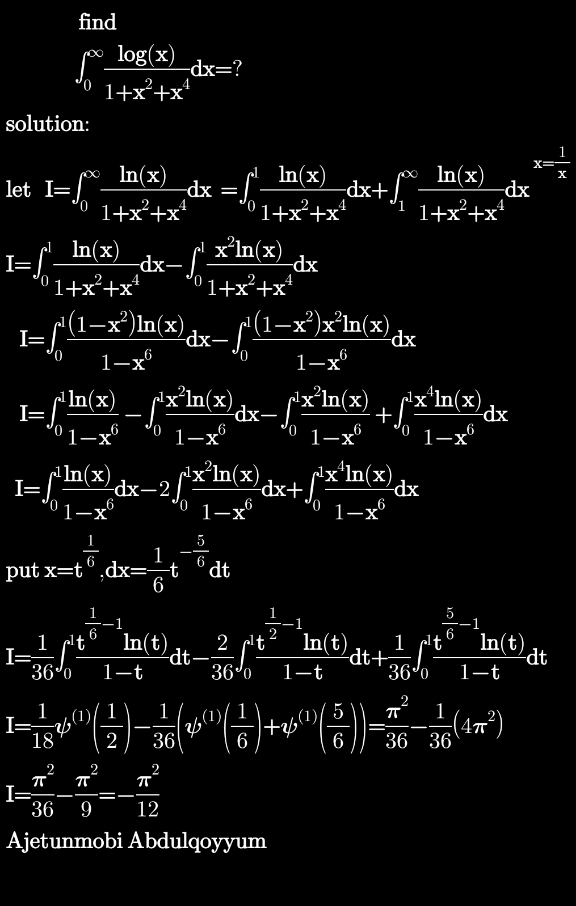
Commented by liki last updated on 19/Mar/21

Drop your whatssap number here!

Commented by mathmax by abdo last updated on 31/May/21

you are right liki \dots

Answered by Ar Brandon last updated on 18/Mar/21

Δ = \int_{0}^{\infty} \frac{lnx}{x^{4} + x^{2} + 1} dx

= \int_{0}^{1} \frac{lnx}{x^{4} + x^{2} + 1} dx + \int_{1}^{\infty} \frac{lnx}{x^{4} + x^{2} + 1} dx

= \int_{0}^{1} \frac{lnx}{x^{4} + x^{2} + 1} dx - \int_{0}^{1} \frac{x^{2} lnx}{x^{4} + x^{2} + 1} dx

= \int_{0}^{1} \frac{(1 - x^{2}) lnx}{(1 - x^{6})} dx - \int_{0}^{1} \frac{x^{2} (1 - x^{2}) lnx}{(1 - x^{6})} dx

= \frac{1}{36} \int_{0}^{1} \frac{(u^{- \frac{5}{6}} - u^{- \frac{1}{2}}) lnu}{1 - u} du - \frac{1}{36} \int_{0}^{1} \frac{(u^{- \frac{1}{2}} - u^{- \frac{1}{6}}) lnu}{1 - u} du

= - \frac{1}{36} {ψ^{1} (\frac{1}{6}) - ψ^{1} (\frac{1}{2}) - ψ^{1} (\frac{1}{2}) + ψ^{1} (\frac{5}{6})}

= - \frac{1}{36} {\frac{π^{2}}{\sin^{2} (\frac{π}{6})} - 2 ψ^{1} (\frac{1}{2})} = - \frac{1}{36} {4 π^{2} - 2 \times 4 \times \frac{3}{4} ζ (2)}

= - \frac{(4 π^{2} - π^{2})}{36} = - \frac{π^{2}}{12} ★

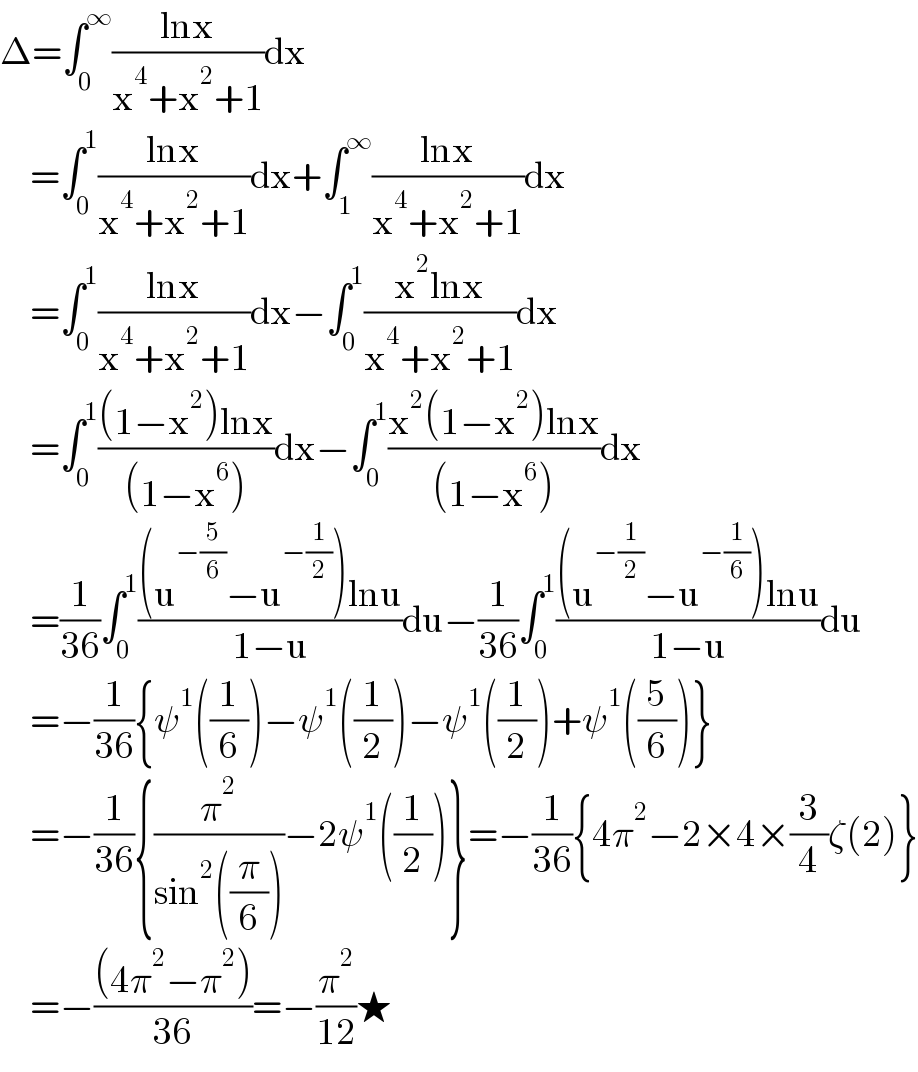
Commented by Dwaipayan Shikari last updated on 18/Mar/21

Commented by Ajetunmobi last updated on 18/Mar/21

you did the same solution as mine

but why ?

Commented by Ar Brandon last updated on 18/Mar/21

Because I wanna learn from you too .

Just as I do with the other members .

Commented by Ajetunmobi last updated on 18/Mar/21

ok sir we are all learnig from each

other

Commented by Ar Brandon last updated on 18/Mar/21

Commented by SLVR last updated on 13/Apr/21

g o o d e v e n i n g m r . a r . B r a n d o n

k i d l y h e l p m e w h a t i s ψ^{'} (1 / 6) . .

h o w i t i s

Facebook Tweet Pin