Menu Close

calculate-x-2-dx-x-2-x-3-2-

Tinku Tara 0 Comments
Pin




Question Number 142869 by mathmax by abdo last updated on 06/Jun/21
calculate ∫_(−∞) ^(+∞) ((x^2 dx)/((x^2 −x+3)^2 ))
$$\mathrm{calculate}\:\int_{−\infty} ^{+\infty} \:\:\frac{\mathrm{x}^{\mathrm{2}} \mathrm{dx}}{\left(\mathrm{x}^{\mathrm{2}} −\mathrm{x}+\mathrm{3}\right)^{\mathrm{2}} } \\ $$
Answered by Ar Brandon last updated on 06/Jun/21
Ψ=∫_(−∞) ^∞ ((x^2 dx)/((x^2 −x+3)^2 )) ∫((x^2 dx)/((x^2 −x+3)^2 ))=((ax+b)/(x^2 −x+3))+∫((cx+d)/(x^2 −x+3))dx ⇒((x^2 dx)/((x^2 −x+3)^2 ))=((a(x^2 −x+3)−(ax+b)(2x−1))/((x^2 −x+3)^2 ))+(((cx+d)(x^2 −x+3))/((x^2 −x+3)^2 )) c=0, a−2a−c+d=1⇒d=a+1, −a+a−2b+3c−d=0⇒d=−2b 3a+b+3d=0⇒6a−a−1+6a+6=0⇒a=−(5/(11)), d=(6/(11)), b=−(3/(11)) Ψ=[−((5x+3)/(11(x^2 −x+3)))+(6/(11))∫(dx/(x^2 −x+3))]_(−∞) ^∞ =(6/(11))∫_(−∞) ^∞ (dx/((x−(1/2))^2 +((11)/4)))=[(6/(11))∙(2/( (√(11))))arctan(((2x−1)/( (√(11)))) )]_(−∞) ^∞ =((12(√(11)))/(121))π
$$\Psi=\int_{−\infty} ^{\infty} \frac{\mathrm{x}^{\mathrm{2}} \mathrm{dx}}{\left(\mathrm{x}^{\mathrm{2}} −\mathrm{x}+\mathrm{3}\right)^{\mathrm{2}} } \\ $$$$\int\frac{\mathrm{x}^{\mathrm{2}} \mathrm{dx}}{\left(\mathrm{x}^{\mathrm{2}} −\mathrm{x}+\mathrm{3}\right)^{\mathrm{2}} }=\frac{\mathrm{ax}+\mathrm{b}}{\mathrm{x}^{\mathrm{2}} −\mathrm{x}+\mathrm{3}}+\int\frac{\mathrm{cx}+\mathrm{d}}{\mathrm{x}^{\mathrm{2}} −\mathrm{x}+\mathrm{3}}\mathrm{dx} \\ $$$$\Rightarrow\frac{\mathrm{x}^{\mathrm{2}} \mathrm{dx}}{\left(\mathrm{x}^{\mathrm{2}} −\mathrm{x}+\mathrm{3}\right)^{\mathrm{2}} }=\frac{\mathrm{a}\left(\mathrm{x}^{\mathrm{2}} −\mathrm{x}+\mathrm{3}\right)−\left(\mathrm{ax}+\mathrm{b}\right)\left(\mathrm{2x}−\mathrm{1}\right)}{\left(\mathrm{x}^{\mathrm{2}} −\mathrm{x}+\mathrm{3}\right)^{\mathrm{2}} }+\frac{\left(\mathrm{cx}+\mathrm{d}\right)\left(\mathrm{x}^{\mathrm{2}} −\mathrm{x}+\mathrm{3}\right)}{\left(\mathrm{x}^{\mathrm{2}} −\mathrm{x}+\mathrm{3}\right)^{\mathrm{2}} } \\ $$$$\mathrm{c}=\mathrm{0},\:\mathrm{a}−\mathrm{2a}−\mathrm{c}+\mathrm{d}=\mathrm{1}\Rightarrow\mathrm{d}=\mathrm{a}+\mathrm{1},\:−\mathrm{a}+\mathrm{a}−\mathrm{2b}+\mathrm{3c}−\mathrm{d}=\mathrm{0}\Rightarrow\mathrm{d}=−\mathrm{2b} \\ $$$$\mathrm{3a}+\mathrm{b}+\mathrm{3d}=\mathrm{0}\Rightarrow\mathrm{6a}−\mathrm{a}−\mathrm{1}+\mathrm{6a}+\mathrm{6}=\mathrm{0}\Rightarrow\mathrm{a}=−\frac{\mathrm{5}}{\mathrm{11}},\:\mathrm{d}=\frac{\mathrm{6}}{\mathrm{11}},\:\mathrm{b}=−\frac{\mathrm{3}}{\mathrm{11}} \\ $$$$\Psi=\left[−\frac{\mathrm{5x}+\mathrm{3}}{\mathrm{11}\left(\mathrm{x}^{\mathrm{2}} −\mathrm{x}+\mathrm{3}\right)}+\frac{\mathrm{6}}{\mathrm{11}}\int\frac{\mathrm{dx}}{\mathrm{x}^{\mathrm{2}} −\mathrm{x}+\mathrm{3}}\right]_{−\infty} ^{\infty} \\ $$$$\:\:\:\:=\frac{\mathrm{6}}{\mathrm{11}}\int_{−\infty} ^{\infty} \frac{\mathrm{dx}}{\left(\mathrm{x}−\frac{\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{2}} +\frac{\mathrm{11}}{\mathrm{4}}}=\left[\frac{\mathrm{6}}{\mathrm{11}}\centerdot\frac{\mathrm{2}}{\:\sqrt{\mathrm{11}}}\mathrm{arctan}\left(\frac{\mathrm{2x}−\mathrm{1}}{\:\sqrt{\mathrm{11}}}\:\right)\right]_{−\infty} ^{\infty} \:=\frac{\mathrm{12}\sqrt{\mathrm{11}}}{\mathrm{121}}\pi \\ $$
Commented by mathmax by abdo last updated on 08/Jun/21
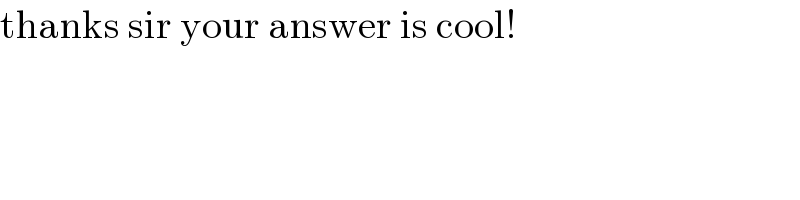
thanks sir your answer is cool!
$$\mathrm{thanks}\:\mathrm{sir}\:\mathrm{your}\:\mathrm{answer}\:\mathrm{is}\:\mathrm{cool}! \\ $$
Answered by mathmax by abdo last updated on 08/Jun/21
Φ=∫_(−∞) ^(+∞) ((x^2 dx)/((x^2 −x+3)^2 )) let ϕ(z)=(z^2 /((z^2 −z+3)^2 )) poles of ϕ? z^2 −z+3=0 →Δ=1−12=−11 →z_1 =((1+i(√(11)))/2) and z_2 =((1−i(√(11)))/2) ϕ(z)=(z^2 /((z−z_1 )^2 (z−z_2 )^2 )) residus theorem give ∫_(−∞) ^(+∞) ϕ(z)dz =2iπRes(ϕ,z_1 ) Res(ϕ,z_1 )=lim_(z→z_1 ) (1/((2−1)!)){(z−z_1 )^2 ϕ(z)}^((1)) =lim_(z→z_1 ) {(z^2 /((z−z_2 )^2 ))}^((1)) =lim_(z→z_1 ) ((2z(z−z_2 )^2 −2z^2 (z−z_2 ))/((z−z_2 )^4 )) =lim_(z→z_1 ) ((2z(z−z_2 )−2z^2 )/((z−z_2 )^3 )) =((2z_1 (z_1 −z_2 )−2z_1 ^2 )/((z_1 −z_2 )^3 )) =((−2z_1 z_2 )/((z_1 −z_2 )^3 )) =((−6)/((i(√(11)))^3 )) =((−6)/(−i11(√(11))))=((−6i)/(11(√(11)))) ⇒ ∫_(−∞) ^(+∞) ϕ(z)dz=2iπ×((−6i)/(11(√(11)))) =((12π)/(11(√(11)))) ⇒ Φ=((12π)/(11(√(11))))
$$\Phi=\int_{−\infty} ^{+\infty} \:\frac{\mathrm{x}^{\mathrm{2}} \mathrm{dx}}{\left(\mathrm{x}^{\mathrm{2}} −\mathrm{x}+\mathrm{3}\right)^{\mathrm{2}} }\:\mathrm{let}\:\varphi\left(\mathrm{z}\right)=\frac{\mathrm{z}^{\mathrm{2}} }{\left(\mathrm{z}^{\mathrm{2}} −\mathrm{z}+\mathrm{3}\right)^{\mathrm{2}} }\:\:\mathrm{poles}\:\mathrm{of}\:\varphi? \\ $$$$\mathrm{z}^{\mathrm{2}} −\mathrm{z}+\mathrm{3}=\mathrm{0}\:\rightarrow\Delta=\mathrm{1}−\mathrm{12}=−\mathrm{11}\:\rightarrow\mathrm{z}_{\mathrm{1}} =\frac{\mathrm{1}+\mathrm{i}\sqrt{\mathrm{11}}}{\mathrm{2}}\:\mathrm{and}\:\mathrm{z}_{\mathrm{2}} =\frac{\mathrm{1}−\mathrm{i}\sqrt{\mathrm{11}}}{\mathrm{2}} \\ $$$$\varphi\left(\mathrm{z}\right)=\frac{\mathrm{z}^{\mathrm{2}} }{\left(\mathrm{z}−\mathrm{z}_{\mathrm{1}} \right)^{\mathrm{2}} \left(\mathrm{z}−\mathrm{z}_{\mathrm{2}} \right)^{\mathrm{2}} }\:\mathrm{residus}\:\mathrm{theorem}\:\mathrm{give}\: \\ $$$$\int_{−\infty} ^{+\infty} \:\varphi\left(\mathrm{z}\right)\mathrm{dz}\:=\mathrm{2i}\pi\mathrm{Res}\left(\varphi,\mathrm{z}_{\mathrm{1}} \right) \\ $$$$\mathrm{Res}\left(\varphi,\mathrm{z}_{\mathrm{1}} \right)=\mathrm{lim}_{\mathrm{z}\rightarrow\mathrm{z}_{\mathrm{1}} } \:\:\:\frac{\mathrm{1}}{\left(\mathrm{2}−\mathrm{1}\right)!}\left\{\left(\mathrm{z}−\mathrm{z}_{\mathrm{1}} \right)^{\mathrm{2}} \varphi\left(\mathrm{z}\right)\right\}^{\left(\mathrm{1}\right)} \\ $$$$=\mathrm{lim}_{\mathrm{z}\rightarrow\mathrm{z}_{\mathrm{1}} } \:\:\:\left\{\frac{\mathrm{z}^{\mathrm{2}} }{\left(\mathrm{z}−\mathrm{z}_{\mathrm{2}} \right)^{\mathrm{2}} }\right\}^{\left(\mathrm{1}\right)} =\mathrm{lim}_{\mathrm{z}\rightarrow\mathrm{z}_{\mathrm{1}} } \:\:\frac{\mathrm{2z}\left(\mathrm{z}−\mathrm{z}_{\mathrm{2}} \right)^{\mathrm{2}} −\mathrm{2z}^{\mathrm{2}} \left(\mathrm{z}−\mathrm{z}_{\mathrm{2}} \right)}{\left(\mathrm{z}−\mathrm{z}_{\mathrm{2}} \right)^{\mathrm{4}} } \\ $$$$=\mathrm{lim}_{\mathrm{z}\rightarrow\mathrm{z}_{\mathrm{1}} } \:\:\:\frac{\mathrm{2z}\left(\mathrm{z}−\mathrm{z}_{\mathrm{2}} \right)−\mathrm{2z}^{\mathrm{2}} }{\left(\mathrm{z}−\mathrm{z}_{\mathrm{2}} \right)^{\mathrm{3}} }\:=\frac{\mathrm{2z}_{\mathrm{1}} \left(\mathrm{z}_{\mathrm{1}} −\mathrm{z}_{\mathrm{2}} \right)−\mathrm{2z}_{\mathrm{1}} ^{\mathrm{2}} }{\left(\mathrm{z}_{\mathrm{1}} −\mathrm{z}_{\mathrm{2}} \right)^{\mathrm{3}} } \\ $$$$=\frac{−\mathrm{2z}_{\mathrm{1}} \mathrm{z}_{\mathrm{2}} }{\left(\mathrm{z}_{\mathrm{1}} −\mathrm{z}_{\mathrm{2}} \right)^{\mathrm{3}} }\:=\frac{−\mathrm{6}}{\left(\mathrm{i}\sqrt{\mathrm{11}}\right)^{\mathrm{3}} }\:=\frac{−\mathrm{6}}{−\mathrm{i11}\sqrt{\mathrm{11}}}=\frac{−\mathrm{6i}}{\mathrm{11}\sqrt{\mathrm{11}}}\:\:\Rightarrow \\ $$$$\int_{−\infty} ^{+\infty} \:\varphi\left(\mathrm{z}\right)\mathrm{dz}=\mathrm{2i}\pi×\frac{−\mathrm{6i}}{\mathrm{11}\sqrt{\mathrm{11}}}\:=\frac{\mathrm{12}\pi}{\mathrm{11}\sqrt{\mathrm{11}}}\:\Rightarrow \\ $$$$\Phi=\frac{\mathrm{12}\pi}{\mathrm{11}\sqrt{\mathrm{11}}} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *