call-lim-n-H-n-ln-n-proof-that-is-finite-and-0-1-

Question Number 2783 by 123456 last updated on 27/Nov/15

Double subscripts: use braces to clarify

proof that γ is finite and γ \in (0, 1)

Commented by Filup last updated on 27/Nov/15

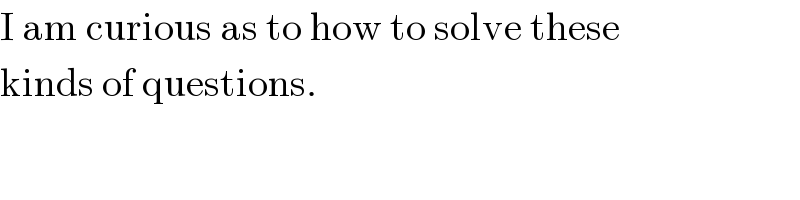
I am curious as to how to solve these

kinds of questions .

Commented by Filup last updated on 27/Nov/15

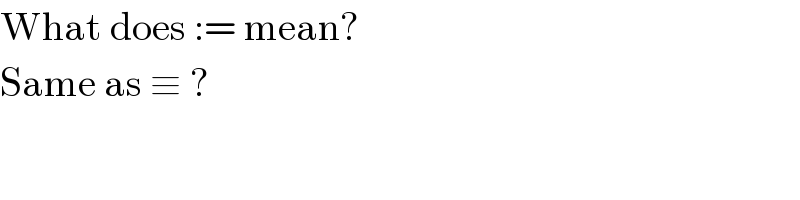
What does := mean ?

Same as \equiv ?

Commented by 123456 last updated on 27/Nov/15

:= mean defined

ex :

f (x) := x

f (1) = 1

Commented by Filup last updated on 27/Nov/15

A h I see!

Answered by prakash jain last updated on 27/Nov/15

γ_{n} = H_{n} - \ln n

H_{n} = 1 + \frac{1}{2} + \frac{1}{3} + . . + \frac{1}{n} = \underset{i = 1}{\sum^{n}} a_{i}

a_{i} = \frac{1}{i}

f (x) = \frac{1}{x} so that f (i) = a_{i}

Since f (x) is strictly decreasing + ve function .

From integral test for series

\int_{N}^{M + 1} f (x) d x ⩽ \underset{n = N}{\sum^{M}} f (n) ⩽ f (N) + \int_{N}^{M} f (x) d x \dots (A)

H_{n - 1} ⩾ \int_{1}^{n} \frac{1}{x} d x = \ln n

S o γ_{n} = H_{n} - \ln n = \frac{1}{n} + H_{n - 1} - \ln n > 0 \dots (1)

γ_{n + 1} = γ_{n} + \frac{1}{n + 1} - \ln (n + 1) + \ln n

\frac{1}{n + 1} ⩽ \ln \frac{n + 1}{n} (c o m p a r i n g a r e a o f r e c t a n g l e s)

\Rightarrow γ_{n + 1} = γ_{n} - [\ln \frac{n + 1}{n} - \frac{1}{n + 1}] < γ_{n}

γ_{n} > 0 a n d γ_{n + 1} < γ_{n}

So \lim_{n \to \infty} γ_{n} e x i s t s and > 0 .

γ_{1} = 1 - \ln 1 = 1

∵ γ_{n + 1} < γ_{n}

0 < γ < 1

Commented by RasheedAhmad last updated on 29/Nov/15

W h a t i s H_{n} ?

Commented by 123456 last updated on 29/Nov/15

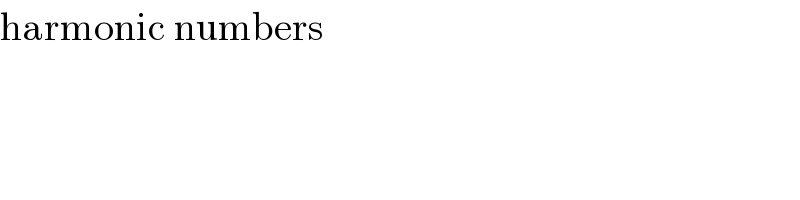
harmonic numbers

Commented by Rasheed Soomro last updated on 29/Nov/15

THANKS!