Question Number 77089 by Dah Solu Tion last updated on 03/Jan/20
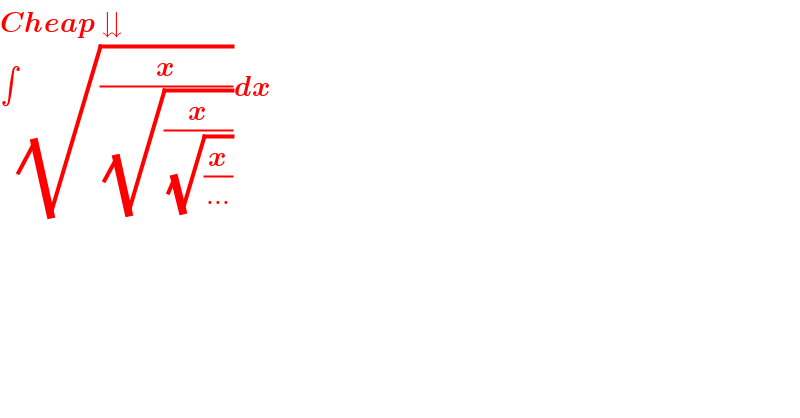
$$\boldsymbol{{Cheap}}\:\downdownarrows \\ $$$$\int\sqrt{\frac{\boldsymbol{{x}}}{\:\sqrt{\frac{\boldsymbol{{x}}}{\:\sqrt{\frac{\boldsymbol{{x}}}{…}}}}}}\boldsymbol{{dx}} \\ $$$$ \\ $$$$ \\ $$
Commented by Tony Lin last updated on 03/Jan/20
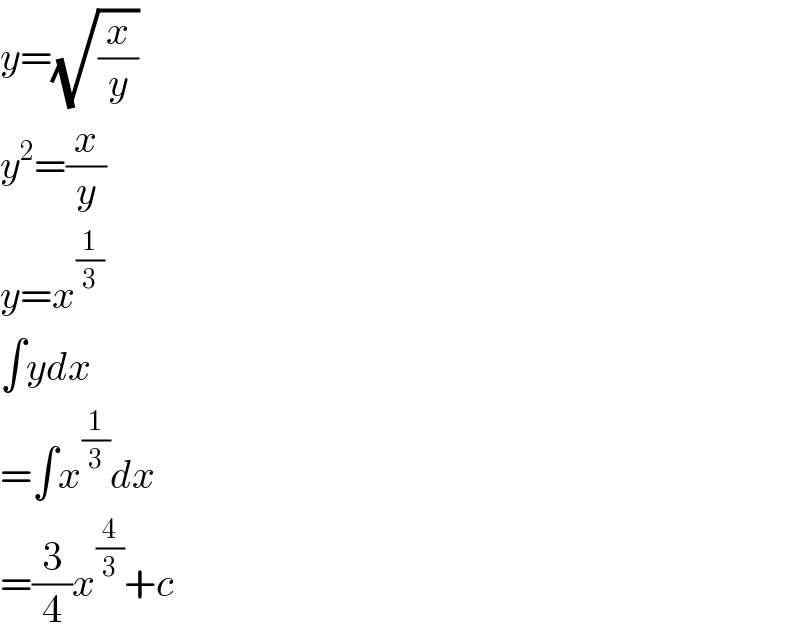
$${y}=\sqrt{\frac{{x}}{{y}}} \\ $$$${y}^{\mathrm{2}} =\frac{{x}}{{y}} \\ $$$${y}={x}^{\frac{\mathrm{1}}{\mathrm{3}}} \\ $$$$\int{ydx} \\ $$$$=\int{x}^{\frac{\mathrm{1}}{\mathrm{3}}} {dx} \\ $$$$=\frac{\mathrm{3}}{\mathrm{4}}{x}^{\frac{\mathrm{4}}{\mathrm{3}}} +{c} \\ $$
Commented by Dah Solu Tion last updated on 03/Jan/20

$${Nice}\:{one}\:{boss} \\ $$
