Question Number 138691 by liberty last updated on 16/Apr/21
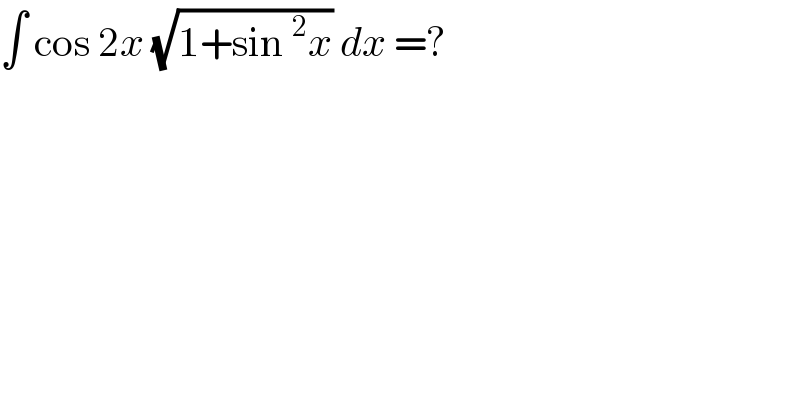
$$\int\:\mathrm{cos}\:\mathrm{2}{x}\:\sqrt{\mathrm{1}+\mathrm{sin}\:^{\mathrm{2}} {x}}\:{dx}\:=? \\ $$
Answered by mathmax by abdo last updated on 17/Apr/21
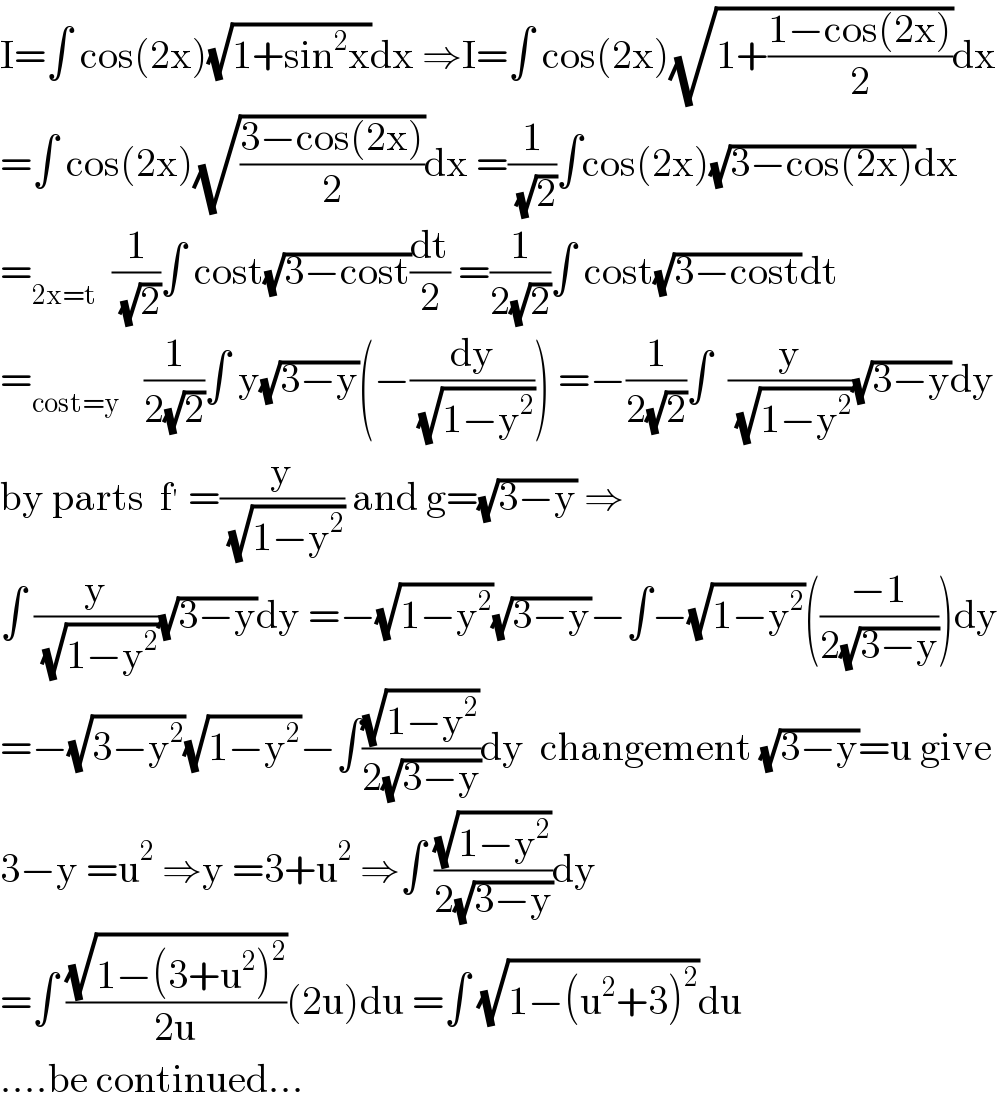
$$\mathrm{I}=\int\:\mathrm{cos}\left(\mathrm{2x}\right)\sqrt{\mathrm{1}+\mathrm{sin}^{\mathrm{2}} \mathrm{x}}\mathrm{dx}\:\Rightarrow\mathrm{I}=\int\:\mathrm{cos}\left(\mathrm{2x}\right)\sqrt{\mathrm{1}+\frac{\mathrm{1}−\mathrm{cos}\left(\mathrm{2x}\right)}{\mathrm{2}}}\mathrm{dx} \\ $$$$=\int\:\mathrm{cos}\left(\mathrm{2x}\right)\sqrt{\frac{\mathrm{3}−\mathrm{cos}\left(\mathrm{2x}\right)}{\mathrm{2}}}\mathrm{dx}\:=\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}\int\mathrm{cos}\left(\mathrm{2x}\right)\sqrt{\mathrm{3}−\mathrm{cos}\left(\mathrm{2x}\right)}\mathrm{dx} \\ $$$$=_{\mathrm{2x}=\mathrm{t}} \:\:\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}\int\:\mathrm{cost}\sqrt{\mathrm{3}−\mathrm{cost}}\frac{\mathrm{dt}}{\mathrm{2}}\:=\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{2}}}\int\:\mathrm{cost}\sqrt{\mathrm{3}−\mathrm{cost}}\mathrm{dt} \\ $$$$=_{\mathrm{cost}=\mathrm{y}} \:\:\:\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{2}}}\int\:\mathrm{y}\sqrt{\mathrm{3}−\mathrm{y}}\left(−\frac{\mathrm{dy}}{\:\sqrt{\mathrm{1}−\mathrm{y}^{\mathrm{2}} }}\right)\:=−\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{2}}}\int\:\:\frac{\mathrm{y}}{\:\sqrt{\mathrm{1}−\mathrm{y}^{\mathrm{2}} }}\sqrt{\mathrm{3}−\mathrm{y}}\mathrm{dy} \\ $$$$\mathrm{by}\:\mathrm{parts}\:\:\mathrm{f}^{'} \:=\frac{\mathrm{y}}{\:\sqrt{\mathrm{1}−\mathrm{y}^{\mathrm{2}} }}\:\mathrm{and}\:\mathrm{g}=\sqrt{\mathrm{3}−\mathrm{y}}\:\Rightarrow \\ $$$$\int\:\frac{\mathrm{y}}{\:\sqrt{\mathrm{1}−\mathrm{y}^{\mathrm{2}} }}\sqrt{\mathrm{3}−\mathrm{y}}\mathrm{dy}\:=−\sqrt{\mathrm{1}−\mathrm{y}^{\mathrm{2}} }\sqrt{\mathrm{3}−\mathrm{y}}−\int−\sqrt{\mathrm{1}−\mathrm{y}^{\mathrm{2}} }\left(\frac{−\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{3}−\mathrm{y}}}\right)\mathrm{dy} \\ $$$$=−\sqrt{\mathrm{3}−\mathrm{y}^{\mathrm{2}} }\sqrt{\mathrm{1}−\mathrm{y}^{\mathrm{2}} }−\int\frac{\sqrt{\mathrm{1}−\mathrm{y}^{\mathrm{2}} }}{\mathrm{2}\sqrt{\mathrm{3}−\mathrm{y}}}\mathrm{dy}\:\:\mathrm{changement}\:\sqrt{\mathrm{3}−\mathrm{y}}=\mathrm{u}\:\mathrm{give} \\ $$$$\mathrm{3}−\mathrm{y}\:=\mathrm{u}^{\mathrm{2}} \:\Rightarrow\mathrm{y}\:=\mathrm{3}+\mathrm{u}^{\mathrm{2}} \:\Rightarrow\int\:\frac{\sqrt{\mathrm{1}−\mathrm{y}^{\mathrm{2}} }}{\mathrm{2}\sqrt{\mathrm{3}−\mathrm{y}}}\mathrm{dy} \\ $$$$=\int\:\frac{\sqrt{\mathrm{1}−\left(\mathrm{3}+\mathrm{u}^{\mathrm{2}} \right)^{\mathrm{2}} }}{\mathrm{2u}}\left(\mathrm{2u}\right)\mathrm{du}\:=\int\:\sqrt{\mathrm{1}−\left(\mathrm{u}^{\mathrm{2}} +\mathrm{3}\right)^{\mathrm{2}} }\mathrm{du} \\ $$$$….\mathrm{be}\:\mathrm{continued}… \\ $$
