Question Number 137192 by bobhans last updated on 30/Mar/21
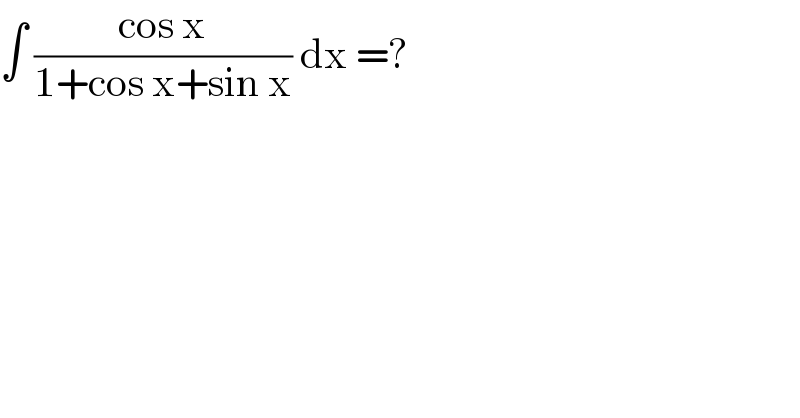
$$\int\:\frac{\mathrm{cos}\:\mathrm{x}}{\mathrm{1}+\mathrm{cos}\:\mathrm{x}+\mathrm{sin}\:\mathrm{x}}\:\mathrm{dx}\:=? \\ $$
Answered by bemath last updated on 30/Mar/21
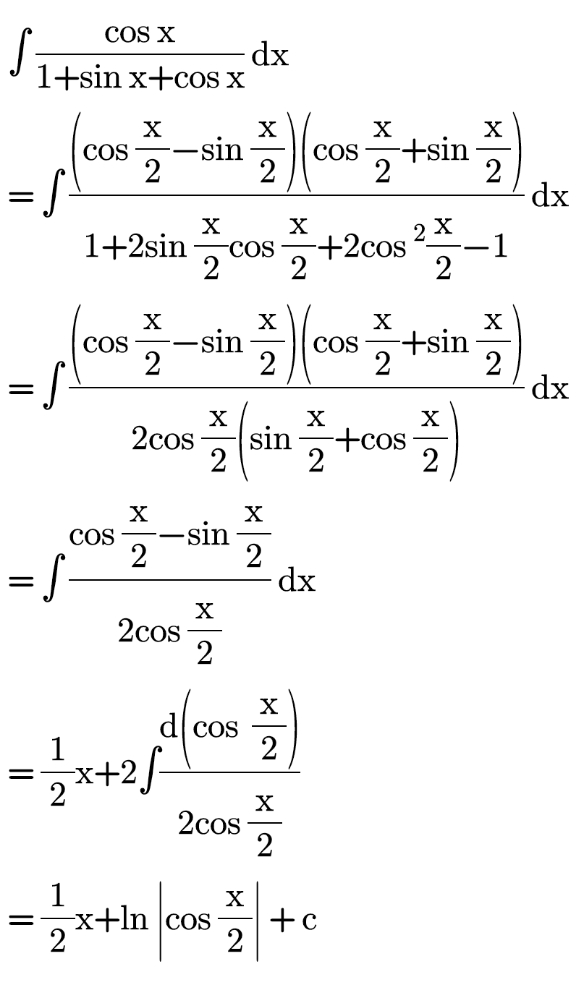
Answered by mathmax by abdo last updated on 30/Mar/21

$$\mathrm{I}\:=\int\:\frac{\mathrm{cosx}}{\mathrm{1}+\mathrm{cosx}+\mathrm{sinx}}\mathrm{dx}\:\mathrm{we}\:\mathrm{do}\:\mathrm{the}\:\mathrm{changement}\:\mathrm{tan}\left(\frac{\mathrm{x}}{\mathrm{2}}\right)=\mathrm{t}\:\Rightarrow \\ $$$$\mathrm{I}\:=\int\:\:\frac{\frac{\mathrm{1}−\mathrm{t}^{\mathrm{2}} }{\mathrm{1}+\mathrm{t}^{\mathrm{2}} }}{\mathrm{1}+\frac{\mathrm{1}−\mathrm{t}^{\mathrm{2}} }{\mathrm{1}+\mathrm{t}^{\mathrm{2}} }\:+\frac{\mathrm{2t}}{\mathrm{1}+\mathrm{t}^{\mathrm{2}} }}\frac{\mathrm{2dt}}{\mathrm{1}+\mathrm{t}^{\mathrm{2}} }\:=\mathrm{2}\int\:\:\:\frac{\mathrm{1}−\mathrm{t}^{\mathrm{2}} }{\left(\mathrm{1}+\mathrm{t}^{\mathrm{2}} \right)\left(\mathrm{1}+\mathrm{t}^{\mathrm{2}} \:+\mathrm{1}−\mathrm{t}^{\mathrm{2}} \:+\mathrm{2t}\right)}\mathrm{dt} \\ $$$$=\mathrm{2}\:\int\:\frac{\mathrm{1}−\mathrm{t}^{\mathrm{2}} }{\left(\mathrm{1}+\mathrm{t}^{\mathrm{2}} \right)\left(\mathrm{2}+\mathrm{2t}\right)}\:=\mathrm{2}\:\int\:\:\frac{\left(\mathrm{1}−\mathrm{t}\right)\left(\mathrm{1}+\mathrm{t}\right)}{\left(\mathrm{t}^{\mathrm{2}} \:+\mathrm{1}\right)\left(\mathrm{t}+\mathrm{1}\right)}\:=\mathrm{2}\:\int\frac{\mathrm{1}−\mathrm{t}}{\mathrm{1}+\mathrm{t}^{\mathrm{2}} }\mathrm{dt} \\ $$$$=\mathrm{2arctant}−\mathrm{ln}\left(\mathrm{1}+\mathrm{t}^{\mathrm{2}} \right)\:+\mathrm{C} \\ $$$$=\mathrm{2}.\frac{\mathrm{x}}{\mathrm{2}}−\mathrm{ln}\left(\mathrm{1}+\mathrm{tan}^{\mathrm{2}} \left(\frac{\mathrm{t}}{\mathrm{2}}\right)\right)\:+\mathrm{C}\:=\mathrm{x}−\mathrm{ln}\left(\mathrm{1}+\mathrm{tan}^{\mathrm{2}} \left(\frac{\mathrm{x}}{\mathrm{2}}\right)\right)\:+\mathrm{C} \\ $$
