Question Number 5882 by sanusihammed last updated on 03/Jun/16
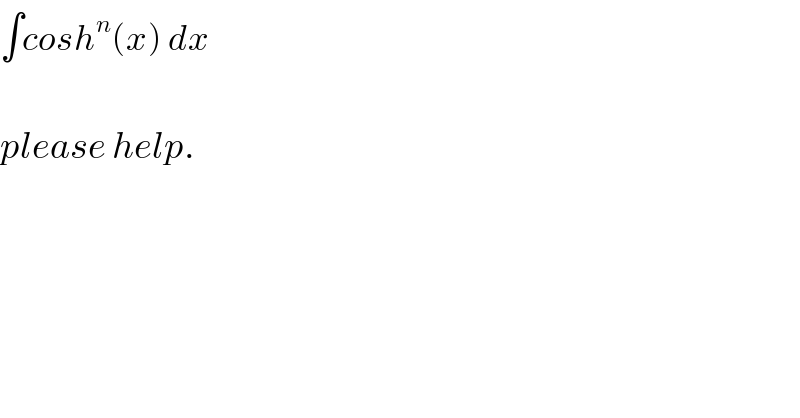
Answered by Yozzii last updated on 03/Jun/16
![cosh^n (x)=(((e^x +e^(−x) )/2))^n n∈N+{0} (assumed) cosh^n (x)=(1/2^n )Σ_(k=0) ^n { ((n),(k) )(e^x )^(n−k) (e^(−x) )^k } (Binomial theorem) cosh^n x=2^(−n) Σ_(k=0) ^n { ((n),(k) )e^(x(n−2k)) } For term independent of x, ⇒n−2k=0 or k=(n/2) where k∈Z. So,if n is even then a term independent of x exists and is given by 2^(−n) ((n),((n/2)) ). Define the set R={n,k∈Z^≥ , n≡0(mod 2)∣0≤k≤n and k≠(n/2)}. ⇒∫cosh^n xdx=∫{2^(−n) Σ_(k∈R) ((n),(k) )e^(x(n−2k)) +2^(−n) ((n),((n/2)) )}dx ∫cosh^n xdx=2^(−n) {Σ_(k∈R) [ ((n),(k) )(e^(x(n−2k)) /(n−2k))]+x ((n),((n/2)) )}+C This is valid for non−zero even n. If n=0⇒∫cosh^n xdx=x+C −−−−−−−−−−−−−−−−−−−−−−−−−− If n is odd then k=(n/2)∉Z, so that no term independent of x exists. ∴ ∫cosh^n xdx=2^(−n) Σ_(k=0) ^n [ ((n),(k) )(e^(x(n−2k)) /(n−2k))]+C −−−−−−−−−−−−−−−−−−−−−−−−−− In all, for n∈N+{0}, where R={k,n∈Z^≥ , n≡0(mod 2)∣0≤k≤n and k≠(n/2)} ∫cosh^n (x)dx= { ((2^(−n) {Σ_(k∈R) [ ((n),(k) )(e^(x(n−2k)) /(n−2k))]+ ((n),((n/2)) )x}+C if n≡0(mod 2) and n≥2)),((2^(−n) Σ_(k=0) ^n [ ((n),(k) )(e^(x(n−2k)) /(n−2k))]+C if n is odd)),((x+C if n=0)) :} C is a constant of integration.](https://www.tinkutara.com/question/Q5883.png)
Commented by Yozzii last updated on 03/Jun/16
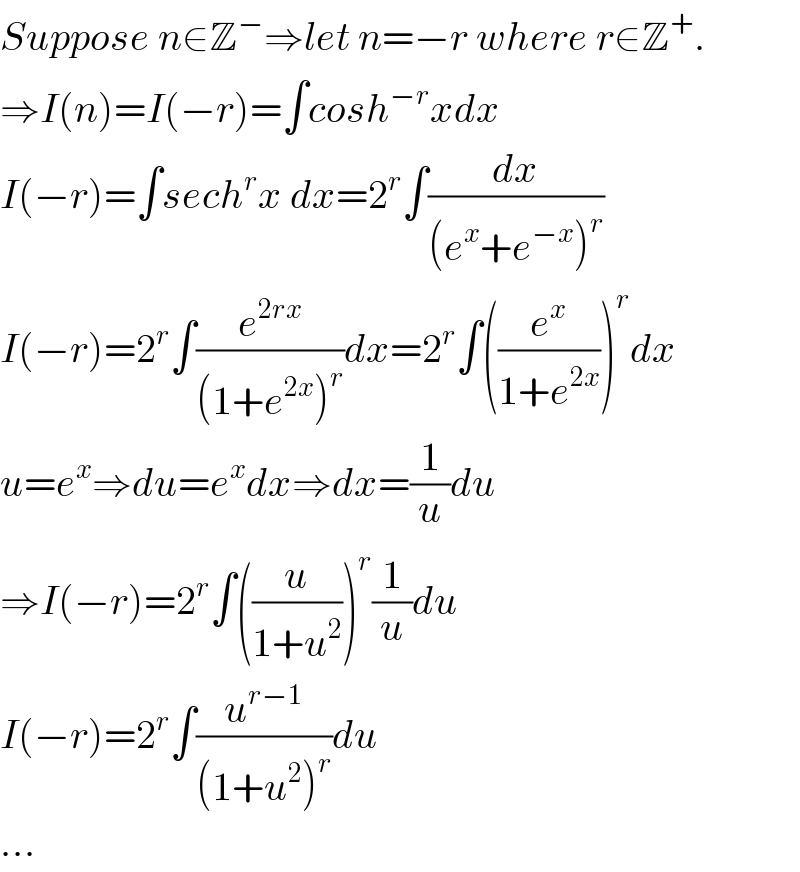
Commented by sanusihammed last updated on 03/Jun/16

Commented by sanusihammed last updated on 03/Jun/16

Commented by sanusihammed last updated on 03/Jun/16

Commented by Yozzii last updated on 03/Jun/16

Commented by sanusihammed last updated on 03/Jun/16