df-x-dx-f-x-x-Solve-f-x-

Question Number 6180 by FilupSmith last updated on 17/Jun/16

\frac{d f (x)}{d x} = f (x) + x

Solve f (x)

Commented by 123456 last updated on 17/Jun/16

\frac{d f}{d x} - f = x

f = f_{n} + f_{g}

\frac{{d f}_{n}}{d x} - f_{n} = 0 \Rightarrow f_{n} = {A e}^{x}

\frac{{d f}_{g}}{d x} - f_{g} = x

f_{g} = a x + b

\frac{{d f}_{g}}{d x} = a

a - (a x + b) = x

- a x + (a - b) = x

(- 1 - a) x + (a - b) = 0

- 1 - a = 0 \Rightarrow a = - 1

a - b = 0 \Rightarrow b = a = - 1

f_{g} = - x - 1

f = {A e}^{x} - x - 1

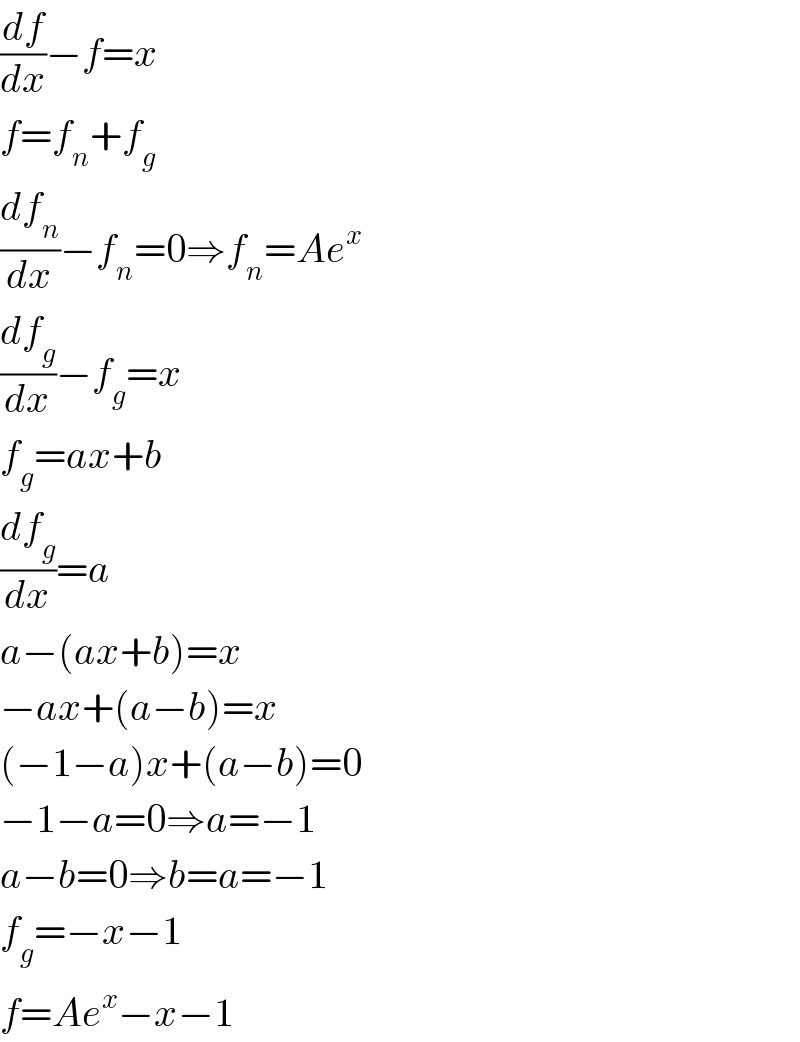
Commented by Rasheed Soomro last updated on 19/Jun/16

{D i d n}^{'} t u n d e r s t a n d

f = f_{n} + f_{g} (S u p p o s i t i o n ?)

\frac{{d f}_{n}}{d x} - f_{n} = 0, \frac{{d f}_{g}}{d x} - f_{g} = x (H o w ? W h y ?)

W h y o n e i s e q u a l t o 0 a n d o t h e r e q u a l t o x ?

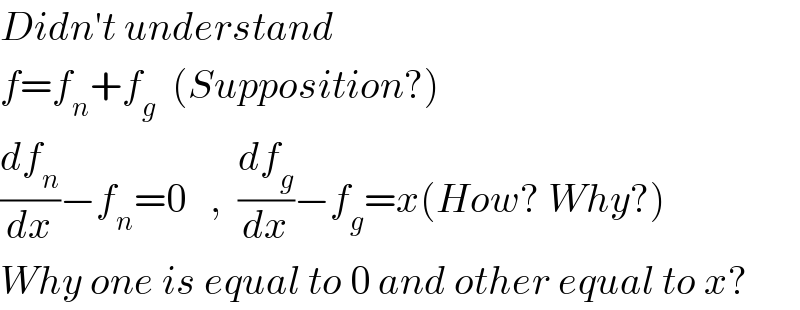
Commented by prakash jain last updated on 20/Jun/16

y^{'} - y = x

Let us say y = f_{1} is a solution such that

f_{1}^{'} - f_{1} = x

Also assume f_{2} is a solution such that

f_{2}^{'} - f_{2} = 0

Now for general solution of y

y = f_{1} + {k f}_{2}

y^{'} = f_{1}^{'} + {k f}_{2}^{'}

y^{'} - y = f_{1}^{'} + {k f}_{2}^{'} - f_{1} - f_{2}

= f_{1}^{'} - f_{1} + k (f_{2}^{'} - f_{2}) = x + k \cdot 0 = x

So general solution has 2 parts

homogenous solution (f_{2})

particular solution (f_{1})

a n y m u l t i p l e o f h o m e g e n o u s s o l u t i o n c a n

b e a d d e d t o p a r t i c u l a r s o l u t i o n a n d t h e s u m

w i l l s t i l l s a t i s f y d i f f e r e n t i a l e q u a t i o n .

Commented by Rasheed Soomro last updated on 21/Jun/16

TH AN X!