Question Number 69110 by Fawole last updated on 20/Sep/19
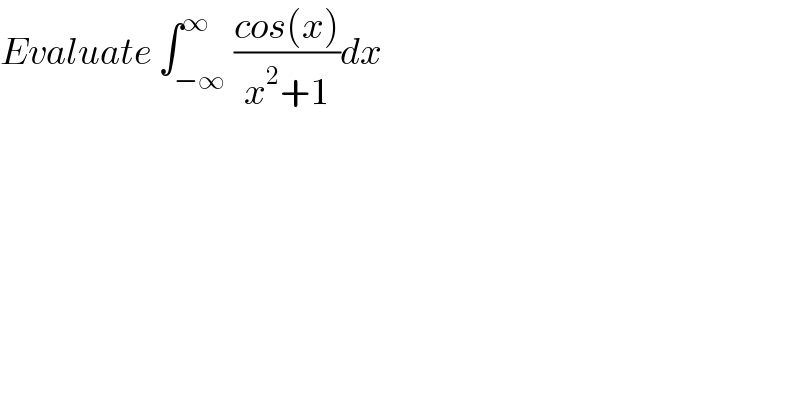
$${Evaluate}\:\int_{−\infty} ^{\infty} \frac{{cos}\left({x}\right)}{{x}^{\mathrm{2}} +\mathrm{1}}{dx} \\ $$
Commented by mathmax by abdo last updated on 20/Sep/19
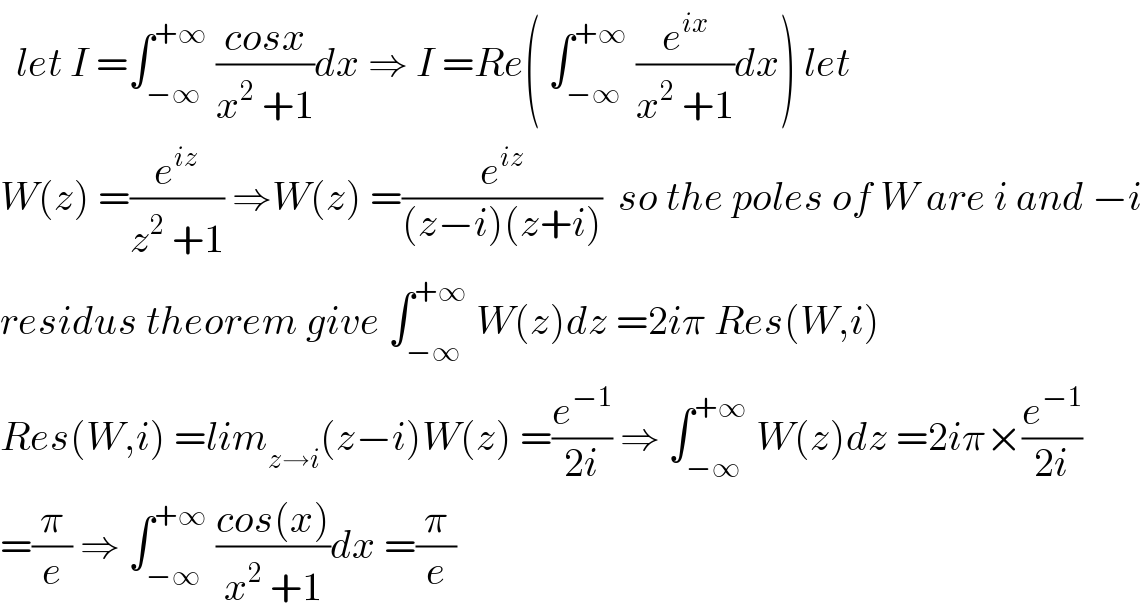
$$\:\:{let}\:{I}\:=\int_{−\infty} ^{+\infty} \:\frac{{cosx}}{{x}^{\mathrm{2}} \:+\mathrm{1}}{dx}\:\Rightarrow\:{I}\:={Re}\left(\:\int_{−\infty} ^{+\infty} \:\frac{{e}^{{ix}} }{{x}^{\mathrm{2}} \:+\mathrm{1}}{dx}\right)\:{let} \\ $$$${W}\left({z}\right)\:=\frac{{e}^{{iz}} }{{z}^{\mathrm{2}} \:+\mathrm{1}}\:\Rightarrow{W}\left({z}\right)\:=\frac{{e}^{{iz}} }{\left({z}−{i}\right)\left({z}+{i}\right)}\:\:{so}\:{the}\:{poles}\:{of}\:{W}\:{are}\:{i}\:{and}\:−{i} \\ $$$${residus}\:{theorem}\:{give}\:\int_{−\infty} ^{+\infty} \:{W}\left({z}\right){dz}\:=\mathrm{2}{i}\pi\:{Res}\left({W},{i}\right) \\ $$$${Res}\left({W},{i}\right)\:={lim}_{{z}\rightarrow{i}} \left({z}−{i}\right){W}\left({z}\right)\:=\frac{{e}^{−\mathrm{1}} }{\mathrm{2}{i}}\:\Rightarrow\:\int_{−\infty} ^{+\infty} \:{W}\left({z}\right){dz}\:=\mathrm{2}{i}\pi×\frac{{e}^{−\mathrm{1}} }{\mathrm{2}{i}} \\ $$$$=\frac{\pi}{{e}}\:\Rightarrow\:\int_{−\infty} ^{+\infty} \:\frac{{cos}\left({x}\right)}{{x}^{\mathrm{2}} \:+\mathrm{1}}{dx}\:=\frac{\pi}{{e}} \\ $$
Commented by Fawole last updated on 20/Sep/19

$${Nice}\:{solution} \\ $$
Commented by mathmax by abdo last updated on 20/Sep/19

$${thanks} \\ $$
