Evaluate-n-1-1-n-1-n-1-1-2-1-3-1-4-

Question Number 2489 by Filup last updated on 21/Nov/15

Evaluate :

\underset{n = 1}{\sum^{\infty}} (\frac{{(- 1)}^{n + 1}}{\sqrt{n}}) = 1 - \frac{1}{\sqrt{2}} + \frac{1}{\sqrt{3}} - \frac{1}{\sqrt{4}} + \dots

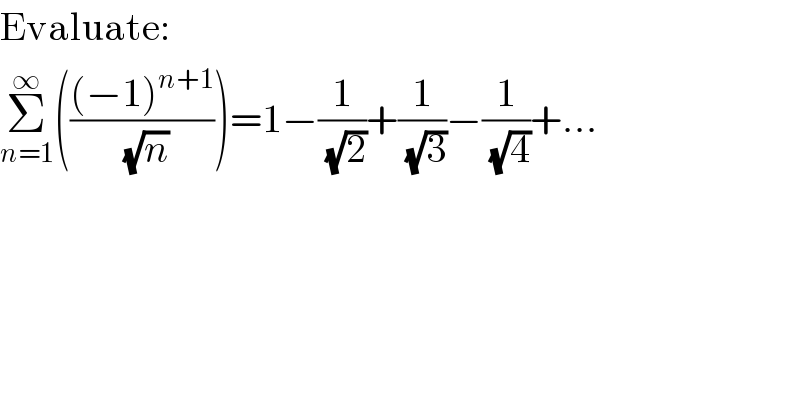
Commented by Yozzi last updated on 21/Nov/15

\underset{r = 1}{\sum^{\infty}} \frac{{(- 1)}^{r + 1}}{\sqrt{n}} = \underset{r = 1}{\sum^{\infty}} \frac{1}{\sqrt{2 r - 1}} - \underset{r = 1}{\sum^{\infty}} \frac{1}{\sqrt{2 r}}

L e t f (x) = {(2 x - 1)}^{- 1 / 2} . f i s d e c r e a s i n g

a n d p o s i t i v e f o r x ⩾ 1 s o t h e i n t e g r a l

t e s t c o u l d s h o w w h e t h e r \underset{r = 1}{\sum^{\infty}} \frac{1}{\sqrt{2 r - 1}} i s

c o n v e r g e n t o r n o t .

L e t I = \int_{1}^{\infty} f (x) d x = \lim_{m \to \infty} \int_{1}^{m} {(2 x - 1)}^{- 1 / 2} d x

I = \lim_{m \to \infty} (\frac{{(2 x - 1)}^{1 / 2}}{2 \times 1 / 2}) ∣_{1}^{m}

I = \lim_{m \to \infty} [\sqrt{2 m - 1} - \sqrt{2 \times 1 - 1}]

I = \lim_{m \to \infty} [\sqrt{2 m - 1} - 1] = \sqrt{2 \times \infty - 1} - 1 = \infty

T h u s, s i n c e I d o e s n o t e x i s t, \underset{r = 1}{\sum^{\infty}} \frac{1}{\sqrt{2 r - 1}}

i s d i v e r g e n t .

{H e n c e, \underset{r = 1}{\sum^{\infty}} \frac{{(- 1)}^{n + 1}}{\sqrt{n}} i s d i v e r g e n t .} (\times)

Answered by Yozzi last updated on 21/Nov/15

\underset{r = 1}{\sum^{\infty}} (\frac{{(- 1)}^{n + 1}}{\sqrt{n}}) d o e s n o t c o n v e r g e .

(I n c o r r e c t - d i s r e g a r d t h i s c o n t r i b u t i o n) .

Commented by prakash jain last updated on 21/Nov/15

The sequence may be conditinally convergent .

I think the tests in comment only prove

that it is not absolutely convergent .

Commented by 123456 last updated on 21/Nov/15

0 < a_{n + 1} < a_{n} (by alternating test it converge)

its coditionly converge since

Σ 1 / \sqrt{n}

diverge by p series test

Answered by prakash jain last updated on 21/Nov/15

η (s) = \underset{n = 1}{\sum^{\infty}} \frac{{(- 1)}^{n}}{n^{s}} (Dirichlet eta function)

The given series = η (\frac{1}{2})