Question Number 33 by user2 last updated on 25/Jan/15

$$\mathrm{Evaluate}\:\:\int\mathrm{sin}\:{x}\:\sqrt{\mathrm{1}−\mathrm{cos}\:\mathrm{2}{x}\:}{dx}. \\ $$
Answered by user2 last updated on 03/Nov/14
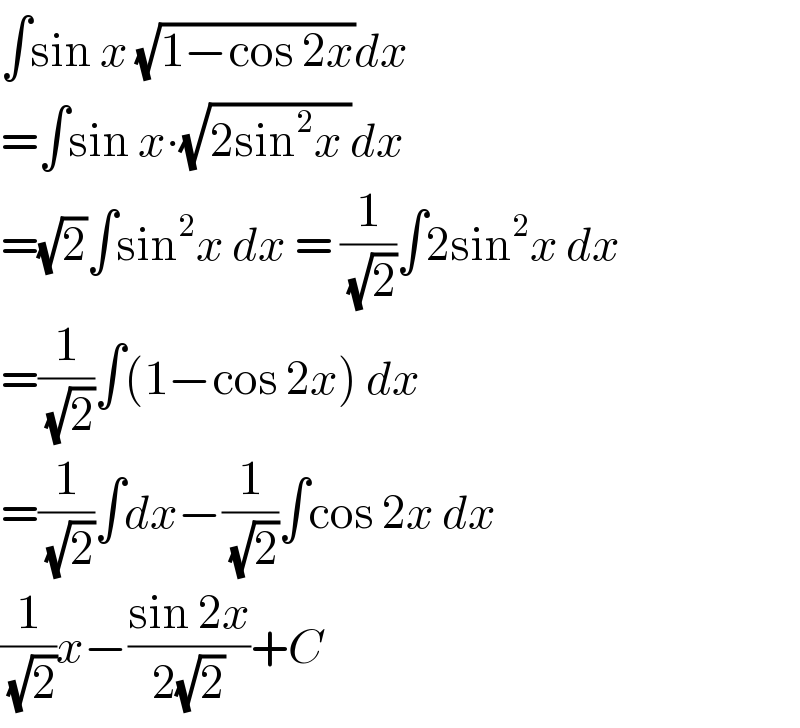
$$\int\mathrm{sin}\:{x}\:\sqrt{\mathrm{1}−\mathrm{cos}\:\mathrm{2}{x}}{dx} \\ $$$$=\int\mathrm{sin}\:{x}\centerdot\sqrt{\mathrm{2sin}^{\mathrm{2}} {x}\:}{dx}\: \\ $$$$=\sqrt{\mathrm{2}}\int\mathrm{sin}^{\mathrm{2}} {x}\:{dx}\:=\:\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}\int\mathrm{2sin}^{\mathrm{2}} {x}\:{dx} \\ $$$$=\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}\int\left(\mathrm{1}−\mathrm{cos}\:\mathrm{2}{x}\right)\:{dx} \\ $$$$=\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}\int{dx}−\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}\int\mathrm{cos}\:\mathrm{2}{x}\:{dx} \\ $$$$\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}{x}−\frac{\mathrm{sin}\:\mathrm{2}{x}}{\mathrm{2}\sqrt{\mathrm{2}}}+{C} \\ $$
