f-x-x-x-find-d-dx-f-x-and-for-what-x-we-will-get-the-maximum-of-the-function-

Question Number 11546 by Nayon last updated on 28/Mar/17

f (x) =^{x} \sqrt{x} f i n d \frac{d}{d x} (f (x))

a n d f o r w h a t x, w e w i l l g e t t h e

m a x i m u m o f t h e f u n c t i o n . . ?

Answered by sma3l2996 last updated on 28/Mar/17

\frac{d (f (x))}{d x} = \frac{d (\sqrt[x]{x})}{d x} = \frac{d (e^{l n (x^{\frac{1}{x}})})}{d x} = \frac{d (e^{\frac{l n (x)}{x}})}{d x}

= \frac{d (\frac{l n (x)}{x})}{d x} e^{\frac{l n (x)}{x}} = \frac{1 - l n (x)}{x^{2}} e^{l n (\sqrt[x]{x})}

= \frac{(1 - l n (x)) \sqrt[x]{x}}{x^{2}}

w h e n f g e t t h e m a x i m u m s o f^{'} (x) = 0

\frac{(1 - l n (x)) \sqrt[x]{x}}{x^{2}} = 0 a n d \sqrt[x]{x} = e^{\frac{l n (x)}{x}} > 0

1 - l n (x) = 0 \Leftrightarrow l n (x) = 1

x = e

Commented by Nayon last updated on 28/Mar/17

t h a n k s a l o t

Answered by Joel576 last updated on 28/Mar/17

y = x^{\frac{1}{x}}

\ln y = \frac{1}{x} . \ln x

\frac{d}{d y} (\ln y) = \frac{d}{d x} (\frac{1}{x} . \ln x)

\frac{d y}{d x} \frac{1}{y} = \frac{1}{x^{2}} - \frac{\ln x}{x^{2}}

\frac{d y}{d x} \frac{1}{y} = \frac{1 - \ln x}{x^{2}}

\frac{d y}{d x} = y (\frac{1 - \ln x}{x^{2}})

f^{'} (x) = \frac{d y}{d x} = \frac{x^{\frac{1}{x}} (1 - \ln x)}{x^{2}}

Function f (x) will be maximum if f^{'} (x) = 0

\frac{x^{\frac{1}{x}} (1 - \ln x)}{x^{2}} = 0

∙ x^{\frac{1}{x}} = 0 \to undefined (?)

∙ 1 - \ln x = 0

\ln x = 1

x = e

Commented by Nayon last updated on 28/Mar/17

i n t h e t h i r d l i n e \frac{d}{d x} (l n y) = \frac{d}{d x} (\frac{1}{x} l n x)

o r \frac{d}{d y} (l n y) = \frac{d}{d x} (\frac{1}{x} . l n x) ? ? ? ? ? ? ? ?

Commented by Joel576 last updated on 28/Mar/17

Differential at both section

\frac{d}{d y} (\ln y) = \frac{d}{d x} (\frac{1}{x} . \ln x)

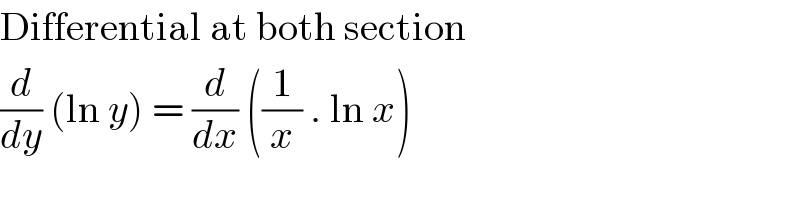
Commented by Nayon last updated on 28/Mar/17

w r o n g {i t}^{'} l l b e \frac{d}{d y} l n y . \frac{d y}{d x}

Facebook Tweet Pin