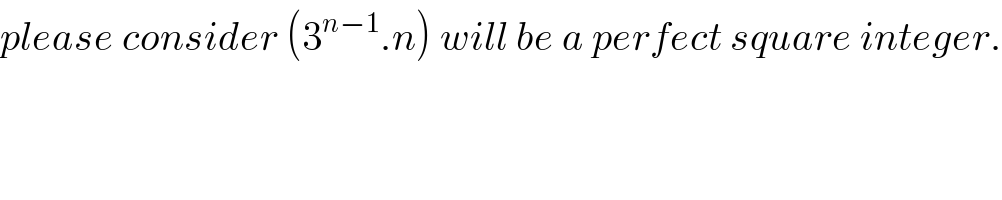Find-all-positive-integers-n-such-that-n-2014-and-3-n-1-n-will-be-a-perfect-square-integer-

Question Number 1379 by 314159 last updated on 27/Jul/15

F i n d a l l p o s i t i v e i n t e g e r s n s u c h t h a t

n ⩽ 2014 a n d 3^{n - 1} . n w i l l b e a p e r f e c t s q u a r e i n t e g e r .

Commented by 123456 last updated on 26/Jul/15

m \equiv 0, 1, 2, 3, 4 (\mod 5)

n = m^{2} \equiv 0, 1, 4 (\mod 5)

n - 1 \equiv 0 (\mod 2)

n \equiv 1 (\mod 2)

N . C : {\begin{cases} n \equiv 0 (\mod 5) \lor n \equiv 1 (\mod 5) \lor n \equiv 4 (\mod 5) \\ n \equiv 1 (\mod 2) \end{cases}

Commented by 314159 last updated on 27/Jul/15

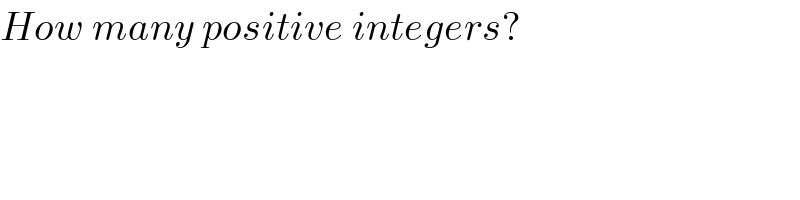
H o w m a n y p o s i t i v e i n t e g e r s ?

Commented by prakash jain last updated on 27/Jul/15

n = 3^{2 k} . {(2 l + 1)}^{2} k, l \in N

n o d d, 3^{n - 1} . 3^{2 k} . {(2 l + 1)}^{2} = 3^{(e v e n p o w e r)} . (w h o l e s q u a r e)

n = 3^{2 k - 1} . {(2 l)}^{2} k, l \in N

n e v e n, 3^{n - 1} . 3^{2 k - 1} . {(2 l)}^{2} = 3^{(e v e n p o w e r)} . (w h o l e s q u a r e)

choose k and l large enough so that n ⩾ 2014 .

There are infinitely many + ve integers satisfying

th condiion .

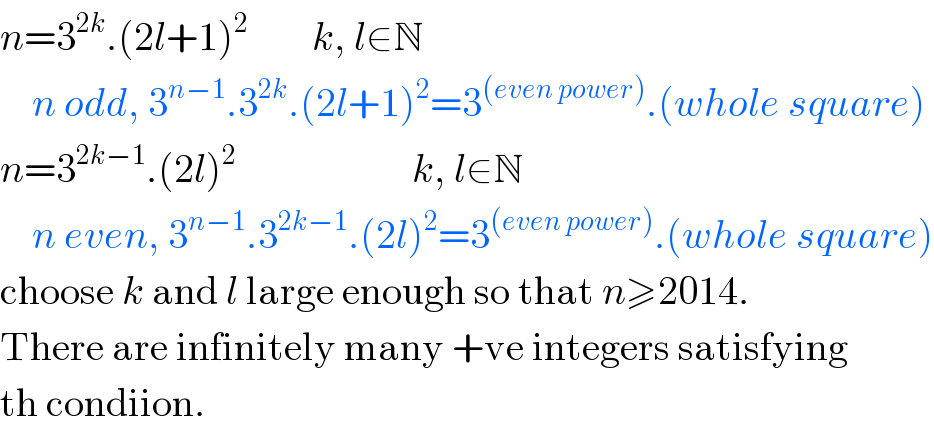
Commented by 314159 last updated on 27/Jul/15

p l e a s e . . c o n s i d e r n ⩽ 2014 \dots

s o r r y . . f o r m y m i s t a k e

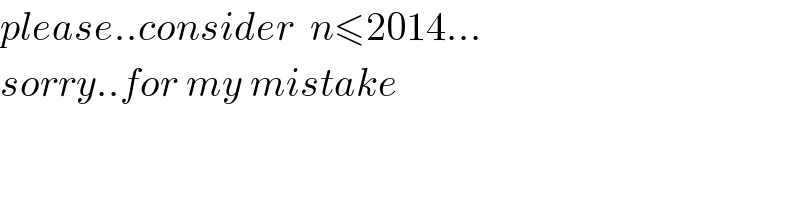
Commented by 314159 last updated on 28/Jul/15

p l e a s e c o n s i d e r (3^{n - 1} . n) w i l l b e a p e r f e c t s q u a r e i n t e g e r .

Facebook Tweet Pin