Find-grad-log-r-

Question Number 2 by user1 last updated on 25/Jan/15

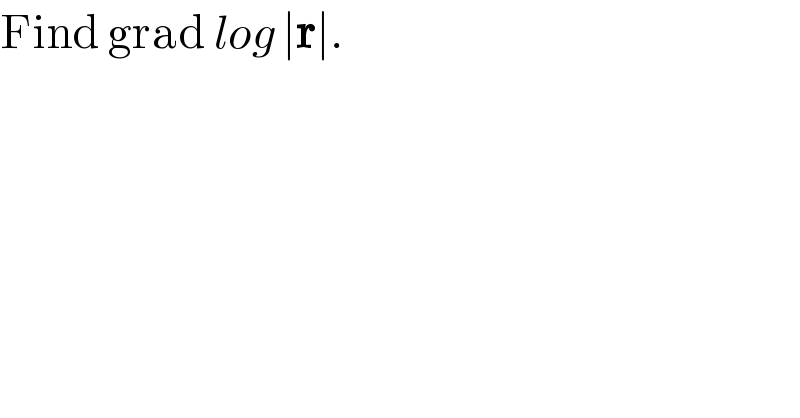
Find grad l o g ∣ r ∣ .

Answered by user1 last updated on 29/Oct/14

We have r = \sqrt{(x^{2} + y^{2} + z^{2})}

\log ∣ r ∣= \frac{1}{2} \log (x^{2} + y^{2} + z^{2})

Now, \frac{\partial}{\partial x} \log ∣ r ∣= \frac{1}{2} \cdot \frac{2 x}{(x^{2} + y^{2} + z^{2})} = \frac{x}{r^{2}}

Similarly, \frac{\partial}{\partial y} \log ∣ r ∣= \frac{y}{r^{2}}, \frac{\partial}{\partial z} \log ∣ r ∣= \frac{z}{r^{2}}

∴ grad \log ∣ r ∣= \frac{1}{r^{2}} (i x + j y + k z) = \frac{r}{r^{2}}

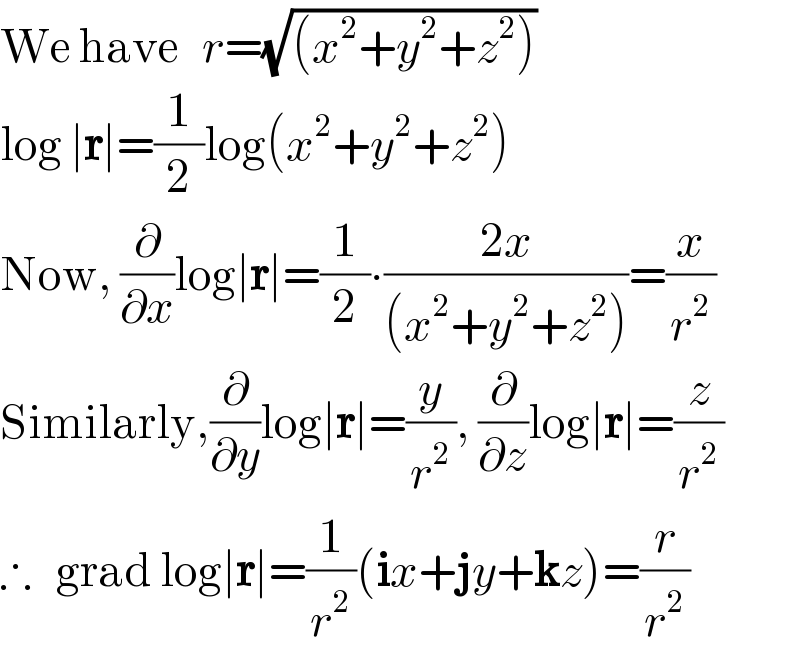
Commented by SWPlaysMC last updated on 16/Dec/21

Ahh yes, the first question ever asked on this app \dots

What happened to question ID 1 ? Did it EVER exist ?

E d i t : A l l h a i l u s e r 1!

Commented by talminator2856791 last updated on 05/Sep/21

no clue .

Commented by talminator2856791 last updated on 05/Sep/21

it appears that user 1 also doesnt

exist now .

Commented by MathematicalUser2357 last updated on 08/Jan/24

B U G