Question Number 143575 by Mathspace last updated on 15/Jun/21
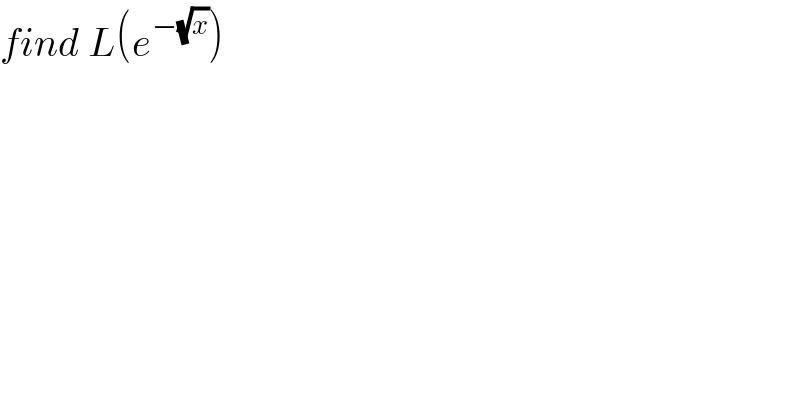
$${find}\:{L}\left({e}^{−\sqrt{{x}}} \right) \\ $$
Answered by Dwaipayan Shikari last updated on 15/Jun/21
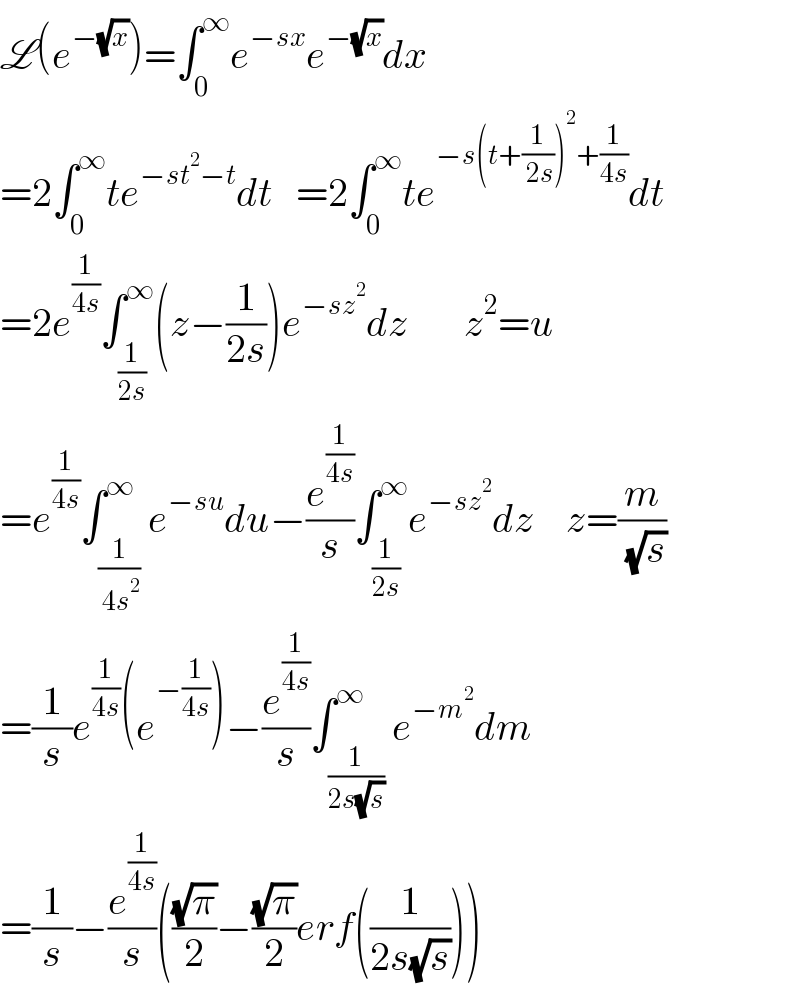
$$\mathscr{L}\left({e}^{−\sqrt{{x}}} \right)=\int_{\mathrm{0}} ^{\infty} {e}^{−{sx}} {e}^{−\sqrt{{x}}} {dx} \\ $$$$=\mathrm{2}\int_{\mathrm{0}} ^{\infty} {te}^{−{st}^{\mathrm{2}} −{t}} {dt}\:\:\:=\mathrm{2}\int_{\mathrm{0}} ^{\infty} {te}^{−{s}\left({t}+\frac{\mathrm{1}}{\:\mathrm{2}{s}}\right)^{\mathrm{2}} +\frac{\mathrm{1}}{\mathrm{4}{s}}} {dt}\: \\ $$$$=\mathrm{2}{e}^{\frac{\mathrm{1}}{\mathrm{4}{s}}} \int_{\frac{\mathrm{1}}{\mathrm{2}{s}}} ^{\infty} \left({z}−\frac{\mathrm{1}}{\mathrm{2}{s}}\right){e}^{−{sz}^{\mathrm{2}} } {dz}\:\:\:\:\:\:\:{z}^{\mathrm{2}} ={u} \\ $$$$={e}^{\frac{\mathrm{1}}{\mathrm{4}{s}}} \int_{\frac{\mathrm{1}}{\:\mathrm{4}{s}^{\mathrm{2}} }} ^{\infty} {e}^{−{su}} {du}−\frac{{e}^{\frac{\mathrm{1}}{\mathrm{4}{s}}} }{{s}}\int_{\frac{\mathrm{1}}{\mathrm{2}{s}}} ^{\infty} {e}^{−{sz}^{\mathrm{2}} } {dz}\:\:\:\:{z}=\frac{{m}}{\:\sqrt{{s}}} \\ $$$$=\frac{\mathrm{1}}{{s}}{e}^{\frac{\mathrm{1}}{\mathrm{4}{s}}} \left({e}^{−\frac{\mathrm{1}}{\mathrm{4}{s}}} \right)−\frac{{e}^{\frac{\mathrm{1}}{\mathrm{4}{s}}} }{{s}}\int_{\frac{\mathrm{1}}{\mathrm{2}{s}\sqrt{{s}}}} ^{\infty} {e}^{−{m}^{\mathrm{2}} } {dm} \\ $$$$=\frac{\mathrm{1}}{{s}}−\frac{{e}^{\frac{\mathrm{1}}{\mathrm{4}{s}}} }{{s}}\left(\frac{\sqrt{\pi}}{\mathrm{2}}−\frac{\sqrt{\pi}}{\mathrm{2}}{erf}\left(\frac{\mathrm{1}}{\mathrm{2}{s}\sqrt{{s}}}\right)\right) \\ $$
Commented by mathmax by abdo last updated on 16/Jun/21

$$\mathrm{thank}\:\mathrm{you}\:\mathrm{sir} \\ $$
