Menu Close

find-lim-x-0-sin-1-cosx-1-cos-sinx-x-2-

Tinku Tara 0 Comments
Pin




Question Number 143255 by Mathspace last updated on 12/Jun/21
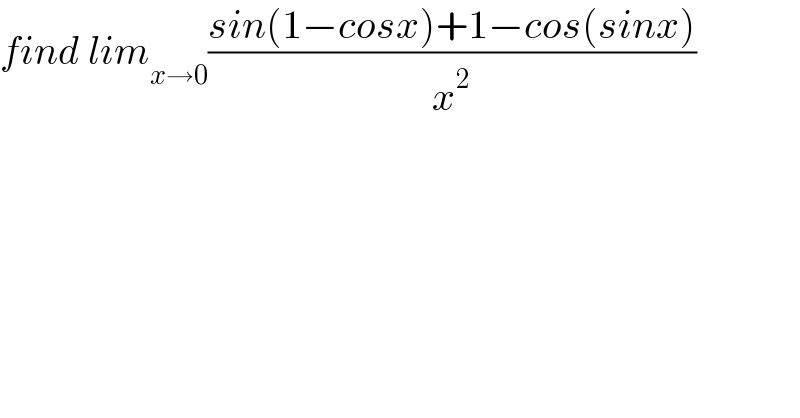
find lim_(x→0) ((sin(1−cosx)+1−cos(sinx))/x^2 )
$${find}\:{lim}_{{x}\rightarrow\mathrm{0}} \frac{{sin}\left(\mathrm{1}−{cosx}\right)+\mathrm{1}−{cos}\left({sinx}\right)}{{x}^{\mathrm{2}} } \\ $$
Answered by bramlexs22 last updated on 12/Jun/21
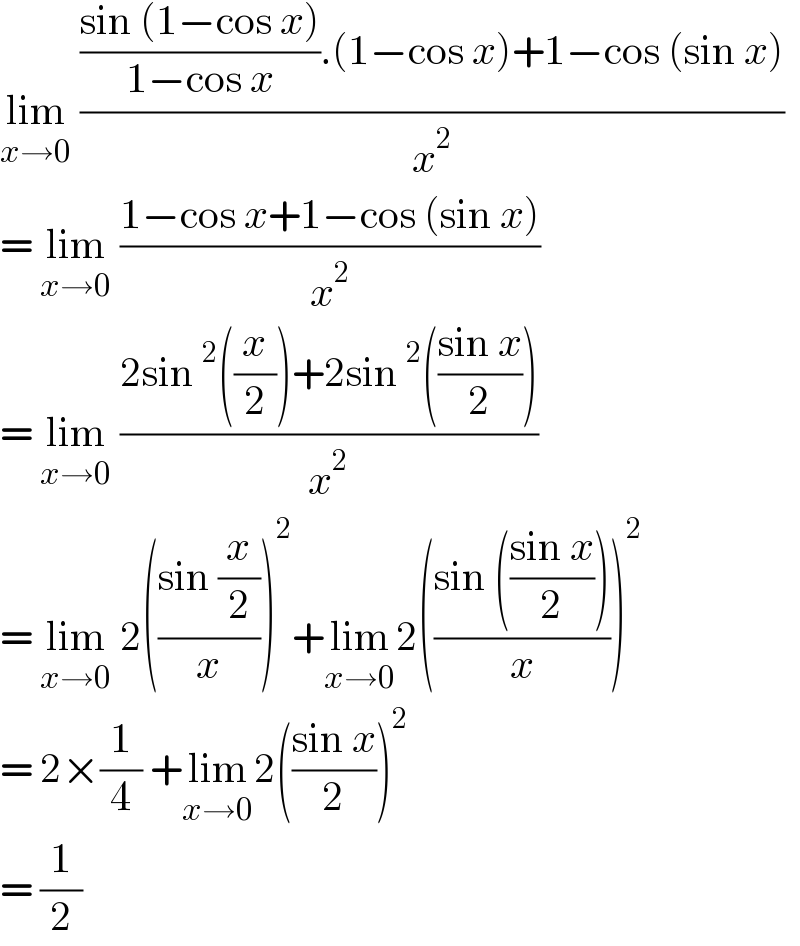
lim_(x→0) ((((sin (1−cos x))/(1−cos x)).(1−cos x)+1−cos (sin x))/x^2 ) = lim_(x→0) ((1−cos x+1−cos (sin x))/x^2 ) = lim_(x→0) ((2sin^2 ((x/2))+2sin^2 (((sin x)/2)))/x^2 ) = lim_(x→0) 2(((sin (x/2))/x))^2 +lim_(x→0) 2(((sin (((sin x)/2)))/x))^2 = 2×(1/4) +lim_(x→0) 2(((sin x)/2))^2 = (1/2)
$$\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\frac{\mathrm{sin}\:\left(\mathrm{1}−\mathrm{cos}\:{x}\right)}{\mathrm{1}−\mathrm{cos}\:{x}}.\left(\mathrm{1}−\mathrm{cos}\:{x}\right)+\mathrm{1}−\mathrm{cos}\:\left(\mathrm{sin}\:{x}\right)}{{x}^{\mathrm{2}} } \\ $$$$=\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\mathrm{1}−\mathrm{cos}\:{x}+\mathrm{1}−\mathrm{cos}\:\left(\mathrm{sin}\:{x}\right)}{{x}^{\mathrm{2}} } \\ $$$$=\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\mathrm{2sin}\:^{\mathrm{2}} \left(\frac{{x}}{\mathrm{2}}\right)+\mathrm{2sin}\:^{\mathrm{2}} \left(\frac{\mathrm{sin}\:{x}}{\mathrm{2}}\right)}{{x}^{\mathrm{2}} } \\ $$$$=\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\mathrm{2}\left(\frac{\mathrm{sin}\:\frac{{x}}{\mathrm{2}}}{{x}}\right)^{\mathrm{2}} +\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}2}\left(\frac{\mathrm{sin}\:\left(\frac{\mathrm{sin}\:{x}}{\mathrm{2}}\right)}{{x}}\right)^{\mathrm{2}} \\ $$$$=\:\mathrm{2}×\frac{\mathrm{1}}{\mathrm{4}}\:+\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}2}\left(\frac{\mathrm{sin}\:{x}}{\mathrm{2}}\right)^{\mathrm{2}} \\ $$$$=\:\frac{\mathrm{1}}{\mathrm{2}} \\ $$
Answered by mathmax by abdo last updated on 12/Jun/21
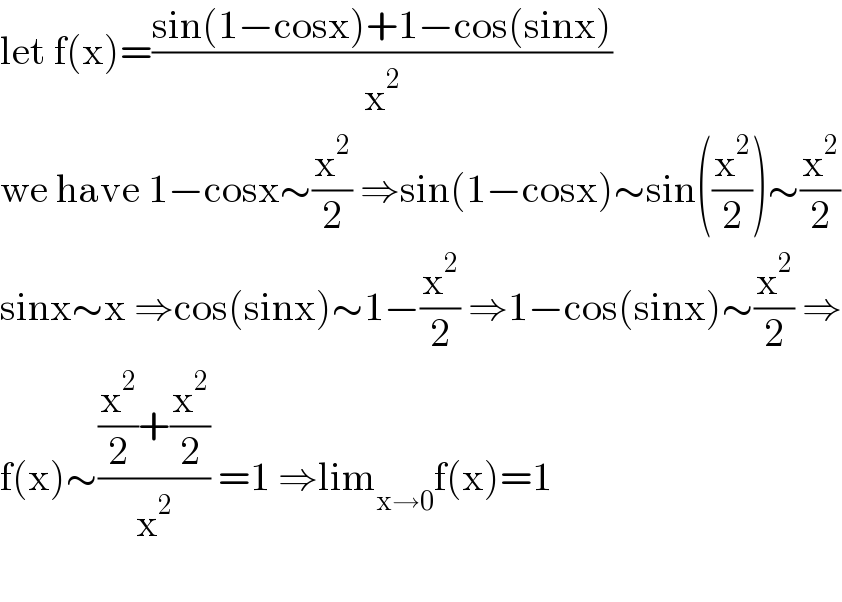
let f(x)=((sin(1−cosx)+1−cos(sinx))/x^2 ) we have 1−cosx∼(x^2 /2) ⇒sin(1−cosx)∼sin((x^2 /2))∼(x^2 /2) sinx∼x ⇒cos(sinx)∼1−(x^2 /2) ⇒1−cos(sinx)∼(x^2 /2) ⇒ f(x)∼(((x^2 /2)+(x^2 /2))/x^2 ) =1 ⇒lim_(x→0) f(x)=1
$$\mathrm{let}\:\mathrm{f}\left(\mathrm{x}\right)=\frac{\mathrm{sin}\left(\mathrm{1}−\mathrm{cosx}\right)+\mathrm{1}−\mathrm{cos}\left(\mathrm{sinx}\right)}{\mathrm{x}^{\mathrm{2}} } \\ $$$$\mathrm{we}\:\mathrm{have}\:\mathrm{1}−\mathrm{cosx}\sim\frac{\mathrm{x}^{\mathrm{2}} }{\mathrm{2}}\:\Rightarrow\mathrm{sin}\left(\mathrm{1}−\mathrm{cosx}\right)\sim\mathrm{sin}\left(\frac{\mathrm{x}^{\mathrm{2}} }{\mathrm{2}}\right)\sim\frac{\mathrm{x}^{\mathrm{2}} }{\mathrm{2}} \\ $$$$\mathrm{sinx}\sim\mathrm{x}\:\Rightarrow\mathrm{cos}\left(\mathrm{sinx}\right)\sim\mathrm{1}−\frac{\mathrm{x}^{\mathrm{2}} }{\mathrm{2}}\:\Rightarrow\mathrm{1}−\mathrm{cos}\left(\mathrm{sinx}\right)\sim\frac{\mathrm{x}^{\mathrm{2}} }{\mathrm{2}}\:\Rightarrow \\ $$$$\mathrm{f}\left(\mathrm{x}\right)\sim\frac{\frac{\mathrm{x}^{\mathrm{2}} }{\mathrm{2}}+\frac{\mathrm{x}^{\mathrm{2}} }{\mathrm{2}}}{\mathrm{x}^{\mathrm{2}} }\:=\mathrm{1}\:\Rightarrow\mathrm{lim}_{\mathrm{x}\rightarrow\mathrm{0}} \mathrm{f}\left(\mathrm{x}\right)=\mathrm{1} \\ $$$$ \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *