Question Number 76077 by vishalbhardwaj last updated on 23/Dec/19

Commented by MJS last updated on 23/Dec/19

Commented by MJS last updated on 23/Dec/19

Commented by vishalbhardwaj last updated on 23/Dec/19
Answered by MJS last updated on 23/Dec/19
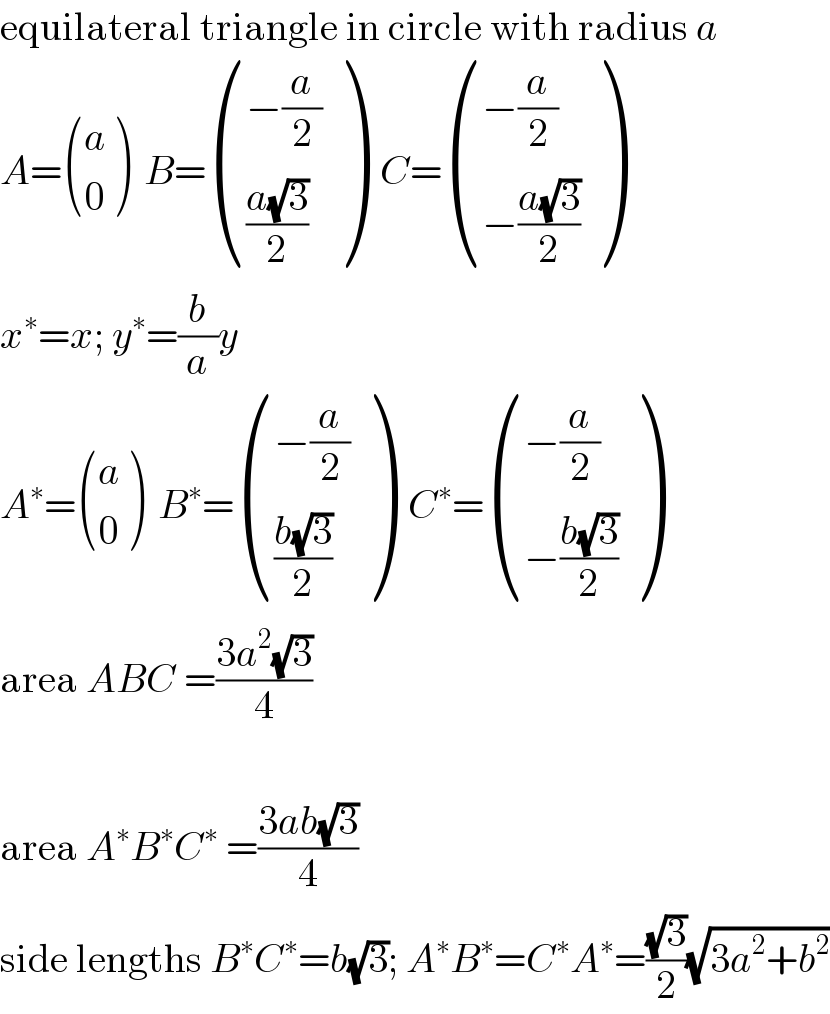
Commented by vishalbhardwaj last updated on 23/Dec/19

Answered by MJS last updated on 23/Dec/19
![A= ((a),(0) ) B= ((x),(((b/a)(√(a^2 −x^2 )))) ) C= ((x),((−(b/a)(√(a^2 −x^2 )))) ) ∣AB∣^2 =∣CA∣^2 =(x−a)(x−((a(a^2 +b^2 ))/(a^2 −b^2 ))) ∣BC∣^2 =((4b^2 )/a^2 )(a^2 −x^2 ) area (o, p, q) = =(1/4)(√((o+p+q)(−o+p+q)(o−p+q)(o+p−q))) =(1/4)(√(2(o^2 p^2 +o^2 q^2 +p^2 q^2 −(o^4 +p^4 +q^4 ))) area (o, p, p) =(1/4)o(√(4p^2 −o^2 )) o^2 =((4b^2 )/a^2 )(a^2 −x^2 )∧p^2 =(x−a)(x−((a(a^2 +b^2 ))/(a^2 −b^2 ))) area (o, p, p) =(b/a)∣x−a∣(√(a^2 −x^2 )) (d/dx)[(b/a)∣x−a∣(√(a^2 −x^2 ))]=±((b(2x^2 −ax−a^2 ))/(a(√(a^2 −x^3 ))))=0 ⇒ x=−(a/2)](https://www.tinkutara.com/question/Q76089.png)
