Question Number 67208 by mr W last updated on 24/Aug/19
![Find the times in a day when the hour′s, minute′s and second′s hand of a clock occupy the same angular position. [old question reposted]](https://www.tinkutara.com/question/Q67208.png)
Commented by Kunal12588 last updated on 24/Aug/19
at 12 am and 12 pm?
Commented by mr W last updated on 24/Aug/19
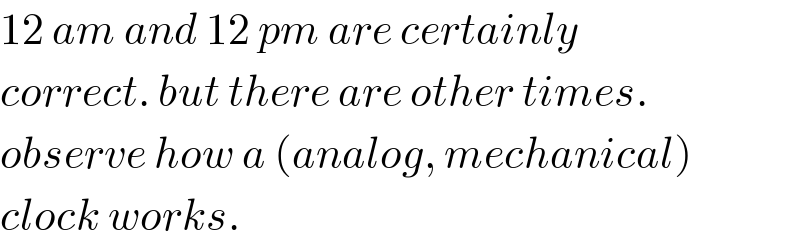
Commented by Tinkutara@ last updated on 24/Aug/19

Commented by Kunal12588 last updated on 24/Aug/19

Commented by mr W last updated on 24/Aug/19
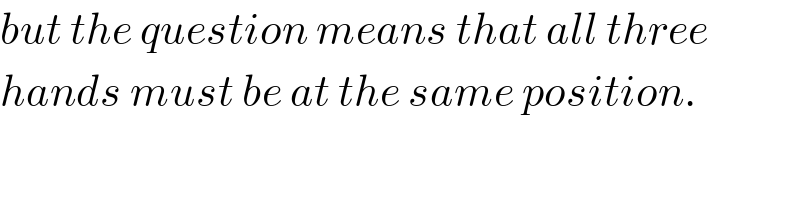
Commented by behi83417@gmail.com last updated on 24/Aug/19
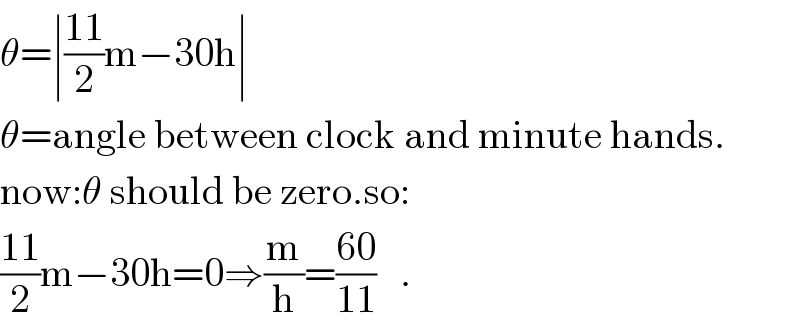
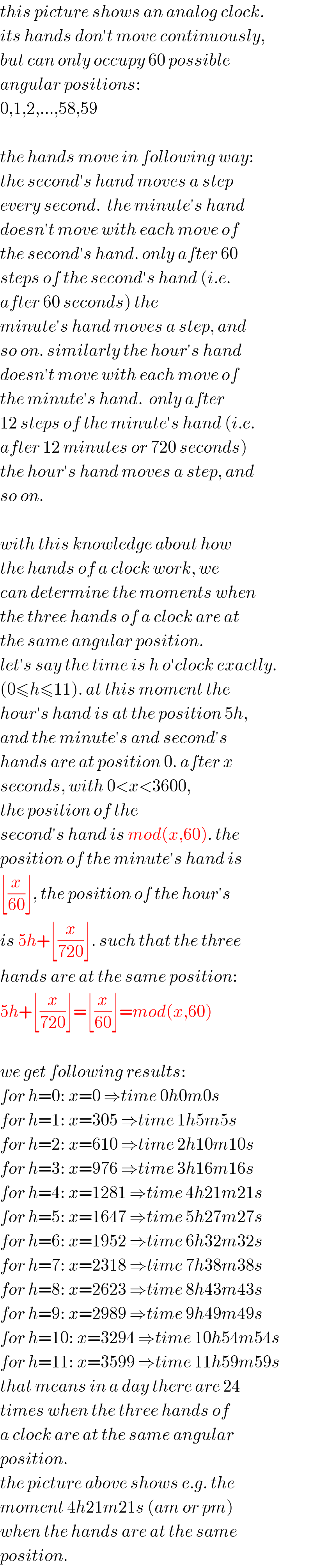
Answered by mr W last updated on 25/Aug/19

Commented by mr W last updated on 25/Aug/19
Commented by JDamian last updated on 25/Aug/19

Commented by mr W last updated on 25/Aug/19

