Menu Close

Find-x-such-that-x-2-5x-5-x-2-21x-10-1-

Tinku Tara 0 Comments
Pin




Question Number 4013 by Yozzii last updated on 26/Dec/15
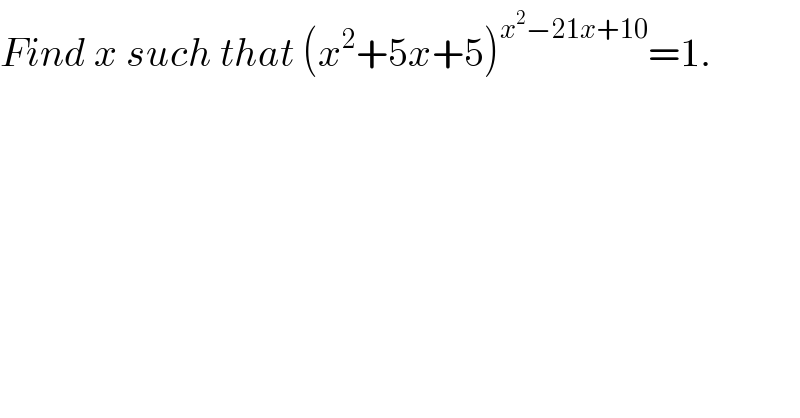
Find x such that (x^2 +5x+5)^(x^2 −21x+10) =1.
$${Find}\:{x}\:{such}\:{that}\:\left({x}^{\mathrm{2}} +\mathrm{5}{x}+\mathrm{5}\right)^{{x}^{\mathrm{2}} −\mathrm{21}{x}+\mathrm{10}} =\mathrm{1}. \\ $$
Commented by 123456 last updated on 26/Dec/15
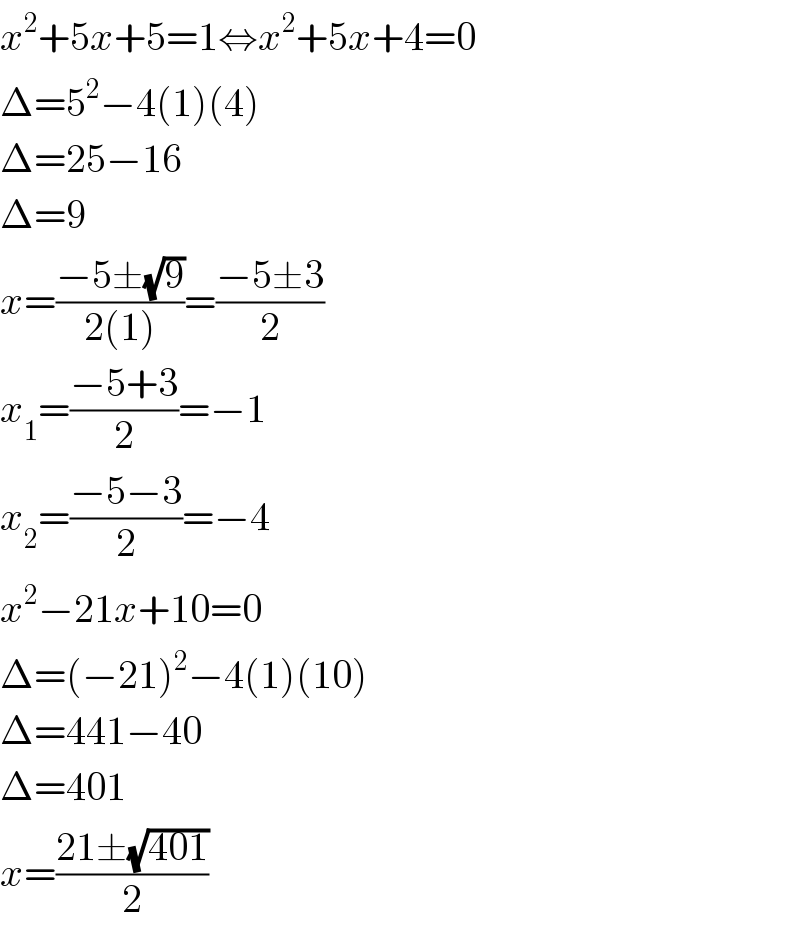
x^2 +5x+5=1⇔x^2 +5x+4=0 Δ=5^2 −4(1)(4) Δ=25−16 Δ=9 x=((−5±(√9))/(2(1)))=((−5±3)/2) x_1 =((−5+3)/2)=−1 x_2 =((−5−3)/2)=−4 x^2 −21x+10=0 Δ=(−21)^2 −4(1)(10) Δ=441−40 Δ=401 x=((21±(√(401)))/2)
$${x}^{\mathrm{2}} +\mathrm{5}{x}+\mathrm{5}=\mathrm{1}\Leftrightarrow{x}^{\mathrm{2}} +\mathrm{5}{x}+\mathrm{4}=\mathrm{0} \\ $$$$\Delta=\mathrm{5}^{\mathrm{2}} −\mathrm{4}\left(\mathrm{1}\right)\left(\mathrm{4}\right) \\ $$$$\Delta=\mathrm{25}−\mathrm{16} \\ $$$$\Delta=\mathrm{9} \\ $$$${x}=\frac{−\mathrm{5}\pm\sqrt{\mathrm{9}}}{\mathrm{2}\left(\mathrm{1}\right)}=\frac{−\mathrm{5}\pm\mathrm{3}}{\mathrm{2}} \\ $$$${x}_{\mathrm{1}} =\frac{−\mathrm{5}+\mathrm{3}}{\mathrm{2}}=−\mathrm{1} \\ $$$${x}_{\mathrm{2}} =\frac{−\mathrm{5}−\mathrm{3}}{\mathrm{2}}=−\mathrm{4} \\ $$$${x}^{\mathrm{2}} −\mathrm{21}{x}+\mathrm{10}=\mathrm{0} \\ $$$$\Delta=\left(−\mathrm{21}\right)^{\mathrm{2}} −\mathrm{4}\left(\mathrm{1}\right)\left(\mathrm{10}\right) \\ $$$$\Delta=\mathrm{441}−\mathrm{40} \\ $$$$\Delta=\mathrm{401} \\ $$$${x}=\frac{\mathrm{21}\pm\sqrt{\mathrm{401}}}{\mathrm{2}} \\ $$
Answered by RasheedSindhi last updated on 26/Dec/15
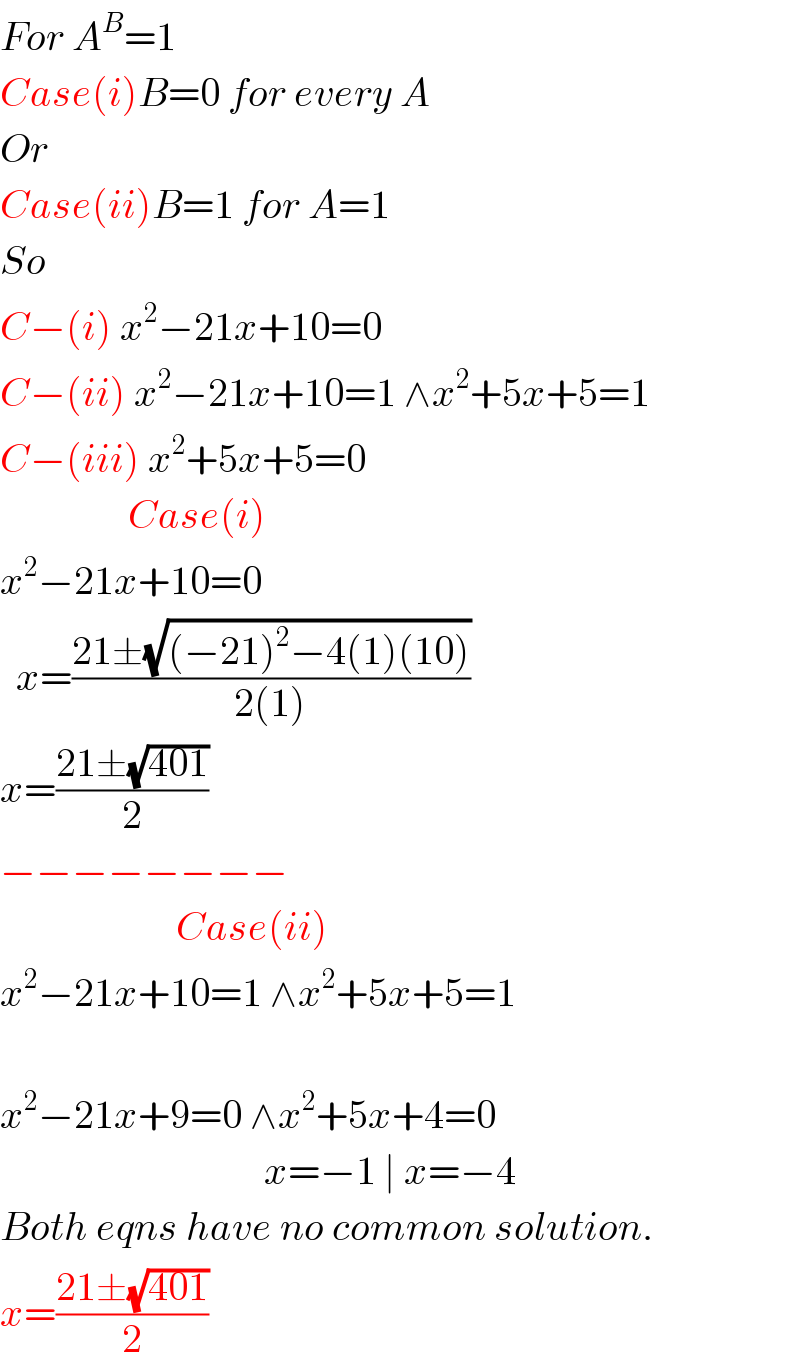
For A^B =1 Case(i)B=0 for every A Or Case(ii)B=1 for A=1 So C−(i) x^2 −21x+10=0 C−(ii) x^2 −21x+10=1 ∧x^2 +5x+5=1 C−(iii) x^2 +5x+5=0 Case(i) x^2 −21x+10=0 x=((21±(√((−21)^2 −4(1)(10))))/(2(1))) x=((21±(√(401)))/2) −−−−−−−− Case(ii) x^2 −21x+10=1 ∧x^2 +5x+5=1 x^2 −21x+9=0 ∧x^2 +5x+4=0 x=−1 ∣ x=−4 Both eqns have no common solution. x=((21±(√(401)))/2)
$${For}\:{A}^{{B}} =\mathrm{1} \\ $$$${Case}\left({i}\right){B}=\mathrm{0}\:{for}\:{every}\:{A} \\ $$$${Or} \\ $$$${Case}\left({ii}\right){B}=\mathrm{1}\:{for}\:{A}=\mathrm{1} \\ $$$${So} \\ $$$${C}−\left({i}\right)\:{x}^{\mathrm{2}} −\mathrm{21}{x}+\mathrm{10}=\mathrm{0} \\ $$$${C}−\left({ii}\right)\:{x}^{\mathrm{2}} −\mathrm{21}{x}+\mathrm{10}=\mathrm{1}\:\wedge{x}^{\mathrm{2}} +\mathrm{5}{x}+\mathrm{5}=\mathrm{1} \\ $$$${C}−\left({iii}\right)\:{x}^{\mathrm{2}} +\mathrm{5}{x}+\mathrm{5}=\mathrm{0}\: \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:{Case}\left({i}\right) \\ $$$${x}^{\mathrm{2}} −\mathrm{21}{x}+\mathrm{10}=\mathrm{0} \\ $$$$\:\:{x}=\frac{\mathrm{21}\pm\sqrt{\left(−\mathrm{21}\right)^{\mathrm{2}} −\mathrm{4}\left(\mathrm{1}\right)\left(\mathrm{10}\right)}}{\mathrm{2}\left(\mathrm{1}\right)} \\ $$$${x}=\frac{\mathrm{21}\pm\sqrt{\mathrm{401}}}{\mathrm{2}} \\ $$$$−−−−−−−− \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:{Case}\left({ii}\right) \\ $$$${x}^{\mathrm{2}} −\mathrm{21}{x}+\mathrm{10}=\mathrm{1}\:\wedge{x}^{\mathrm{2}} +\mathrm{5}{x}+\mathrm{5}=\mathrm{1} \\ $$$$ \\ $$$${x}^{\mathrm{2}} −\mathrm{21}{x}+\mathrm{9}=\mathrm{0}\:\wedge{x}^{\mathrm{2}} +\mathrm{5}{x}+\mathrm{4}=\mathrm{0} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:{x}=−\mathrm{1}\:\mid\:{x}=−\mathrm{4} \\ $$$${Both}\:{eqns}\:{have}\:{no}\:{common}\:{solution}. \\ $$$${x}=\frac{\mathrm{21}\pm\sqrt{\mathrm{401}}}{\mathrm{2}} \\ $$
Commented by Yozzii last updated on 26/Dec/15
True. And my question hadn′t specified real x only. Good!
$${True}.\:{And}\:{my}\:{question}\:{hadn}'{t}\:{specified} \\ $$$${real}\:{x}\:{only}.\:{Good}! \\ $$
Commented by Rasheed Soomro last updated on 26/Dec/15
If we consider complex numbers many cases can be considered (however it is not necessary that given equations support all these cases.) For A=ω (Cuberoot of unity) B may be 3k type number. A=i B may be doubly even numbers ETC.
$${If}\:{we}\:{consider}\:{complex}\:{numbers} \\ $$$${many}\:{cases}\:{can}\:{be}\:{considered}\: \\ $$$$\left({however}\:{it}\:{is}\:{not}\:{necessary}\:{that}\right. \\ $$$${given}\:{equations}\:{support}\:{all}\:{these} \\ $$$$\left.{cases}.\right) \\ $$$${For}\:{A}=\omega\:\left({Cuberoot}\:{of}\:{unity}\right)\:{B} \\ $$$${may}\:{be}\:\mathrm{3}{k}\:{type}\:{number}. \\ $$$${A}={i}\:{B}\:{may}\:{be}\:{doubly}\:{even}\:{numbers} \\ $$$${ETC}. \\ $$
Commented by Yozzii last updated on 26/Dec/15
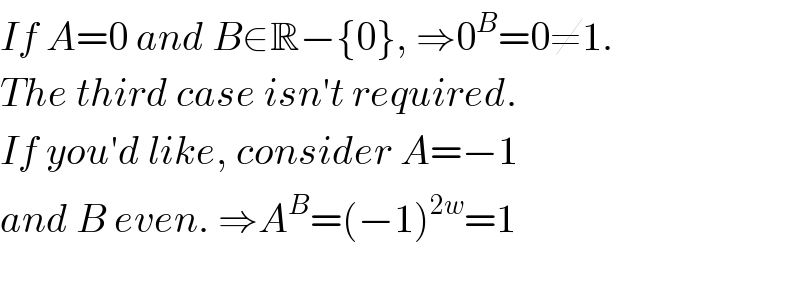
If A=0 and B∈R−{0}, ⇒0^B =0≠1. The third case isn′t required. If you′d like, consider A=−1 and B even. ⇒A^B =(−1)^(2w) =1
$${If}\:{A}=\mathrm{0}\:{and}\:{B}\in\mathbb{R}−\left\{\mathrm{0}\right\},\:\Rightarrow\mathrm{0}^{{B}} =\mathrm{0}\neq\mathrm{1}. \\ $$$${The}\:{third}\:{case}\:{isn}'{t}\:{required}. \\ $$$${If}\:{you}'{d}\:{like},\:{consider}\:{A}=−\mathrm{1}\: \\ $$$${and}\:{B}\:{even}.\:\Rightarrow{A}^{{B}} =\left(−\mathrm{1}\right)^{\mathrm{2}{w}} =\mathrm{1} \\ $$$$ \\ $$
Commented by Yozzii last updated on 26/Dec/15
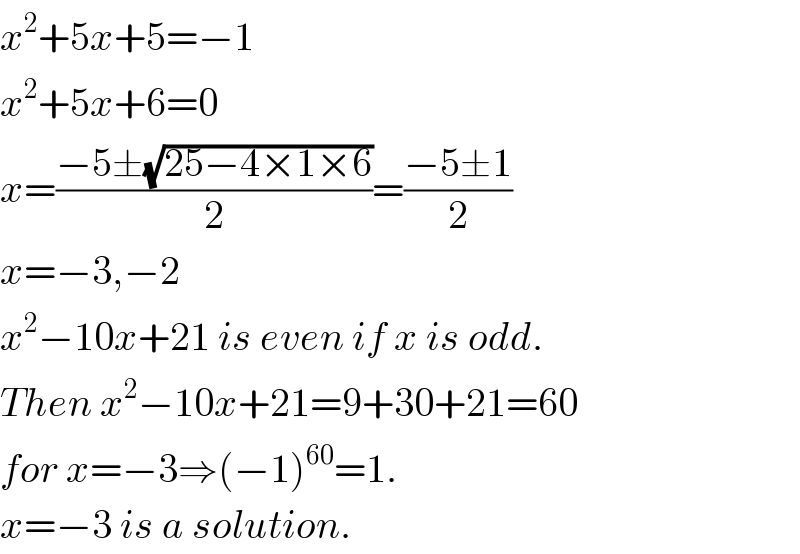
x^2 +5x+5=−1 x^2 +5x+6=0 x=((−5±(√(25−4×1×6)))/2)=((−5±1)/2) x=−3,−2 x^2 −10x+21 is even if x is odd. Then x^2 −10x+21=9+30+21=60 for x=−3⇒(−1)^(60) =1. x=−3 is a solution.
$${x}^{\mathrm{2}} +\mathrm{5}{x}+\mathrm{5}=−\mathrm{1} \\ $$$${x}^{\mathrm{2}} +\mathrm{5}{x}+\mathrm{6}=\mathrm{0} \\ $$$${x}=\frac{−\mathrm{5}\pm\sqrt{\mathrm{25}−\mathrm{4}×\mathrm{1}×\mathrm{6}}}{\mathrm{2}}=\frac{−\mathrm{5}\pm\mathrm{1}}{\mathrm{2}} \\ $$$${x}=−\mathrm{3},−\mathrm{2}\: \\ $$$${x}^{\mathrm{2}} −\mathrm{10}{x}+\mathrm{21}\:{is}\:{even}\:{if}\:{x}\:{is}\:{odd}. \\ $$$${Then}\:{x}^{\mathrm{2}} −\mathrm{10}{x}+\mathrm{21}=\mathrm{9}+\mathrm{30}+\mathrm{21}=\mathrm{60} \\ $$$${for}\:{x}=−\mathrm{3}\Rightarrow\left(−\mathrm{1}\right)^{\mathrm{60}} =\mathrm{1}. \\ $$$${x}=−\mathrm{3}\:{is}\:{a}\:{solution}. \\ $$
Commented by Yozzii last updated on 26/Dec/15
The case by case approach is ideal and systematic! We just need to ensure all cases are considered...
$${The}\:{case}\:{by}\:{case}\:{approach}\:{is}\:{ideal} \\ $$$${and}\:{systematic}! \\ $$$${We}\:{just}\:{need}\:{to}\:{ensure}\:{all}\:{cases}\:{are} \\ $$$${considered}… \\ $$
Commented by RasheedSindhi last updated on 26/Dec/15
THankS A LoT! YourGuidanceIsAlways Welcomed! 3rd Case is my mistake! I am going to correctit! Actually due to hastiness I have made many mistakes in this answer. A=−1 and B ∈ E is a good suggestion,which was not in my mind!
$$\boldsymbol{\mathcal{T}}\mathcal{H}{ank}\mathcal{S}\:\mathcal{A}\:\mathcal{L}{o}\boldsymbol{\mathcal{T}}! \\ $$$$\boldsymbol{\mathrm{YourGuidanceIsAlways}} \\ $$$$\boldsymbol{\mathrm{Welcomed}}! \\ $$$$\mathrm{3}{rd}\:{Case}\:{is}\:{my}\:{mistake}! \\ $$$${I}\:{am}\:{going}\:{to}\:{correctit}! \\ $$$${Actually}\:{due}\:{to}\:{hastiness} \\ $$$${I}\:{have}\:{made}\:{many}\:{mistakes} \\ $$$${in}\:{this}\:{answer}. \\ $$$${A}=−\mathrm{1}\:{and}\:{B}\:\in\:\mathbb{E}\:{is}\:{a}\:{good} \\ $$$${suggestion},{which}\:{was}\:{not}\:{in} \\ $$$${my}\:{mind}! \\ $$
Answered by Alejandro Prieto last updated on 26/Dec/15
Si (x^2 + 5x + 5)^(x^2 − 21x + 10) = 1 (x^2 − 21x + 10)ln(x^2 + 5x + 5) = 0 si ln(x^2 + 5x + 5) = 0 , x^2 + 5x + 5 = 1 entonces x^2 + 5x + 4 = (x + 4)(x + 1) = 0 luego x_1 = −1 x_2 = −4 Si x^2 − 21x + 10 = 0 x_3 = ((21 − (√(401)))/2) x_4 = ((21 + (√(401)))/2)
$$\mathrm{Si}\:\left({x}^{\mathrm{2}} \:+\:\mathrm{5}{x}\:+\:\mathrm{5}\right)^{{x}^{\mathrm{2}} \:−\:\mathrm{21}{x}\:+\:\mathrm{10}} \:=\:\mathrm{1} \\ $$$$\left({x}^{\mathrm{2}} \:−\:\mathrm{21}{x}\:+\:\mathrm{10}\right){ln}\left({x}^{\mathrm{2}} \:+\:\mathrm{5}{x}\:+\:\mathrm{5}\right)\:=\:\mathrm{0} \\ $$$$\mathrm{si}\:{ln}\left({x}^{\mathrm{2}} \:+\:\mathrm{5}{x}\:+\:\mathrm{5}\right)\:=\:\mathrm{0}\:,\:\:\:\:\:\:{x}^{\mathrm{2}} \:+\:\mathrm{5}{x}\:+\:\mathrm{5}\:=\:\mathrm{1} \\ $$$$\mathrm{entonces}\:{x}^{\mathrm{2}} \:+\:\mathrm{5}{x}\:+\:\mathrm{4}\:=\:\left({x}\:+\:\mathrm{4}\right)\left({x}\:+\:\mathrm{1}\right)\:=\:\mathrm{0} \\ $$$$\mathrm{luego}\:{x}_{\mathrm{1}} \:=\:−\mathrm{1} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:{x}_{\mathrm{2}} \:=\:−\mathrm{4} \\ $$$$\mathrm{Si}\:{x}^{\mathrm{2}} \:−\:\mathrm{21}{x}\:+\:\mathrm{10}\:=\:\mathrm{0} \\ $$$${x}_{\mathrm{3}} \:=\:\frac{\mathrm{21}\:−\:\sqrt{\mathrm{401}}}{\mathrm{2}} \\ $$$${x}_{\mathrm{4}} \:=\:\frac{\mathrm{21}\:+\:\sqrt{\mathrm{401}}}{\mathrm{2}} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *