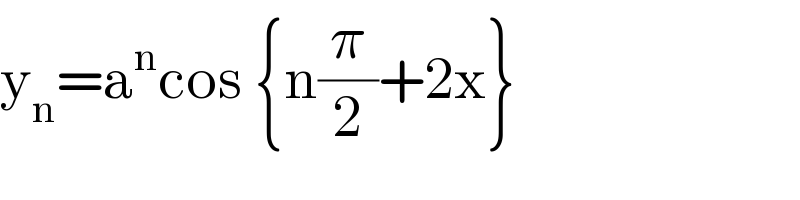Question Number 8488 by Basant007 last updated on 12/Oct/16
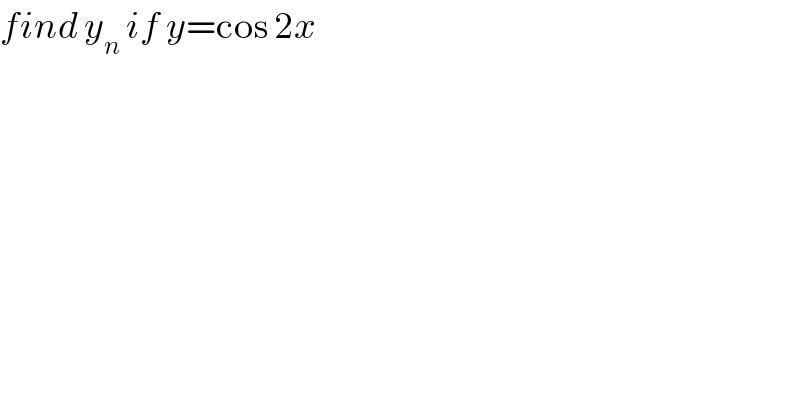
Commented by FilupSmith last updated on 13/Oct/16
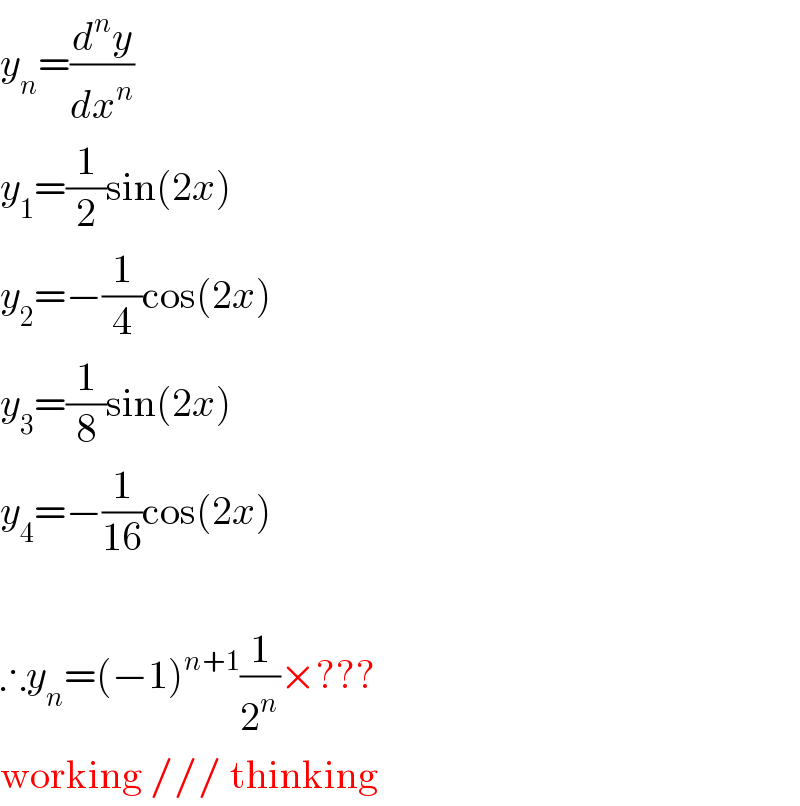
Commented by Rasheed Soomro last updated on 13/Oct/16
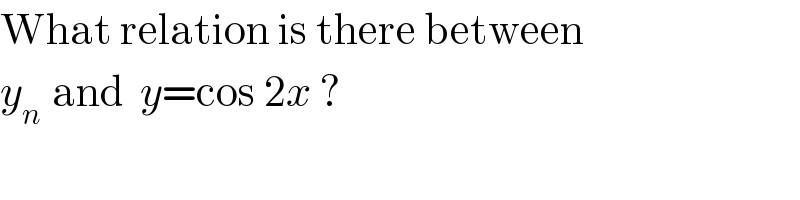
Commented by Basant007 last updated on 13/Oct/16
Commented by Basant007 last updated on 13/Oct/16

Commented by FilupSmith last updated on 13/Oct/16
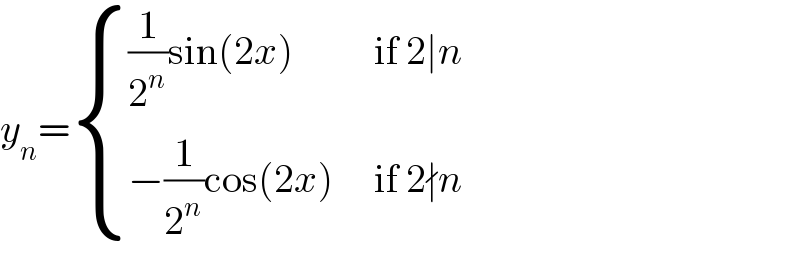
Commented by Basant007 last updated on 13/Oct/16

Commented by Basant007 last updated on 13/Oct/16