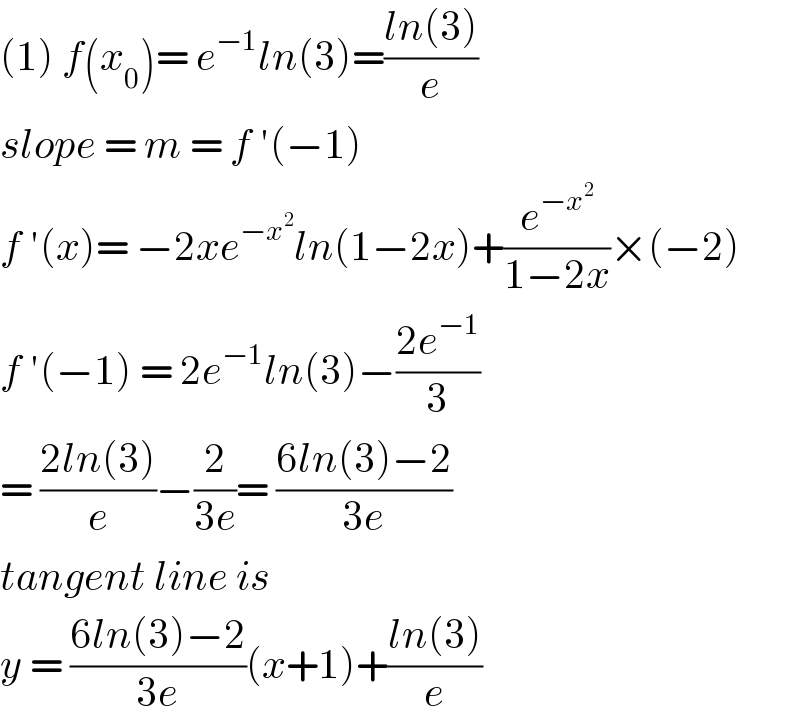Question Number 78136 by msup trace by abdo last updated on 14/Jan/20
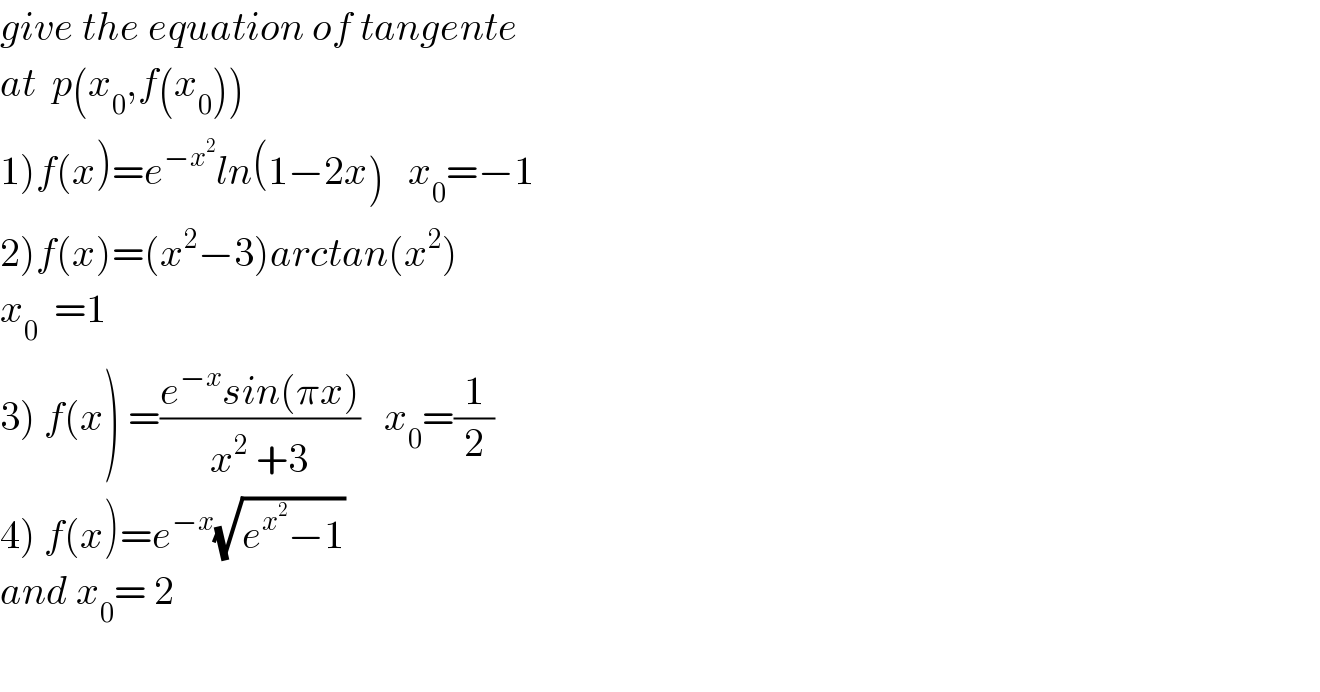
Commented by mathmax by abdo last updated on 14/Jan/20
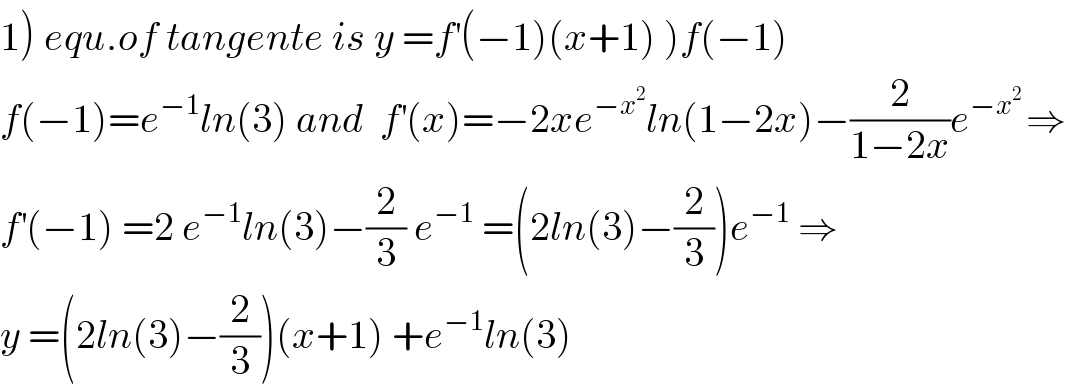
Commented by mathmax by abdo last updated on 14/Jan/20
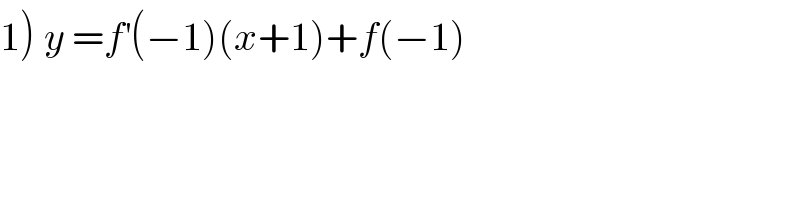
Commented by jagoll last updated on 14/Jan/20
Commented by msup trace by abdo last updated on 15/Jan/20
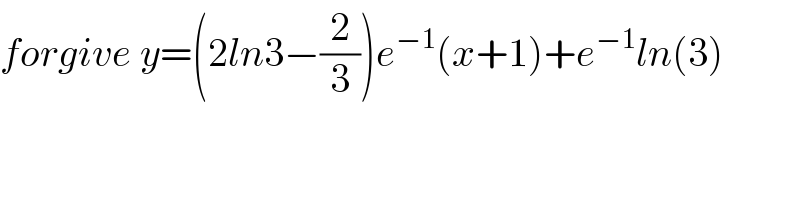
Commented by msup trace by abdo last updated on 15/Jan/20
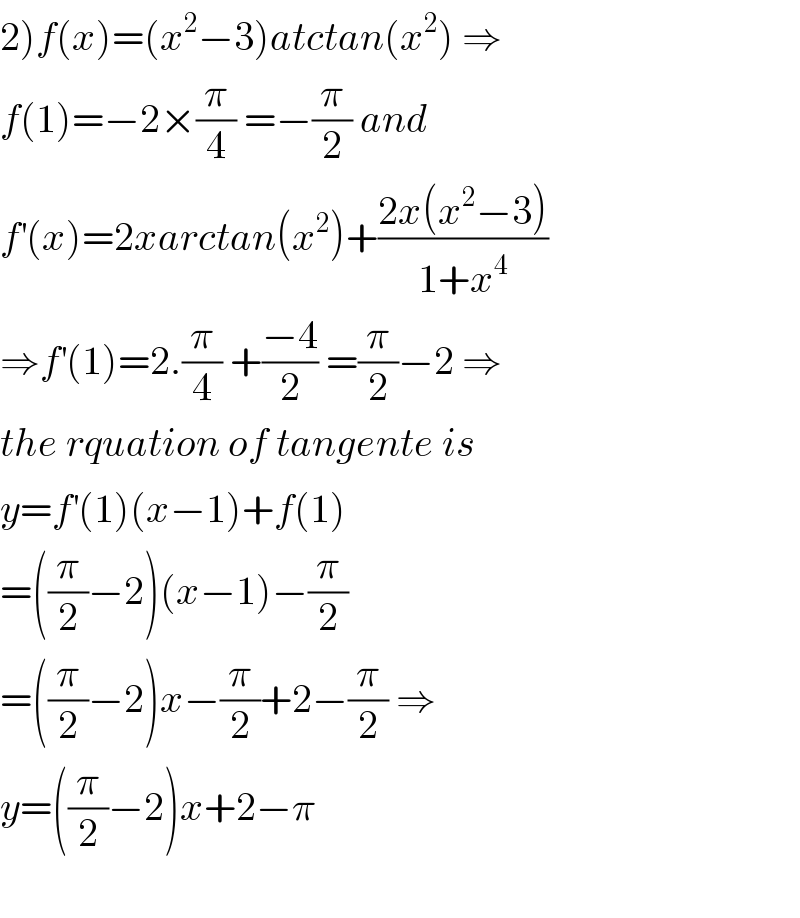
Answered by jagoll last updated on 14/Jan/20