Question Number 134069 by john_santu last updated on 27/Feb/21
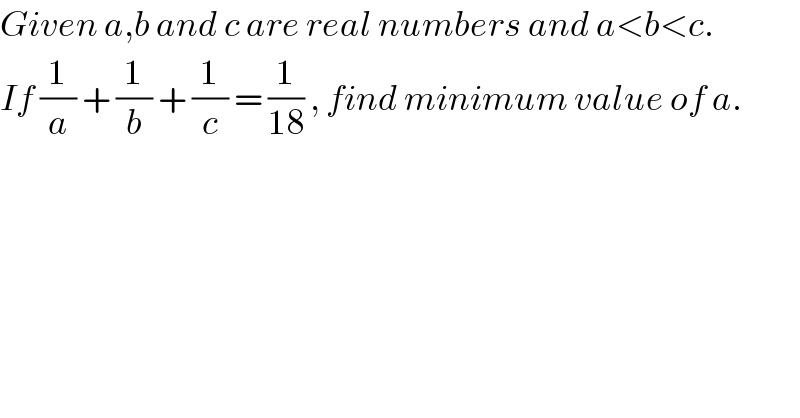
Commented by mr W last updated on 27/Feb/21
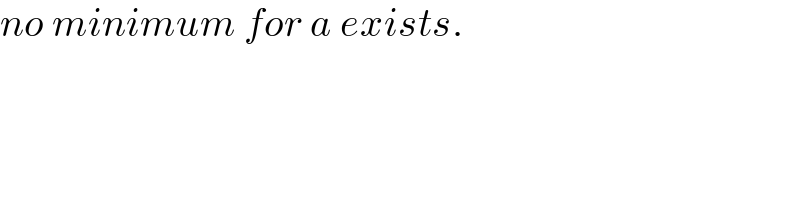
Commented by mr W last updated on 27/Feb/21

Answered by bramlexs22 last updated on 27/Feb/21
![AM−GM (((1/a)+(1/b)+(1/c))/(3 )) ≥ ((1/(abc)))^(1/3) (([ (1/(18)) ])/3) ≥ ((1/(abc)))^(1/3) ⇒ (1/(54)) ≥ ((1/(abc)))^(1/3) (1/(54^3 )) ≥ (1/(abc)) , it hold for a=b=c we get a = 54](https://www.tinkutara.com/question/Q134073.png)
Commented by mr W last updated on 27/Feb/21

