Question Number 136167 by ZiYangLee last updated on 19/Mar/21
$$\mathrm{Given}\:\mathrm{that}\:\mathrm{one}\:\mathrm{of}\:\mathrm{the}\:\mathrm{value}\:\mathrm{for}\:\mathrm{the}\:\mathrm{5}^{\mathrm{th}} \\ $$$$\mathrm{root}\:\mathrm{of}\:\mathrm{a}\:\mathrm{complex}\:\mathrm{number}\:\mathrm{is}\:−\mathrm{1}+{i}. \\ $$$$\mathrm{Find}\:\mathrm{the}\:\mathrm{another}\:\mathrm{four}\:\mathrm{values}. \\ $$
Answered by mr W last updated on 19/Mar/21
![one 5^(th) root is −1+i=(√2)(cos ((3π)/4)+i sin ((3π)/4)) ((3π)/4)+((2π)/5)=((23π)/(20)) ((3π)/4)+((2π)/5)+((2π)/5)=((31π)/(20)) ((3π)/4)+((2π)/5)+((2π)/5)+((2π)/5)=((39π)/(20)) ((3π)/4)−((2π)/5)=((7π)/(20)) the other 5^(th) roots are: (√2)[cos (((7π)/(20)))+i sin (((7π)/(20)))] (√2)[cos (((23π)/(20)))+i sin (((23π)/(20)))] (√2)[cos (((31π)/(20)))+i sin (((31π)/(20)))] (√2)[cos (((39π)/(20)))+i sin (((39π)/(20)))]](https://www.tinkutara.com/question/Q136182.png)
$${one}\:\mathrm{5}^{{th}} \:{root}\:{is}\:−\mathrm{1}+{i}=\sqrt{\mathrm{2}}\left(\mathrm{cos}\:\frac{\mathrm{3}\pi}{\mathrm{4}}+{i}\:\mathrm{sin}\:\frac{\mathrm{3}\pi}{\mathrm{4}}\right) \\ $$$$\frac{\mathrm{3}\pi}{\mathrm{4}}+\frac{\mathrm{2}\pi}{\mathrm{5}}=\frac{\mathrm{23}\pi}{\mathrm{20}} \\ $$$$\frac{\mathrm{3}\pi}{\mathrm{4}}+\frac{\mathrm{2}\pi}{\mathrm{5}}+\frac{\mathrm{2}\pi}{\mathrm{5}}=\frac{\mathrm{31}\pi}{\mathrm{20}} \\ $$$$\frac{\mathrm{3}\pi}{\mathrm{4}}+\frac{\mathrm{2}\pi}{\mathrm{5}}+\frac{\mathrm{2}\pi}{\mathrm{5}}+\frac{\mathrm{2}\pi}{\mathrm{5}}=\frac{\mathrm{39}\pi}{\mathrm{20}} \\ $$$$\frac{\mathrm{3}\pi}{\mathrm{4}}−\frac{\mathrm{2}\pi}{\mathrm{5}}=\frac{\mathrm{7}\pi}{\mathrm{20}} \\ $$$${the}\:{other}\:\mathrm{5}^{{th}} \:{roots}\:{are}: \\ $$$$\sqrt{\mathrm{2}}\left[\mathrm{cos}\:\left(\frac{\mathrm{7}\pi}{\mathrm{20}}\right)+{i}\:\mathrm{sin}\:\left(\frac{\mathrm{7}\pi}{\mathrm{20}}\right)\right] \\ $$$$\sqrt{\mathrm{2}}\left[\mathrm{cos}\:\left(\frac{\mathrm{23}\pi}{\mathrm{20}}\right)+{i}\:\mathrm{sin}\:\left(\frac{\mathrm{23}\pi}{\mathrm{20}}\right)\right] \\ $$$$\sqrt{\mathrm{2}}\left[\mathrm{cos}\:\left(\frac{\mathrm{31}\pi}{\mathrm{20}}\right)+{i}\:\mathrm{sin}\:\left(\frac{\mathrm{31}\pi}{\mathrm{20}}\right)\right] \\ $$$$\sqrt{\mathrm{2}}\left[\mathrm{cos}\:\left(\frac{\mathrm{39}\pi}{\mathrm{20}}\right)+{i}\:\mathrm{sin}\:\left(\frac{\mathrm{39}\pi}{\mathrm{20}}\right)\right] \\ $$
Commented by mr W last updated on 19/Mar/21
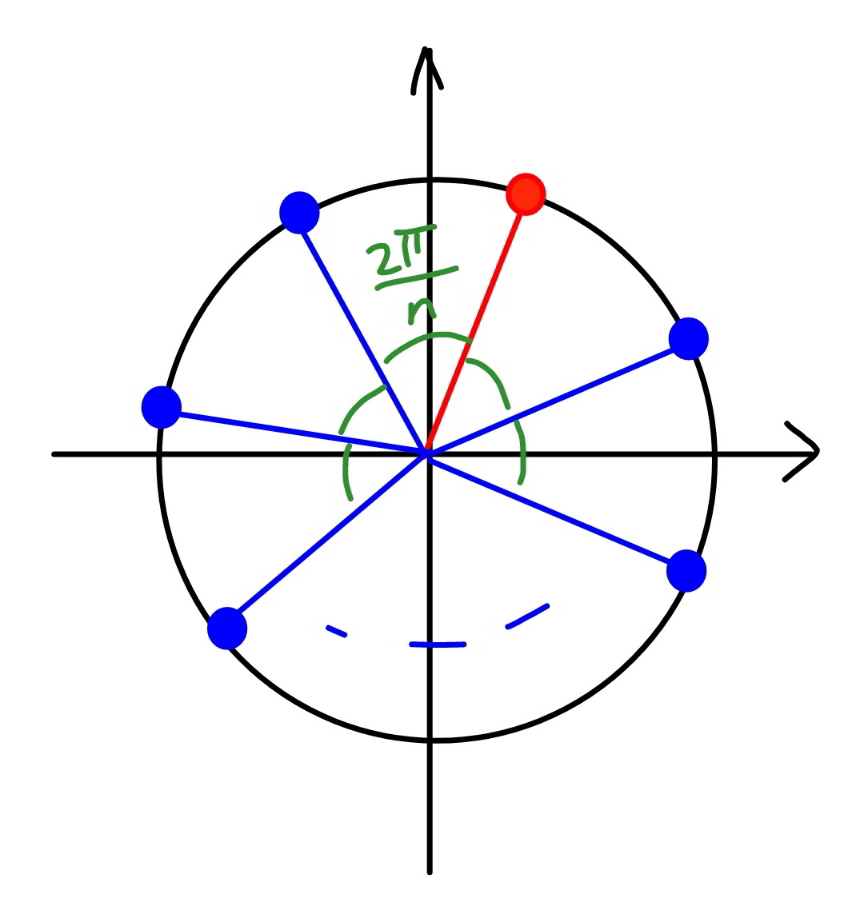
Commented by mr W last updated on 20/Mar/21
$$\blacksquare\:{a}\:{known}\:{n}^{{th}} \:{root}\:{of}\:{z} \\ $$$$\blacksquare\:{the}\:{other}\:{n}^{{th}} \:{roots}\:{of}\:{z} \\ $$$${if}\:{one}\:{of}\:{the}\:{n}^{{th}} \:{roots}\:{of}\:{a}\:{number}\:{is} \\ $$$${re}^{\alpha{i}} ,\:{then}\:{the}\:{other}\:{n}^{{th}} \:{roots}\:{of}\:{it}\:{are} \\ $$$${re}^{\left(\alpha+\frac{\mathrm{2}{k}\pi}{{n}}\right){i}} \:{with}\:{k}=\mathrm{1},\mathrm{2},…,{n}−\mathrm{1} \\ $$
Commented by ZiYangLee last updated on 20/Mar/21
$$\mathrm{Thanks}\:\mathrm{Sir}! \\ $$