Question Number 4918 by FilupSmith last updated on 21/Mar/16

$$\mathrm{Hey}\:\mathrm{guys}!\:{A}\:\mathrm{new}\:\mathrm{graphing}\:\mathrm{app}\:\mathrm{is}\:\mathrm{out} \\ $$$$\mathrm{called}\:{Desmos} \\ $$$$ \\ $$$$\mathrm{It}\:\mathrm{is}\:\mathrm{really}\:\mathrm{amazing}! \\ $$$$ \\ $$$$\mathrm{Just}\:\mathrm{thought}\:\mathrm{I}'{d}\:\mathrm{let}\:\mathrm{you}\:\mathrm{know}! \\ $$
Commented by Yozzii last updated on 21/Mar/16
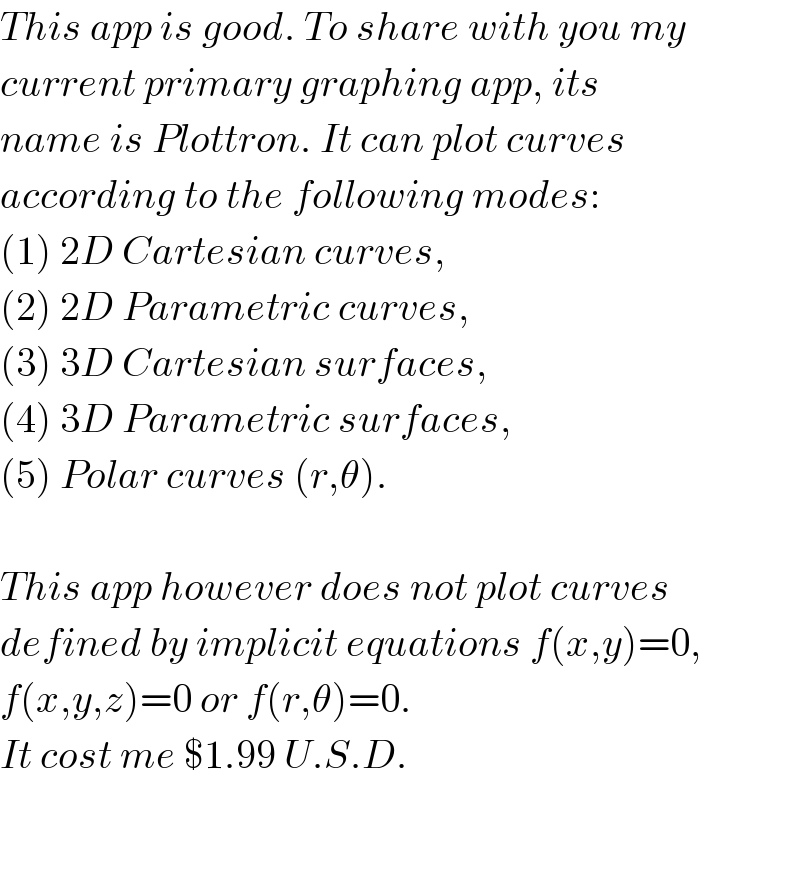
$${This}\:{app}\:{is}\:{good}.\:{To}\:{share}\:{with}\:{you}\:{my} \\ $$$${current}\:{primary}\:{graphing}\:{app},\:{its} \\ $$$${name}\:{is}\:{Plottron}.\:{It}\:{can}\:{plot}\:{curves} \\ $$$${according}\:{to}\:{the}\:{following}\:{modes}: \\ $$$$\left(\mathrm{1}\right)\:\mathrm{2}{D}\:{Cartesian}\:{curves}, \\ $$$$\left(\mathrm{2}\right)\:\mathrm{2}{D}\:{Parametric}\:{curves}, \\ $$$$\left(\mathrm{3}\right)\:\mathrm{3}{D}\:{Cartesian}\:{surfaces}, \\ $$$$\left(\mathrm{4}\right)\:\mathrm{3}{D}\:{Parametric}\:{surfaces}, \\ $$$$\left(\mathrm{5}\right)\:{Polar}\:{curves}\:\left({r},\theta\right). \\ $$$$ \\ $$$${This}\:{app}\:{however}\:{does}\:{not}\:{plot}\:{curves} \\ $$$${defined}\:{by}\:{implicit}\:{equations}\:{f}\left({x},{y}\right)=\mathrm{0}, \\ $$$${f}\left({x},{y},{z}\right)=\mathrm{0}\:{or}\:{f}\left({r},\theta\right)=\mathrm{0}.\: \\ $$$${It}\:{cost}\:{me}\:\$\mathrm{1}.\mathrm{99}\:{U}.{S}.{D}. \\ $$$$ \\ $$$$ \\ $$
