Question Number 6316 by sanusihammed last updated on 23/Jun/16
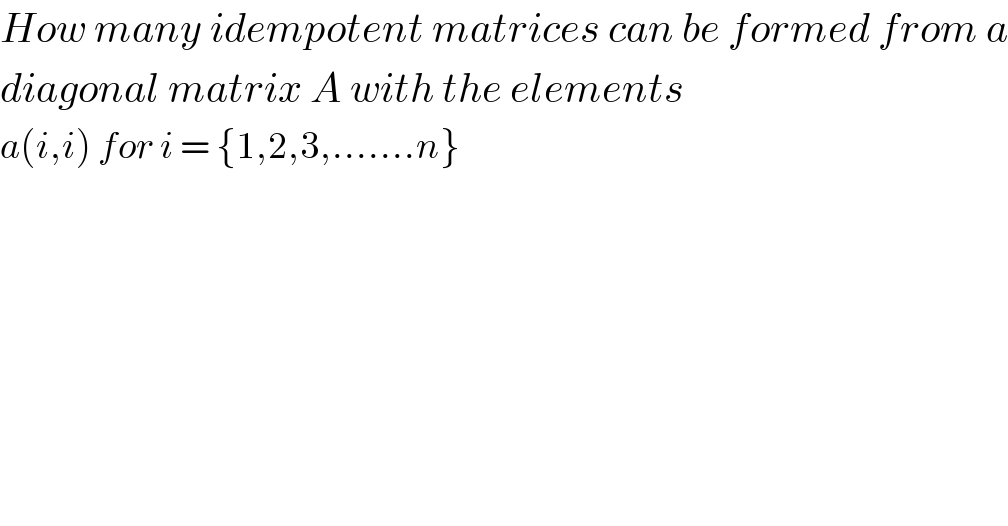
$${How}\:{many}\:{idempotent}\:{matrices}\:{can}\:{be}\:{formed}\:{from}\:{a} \\ $$$${diagonal}\:{matrix}\:{A}\:{with}\:{the}\:{elements}\: \\ $$$${a}\left({i},{i}\right)\:{for}\:{i}\:=\:\left\{\mathrm{1},\mathrm{2},\mathrm{3},…….{n}\right\} \\ $$
Answered by nburiburu last updated on 23/Jun/16
![in R^(n×n) : A idempotent then A^2 =A×A=A if A=D diagonal, then A^2 =[d_(ii) ×d_(ii) ]=[d_(ii) ^2 ]=[d_(ii) ] ∀i then d_(ii) ^2 =d_(ii) => d_(ii) =1 or 0 ∀i Now it has n elements and could be only 2 numbers on each place. So, there are 2^n possibles A.](https://www.tinkutara.com/question/Q6322.png)
$$\:{in}\:\mathbb{R}^{{n}×{n}} :\: \\ $$$${A}\:{idempotent}\:{then}\:{A}^{\mathrm{2}} ={A}×{A}={A} \\ $$$${if}\:{A}={D}\:{diagonal},\:{then} \\ $$$${A}^{\mathrm{2}} =\left[{d}_{{ii}} ×{d}_{{ii}} \right]=\left[{d}_{{ii}} ^{\mathrm{2}} \right]=\left[{d}_{{ii}} \right]\:\forall{i} \\ $$$${then}\:{d}_{{ii}} ^{\mathrm{2}} ={d}_{{ii}} \:=>\:{d}_{{ii}} =\mathrm{1}\:{or}\:\mathrm{0}\:\forall{i} \\ $$$${Now}\:{it}\:{has}\:{n}\:{elements}\:{and}\:{could}\:{be}\:{only}\:\mathrm{2}\:{numbers}\:{on}\:{each}\:{place}. \\ $$$${So},\:{there}\:{are}\:\mathrm{2}^{{n}} \:{possibles}\:{A}. \\ $$$$ \\ $$
