Menu Close

I-dx-a-2-x-1-x-

Tinku Tara 0 Comments
Pin




Question Number 142457 by ajfour last updated on 01/Jun/21
I=∫(dx/( (√(a^2 −(x+(1/x))))))
$$\:{I}=\int\frac{{dx}}{\:\sqrt{{a}^{\mathrm{2}} −\left({x}+\frac{\mathrm{1}}{{x}}\right)}} \\ $$
Answered by MJS_new last updated on 01/Jun/21
I′m afraid you′re ending on elliptic functions again. my try: ∫(dx/( (√(a^2 −(x+(1/x))))))= [t=x+(1/x) → dx=((t+(√(t^2 −4)))/( (√(t^2 −4))))] =(1/2)∫((t+(√(t^2 −4)))/( (√(t^2 −4))(√(a^2 −t))))dt= =(1/2)∫(dt/( (√(a^2 −t))))+(1/2)∫(t/( (√(t^2 −4))(√(a^2 −t))))dt= =−(√(a^2 −t))+(1/2)∫(t/( (√(t^2 −4))(√(a^2 −t))))dt (1/2)∫(t/( (√(t^2 −4))(√(a^2 −t))))dt= [u=((√(t^2 −4))/( (√(a^2 −t)))) → dt=−((2(√(t^2 −4))(√((a^2 −t)^3 )))/(t^2 −2a^2 t+4))] =(1/2)∫du−(1/2)∫(u^2 /( (√(u^4 +4a^2 u^2 +16))))du= =(u/2)−(1/2)∫(u^2 /( (√(u^4 +4a^2 u^2 +16))))du and we cannot solve the remaining integral using elementary calculus
$$\mathrm{I}'\mathrm{m}\:\mathrm{afraid}\:\mathrm{you}'\mathrm{re}\:\mathrm{ending}\:\mathrm{on}\:\mathrm{elliptic}\:\mathrm{functions} \\ $$$$\mathrm{again}. \\ $$$$\mathrm{my}\:\mathrm{try}: \\ $$$$\int\frac{{dx}}{\:\sqrt{{a}^{\mathrm{2}} −\left({x}+\frac{\mathrm{1}}{{x}}\right)}}= \\ $$$$\:\:\:\:\:\left[{t}={x}+\frac{\mathrm{1}}{{x}}\:\rightarrow\:{dx}=\frac{{t}+\sqrt{{t}^{\mathrm{2}} −\mathrm{4}}}{\:\sqrt{{t}^{\mathrm{2}} −\mathrm{4}}}\right] \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\int\frac{{t}+\sqrt{{t}^{\mathrm{2}} −\mathrm{4}}}{\:\sqrt{{t}^{\mathrm{2}} −\mathrm{4}}\sqrt{{a}^{\mathrm{2}} −{t}}}{dt}= \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\int\frac{{dt}}{\:\sqrt{{a}^{\mathrm{2}} −{t}}}+\frac{\mathrm{1}}{\mathrm{2}}\int\frac{{t}}{\:\sqrt{{t}^{\mathrm{2}} −\mathrm{4}}\sqrt{{a}^{\mathrm{2}} −{t}}}{dt}= \\ $$$$=−\sqrt{{a}^{\mathrm{2}} −{t}}+\frac{\mathrm{1}}{\mathrm{2}}\int\frac{{t}}{\:\sqrt{{t}^{\mathrm{2}} −\mathrm{4}}\sqrt{{a}^{\mathrm{2}} −{t}}}{dt} \\ $$$$\frac{\mathrm{1}}{\mathrm{2}}\int\frac{{t}}{\:\sqrt{{t}^{\mathrm{2}} −\mathrm{4}}\sqrt{{a}^{\mathrm{2}} −{t}}}{dt}= \\ $$$$\:\:\:\:\:\left[{u}=\frac{\sqrt{{t}^{\mathrm{2}} −\mathrm{4}}}{\:\sqrt{{a}^{\mathrm{2}} −{t}}}\:\rightarrow\:{dt}=−\frac{\mathrm{2}\sqrt{{t}^{\mathrm{2}} −\mathrm{4}}\sqrt{\left({a}^{\mathrm{2}} −{t}\right)^{\mathrm{3}} }}{{t}^{\mathrm{2}} −\mathrm{2}{a}^{\mathrm{2}} {t}+\mathrm{4}}\right] \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\int{du}−\frac{\mathrm{1}}{\mathrm{2}}\int\frac{{u}^{\mathrm{2}} }{\:\sqrt{{u}^{\mathrm{4}} +\mathrm{4}{a}^{\mathrm{2}} {u}^{\mathrm{2}} +\mathrm{16}}}{du}= \\ $$$$=\frac{{u}}{\mathrm{2}}−\frac{\mathrm{1}}{\mathrm{2}}\int\frac{{u}^{\mathrm{2}} }{\:\sqrt{{u}^{\mathrm{4}} +\mathrm{4}{a}^{\mathrm{2}} {u}^{\mathrm{2}} +\mathrm{16}}}{du} \\ $$$$\mathrm{and}\:\mathrm{we}\:\mathrm{cannot}\:\mathrm{solve}\:\mathrm{the}\:\mathrm{remaining}\:\mathrm{integral} \\ $$$$\mathrm{using}\:\mathrm{elementary}\:\mathrm{calculus} \\ $$
Commented by ajfour last updated on 01/Jun/21
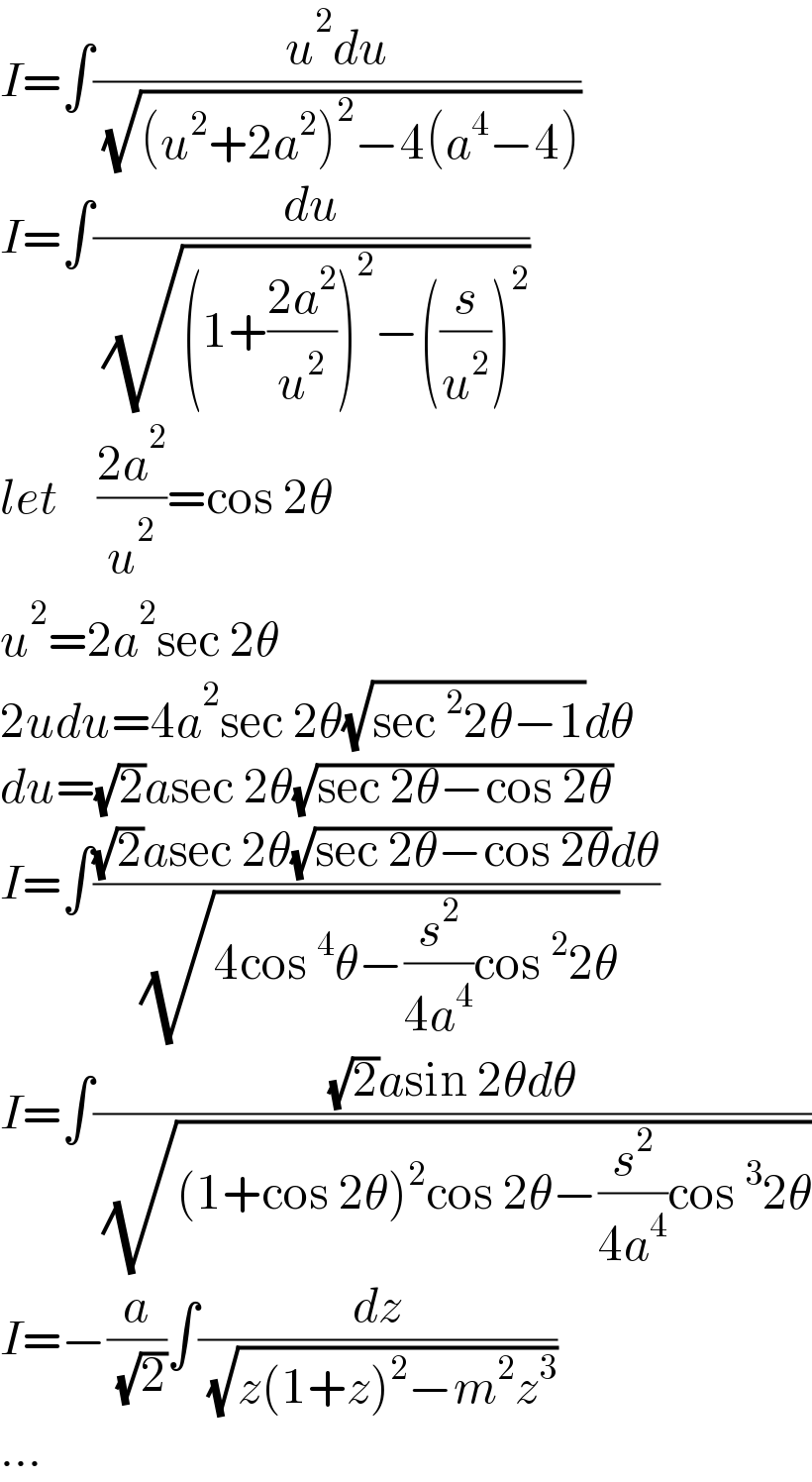
I=∫((u^2 du)/( (√((u^2 +2a^2 )^2 −4(a^4 −4))))) I=∫(du/( (√((1+((2a^2 )/u^2 ))^2 −((s/u^2 ))^2 )))) let ((2a^2 )/u^2 )=cos 2θ u^2 =2a^2 sec 2θ 2udu=4a^2 sec 2θ(√(sec^2 2θ−1))dθ du=(√2)asec 2θ(√(sec 2θ−cos 2θ)) I=∫(((√2)asec 2θ(√(sec 2θ−cos 2θ))dθ)/( (√(4cos^4 θ−(s^2 /(4a^4 ))cos^2 2θ)))) I=∫(((√2)asin 2θdθ)/( (√((1+cos 2θ)^2 cos 2θ−(s^2 /(4a^4 ))cos^3 2θ)))) I=−(a/( (√2)))∫(dz/( (√(z(1+z)^2 −m^2 z^3 )))) ...
$${I}=\int\frac{{u}^{\mathrm{2}} {du}}{\:\sqrt{\left({u}^{\mathrm{2}} +\mathrm{2}{a}^{\mathrm{2}} \right)^{\mathrm{2}} −\mathrm{4}\left({a}^{\mathrm{4}} −\mathrm{4}\right)}} \\ $$$${I}=\int\frac{{du}}{\:\sqrt{\left(\mathrm{1}+\frac{\mathrm{2}{a}^{\mathrm{2}} }{{u}^{\mathrm{2}} }\right)^{\mathrm{2}} −\left(\frac{{s}}{{u}^{\mathrm{2}} }\right)^{\mathrm{2}} }} \\ $$$${let}\:\:\:\:\frac{\mathrm{2}{a}^{\mathrm{2}} }{{u}^{\mathrm{2}} }=\mathrm{cos}\:\mathrm{2}\theta \\ $$$${u}^{\mathrm{2}} =\mathrm{2}{a}^{\mathrm{2}} \mathrm{sec}\:\mathrm{2}\theta \\ $$$$\mathrm{2}{udu}=\mathrm{4}{a}^{\mathrm{2}} \mathrm{sec}\:\mathrm{2}\theta\sqrt{\mathrm{sec}\:^{\mathrm{2}} \mathrm{2}\theta−\mathrm{1}}{d}\theta \\ $$$${du}=\sqrt{\mathrm{2}}{a}\mathrm{sec}\:\mathrm{2}\theta\sqrt{\mathrm{sec}\:\mathrm{2}\theta−\mathrm{cos}\:\mathrm{2}\theta} \\ $$$${I}=\int\frac{\sqrt{\mathrm{2}}{a}\mathrm{sec}\:\mathrm{2}\theta\sqrt{\mathrm{sec}\:\mathrm{2}\theta−\mathrm{cos}\:\mathrm{2}\theta}{d}\theta}{\:\sqrt{\mathrm{4cos}\:^{\mathrm{4}} \theta−\frac{{s}^{\mathrm{2}} }{\mathrm{4}{a}^{\mathrm{4}} }\mathrm{cos}\:^{\mathrm{2}} \mathrm{2}\theta}} \\ $$$${I}=\int\frac{\sqrt{\mathrm{2}}{a}\mathrm{sin}\:\mathrm{2}\theta{d}\theta}{\:\sqrt{\left(\mathrm{1}+\mathrm{cos}\:\mathrm{2}\theta\right)^{\mathrm{2}} \mathrm{cos}\:\mathrm{2}\theta−\frac{{s}^{\mathrm{2}} }{\mathrm{4}{a}^{\mathrm{4}} }\mathrm{cos}\:^{\mathrm{3}} \mathrm{2}\theta}} \\ $$$${I}=−\frac{{a}}{\:\sqrt{\mathrm{2}}}\int\frac{{dz}}{\:\sqrt{{z}\left(\mathrm{1}+{z}\right)^{\mathrm{2}} −{m}^{\mathrm{2}} {z}^{\mathrm{3}} }} \\ $$$$… \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *