Question Number 138656 by ajfour last updated on 16/Apr/21
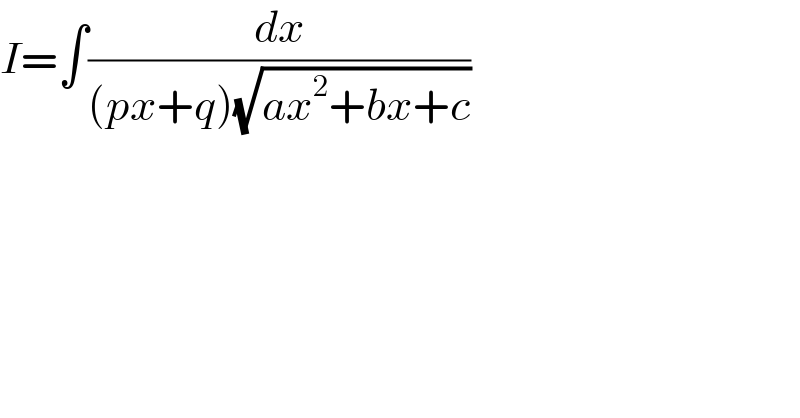
$${I}=\int\frac{{dx}}{\left({px}+{q}\right)\sqrt{{ax}^{\mathrm{2}} +{bx}+{c}}} \\ $$
Answered by Ar Brandon last updated on 16/Apr/21
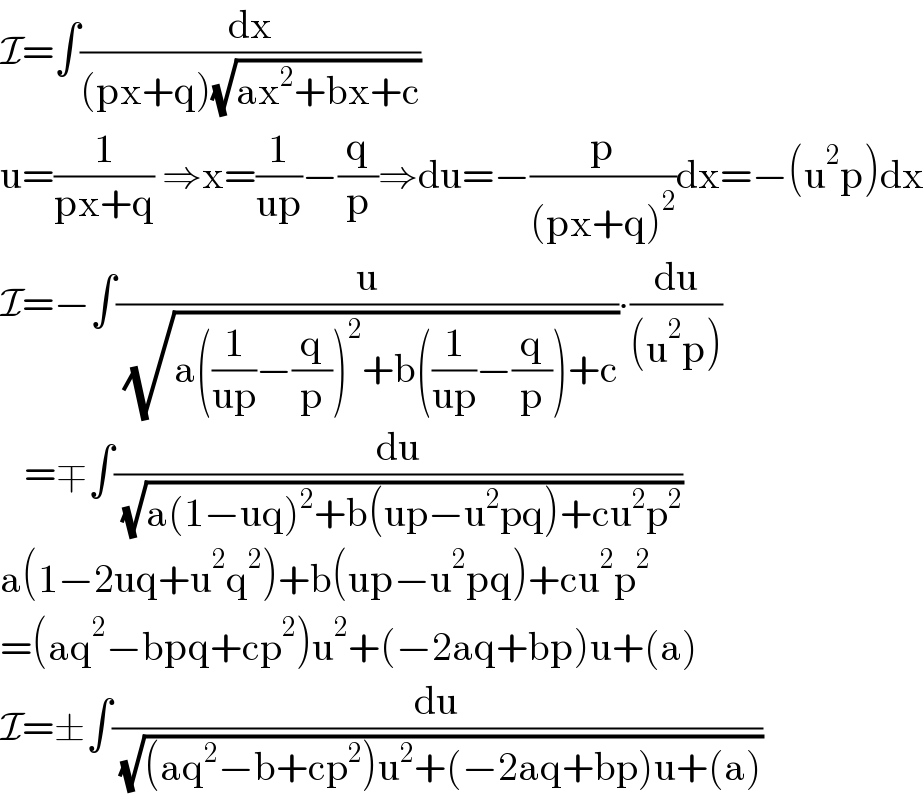
$$\mathcal{I}=\int\frac{\mathrm{dx}}{\left(\mathrm{px}+\mathrm{q}\right)\sqrt{\mathrm{ax}^{\mathrm{2}} +\mathrm{bx}+\mathrm{c}}} \\ $$$$\mathrm{u}=\frac{\mathrm{1}}{\mathrm{px}+\mathrm{q}}\:\Rightarrow\mathrm{x}=\frac{\mathrm{1}}{\mathrm{up}}−\frac{\mathrm{q}}{\mathrm{p}}\Rightarrow\mathrm{du}=−\frac{\mathrm{p}}{\left(\mathrm{px}+\mathrm{q}\right)^{\mathrm{2}} }\mathrm{dx}=−\left(\mathrm{u}^{\mathrm{2}} \mathrm{p}\right)\mathrm{dx} \\ $$$$\mathcal{I}=−\int\frac{\mathrm{u}}{\:\sqrt{\mathrm{a}\left(\frac{\mathrm{1}}{\mathrm{up}}−\frac{\mathrm{q}}{\mathrm{p}}\right)^{\mathrm{2}} +\mathrm{b}\left(\frac{\mathrm{1}}{\mathrm{up}}−\frac{\mathrm{q}}{\mathrm{p}}\right)+\mathrm{c}}}\centerdot\frac{\mathrm{du}}{\left(\mathrm{u}^{\mathrm{2}} \mathrm{p}\right)} \\ $$$$\:\:\:=\mp\int\frac{\mathrm{du}}{\:\sqrt{\mathrm{a}\left(\mathrm{1}−\mathrm{uq}\right)^{\mathrm{2}} +\mathrm{b}\left(\mathrm{up}−\mathrm{u}^{\mathrm{2}} \mathrm{pq}\right)+\mathrm{cu}^{\mathrm{2}} \mathrm{p}^{\mathrm{2}} }} \\ $$$$\mathrm{a}\left(\mathrm{1}−\mathrm{2uq}+\mathrm{u}^{\mathrm{2}} \mathrm{q}^{\mathrm{2}} \right)+\mathrm{b}\left(\mathrm{up}−\mathrm{u}^{\mathrm{2}} \mathrm{pq}\right)+\mathrm{cu}^{\mathrm{2}} \mathrm{p}^{\mathrm{2}} \\ $$$$=\left(\mathrm{aq}^{\mathrm{2}} −\mathrm{bpq}+\mathrm{cp}^{\mathrm{2}} \right)\mathrm{u}^{\mathrm{2}} +\left(−\mathrm{2aq}+\mathrm{bp}\right)\mathrm{u}+\left(\mathrm{a}\right) \\ $$$$\mathcal{I}=\pm\int\frac{\mathrm{du}}{\:\sqrt{\left(\mathrm{aq}^{\mathrm{2}} −\mathrm{b}+\mathrm{cp}^{\mathrm{2}} \right)\mathrm{u}^{\mathrm{2}} +\left(−\mathrm{2aq}+\mathrm{bp}\right)\mathrm{u}+\left(\mathrm{a}\right)}} \\ $$
