Question Number 5546 by FilupSmith last updated on 19/May/16

Commented by FilupSmith last updated on 19/May/16
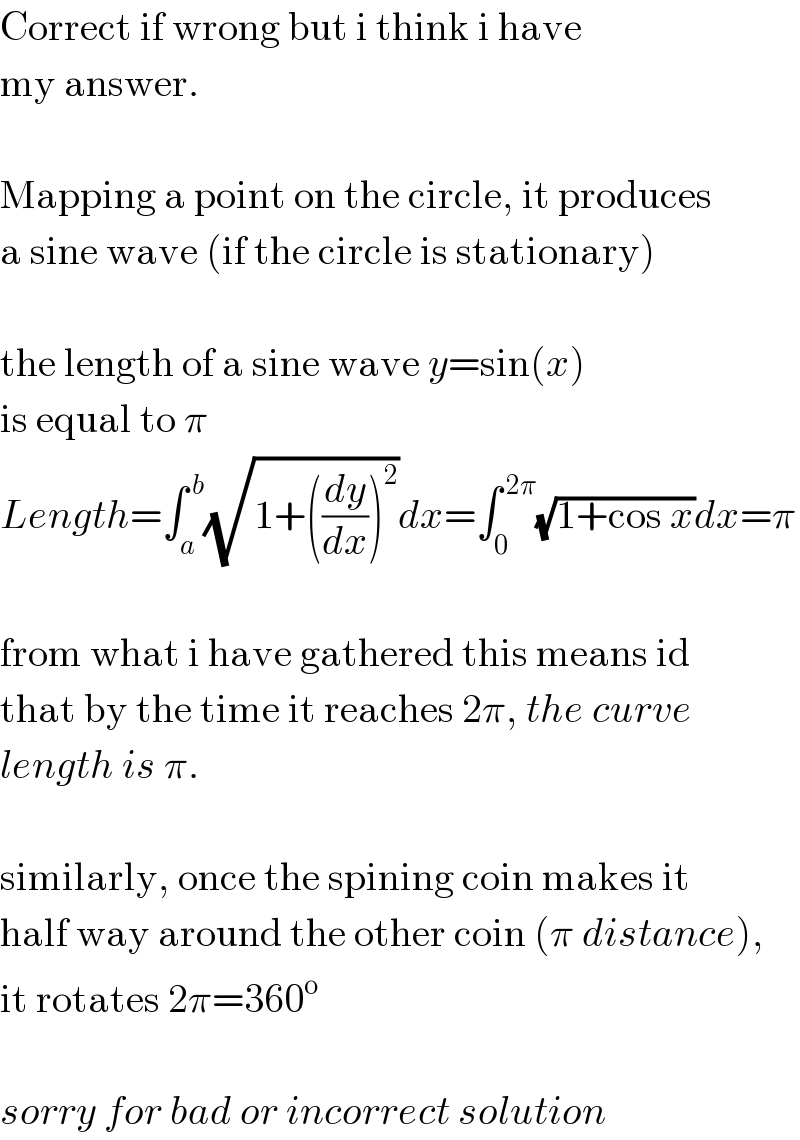
Commented by Yozzii last updated on 20/May/16
![The integral expression for the arc length of the sine curve cannot be expressed in terms of elementary functions. This is because no such result can be the antiderivative of (√(1+cos^2 x)). For the closed interval [0,2π] the arc length ∫_0 ^(2π) (√(1+cos^2 x))dx≈7.6≠π.](https://www.tinkutara.com/question/Q5555.png)
Commented by FilupSmith last updated on 19/May/16

Commented by Yozzii last updated on 19/May/16
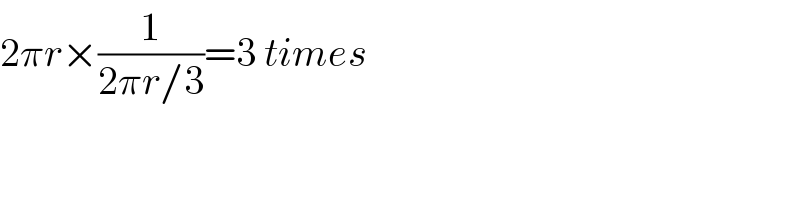
Commented by Yozzii last updated on 19/May/16

Commented by FilupSmith last updated on 19/May/16

Commented by Yozzii last updated on 19/May/16
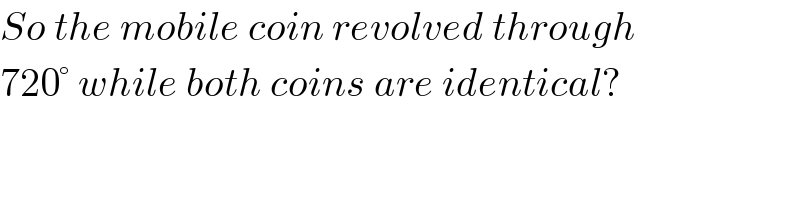
Commented by FilupSmith last updated on 19/May/16

Commented by FilupSmith last updated on 19/May/16
Commented by FilupSmith last updated on 20/May/16

Commented by Yozzii last updated on 20/May/16

Commented by Yozzii last updated on 20/May/16

Commented by Yozzii last updated on 20/May/16

