Question Number 134475 by EDWIN88 last updated on 04/Mar/21

Commented by Dwaipayan Shikari last updated on 04/Mar/21
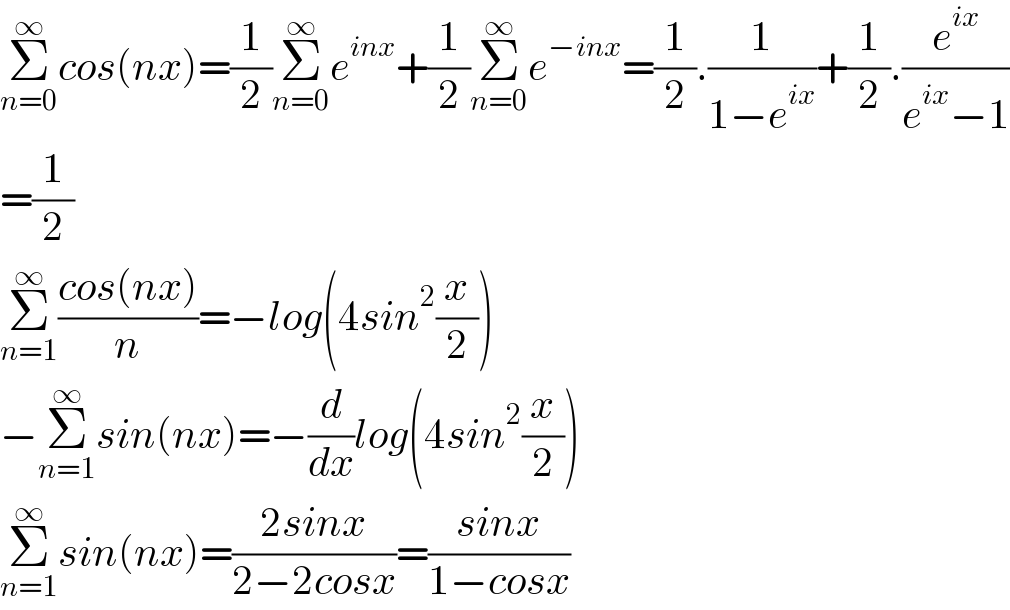
Commented by benjo_mathlover last updated on 04/Mar/21
Commented by Dwaipayan Shikari last updated on 04/Mar/21
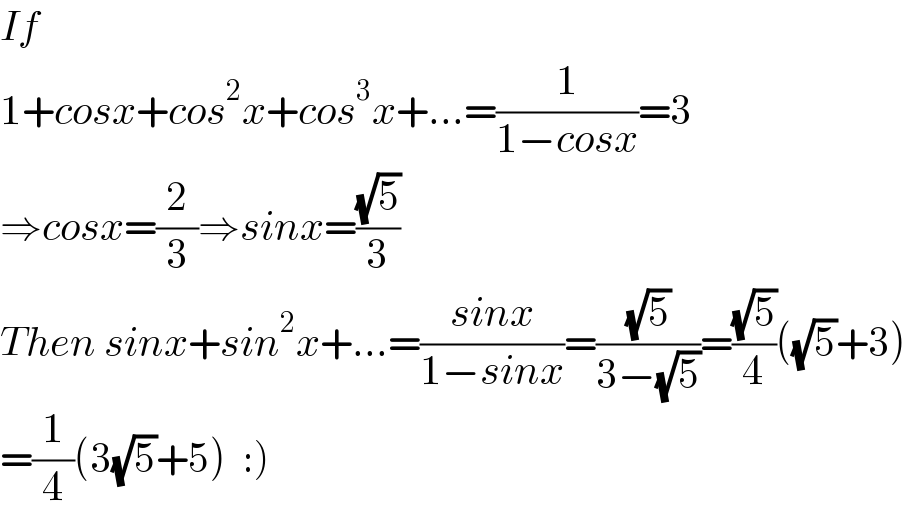
Commented by EDWIN88 last updated on 04/Mar/21
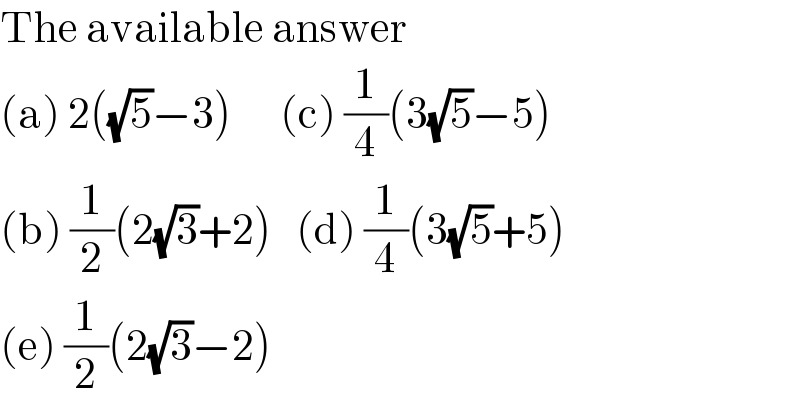
Commented by Dwaipayan Shikari last updated on 04/Mar/21
Commented by EDWIN88 last updated on 04/Mar/21

