Menu Close

If-and-are-the-roots-of-x-2-x-1-0-Find-23-23-without-demoivre-s-theorem-

Tinku Tara 0 Comments
Pin




Question Number 74742 by TawaTawa last updated on 30/Nov/19
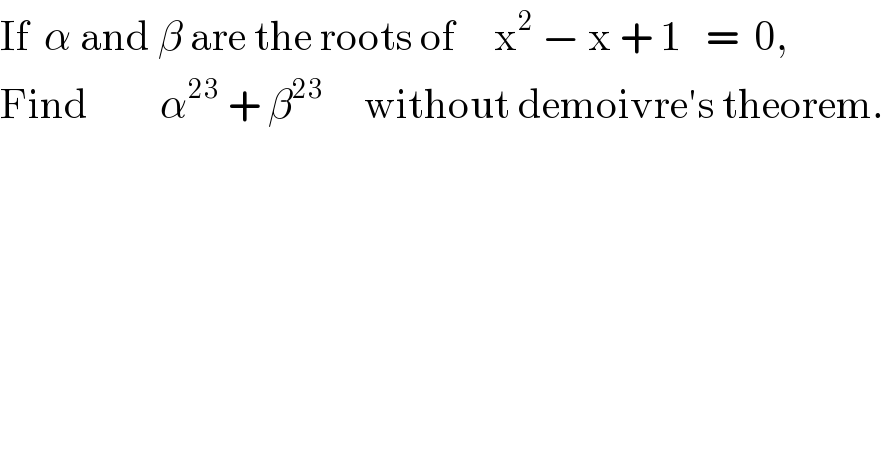
If α and β are the roots of x^2 − x + 1 = 0, Find α^(23) + β^(23) without demoivre′s theorem.
$$\mathrm{If}\:\:\alpha\:\mathrm{and}\:\beta\:\mathrm{are}\:\mathrm{the}\:\mathrm{roots}\:\mathrm{of}\:\:\:\:\:\mathrm{x}^{\mathrm{2}} \:−\:\mathrm{x}\:+\:\mathrm{1}\:\:\:=\:\:\mathrm{0},\:\: \\ $$$$\mathrm{Find}\:\:\:\:\:\:\:\:\:\alpha^{\mathrm{23}} \:+\:\beta^{\mathrm{23}} \:\:\:\:\:\mathrm{without}\:\mathrm{demoivre}'\mathrm{s}\:\mathrm{theorem}. \\ $$
Commented by abdomathmax last updated on 02/Dec/19
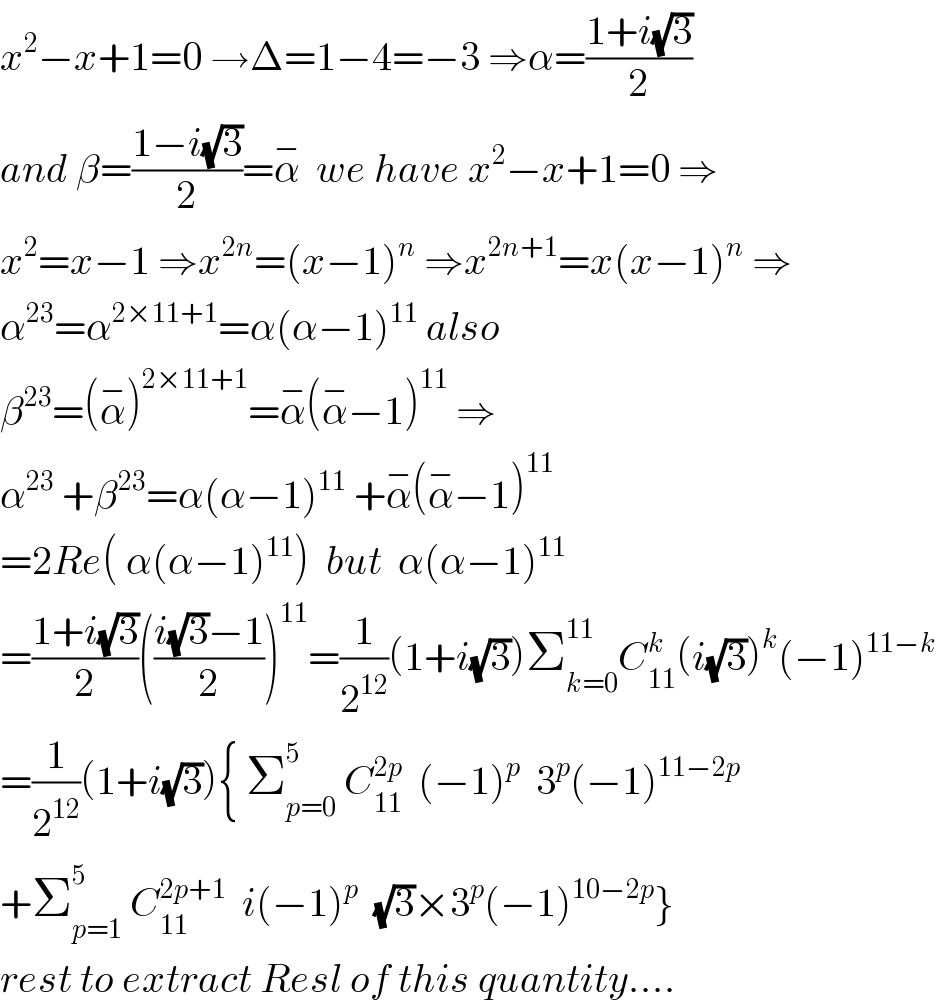
x^2 −x+1=0 →Δ=1−4=−3 ⇒α=((1+i(√3))/2) and β=((1−i(√3))/2)=α^− we have x^2 −x+1=0 ⇒ x^2 =x−1 ⇒x^(2n) =(x−1)^n ⇒x^(2n+1) =x(x−1)^n ⇒ α^(23) =α^(2×11+1) =α(α−1)^(11) also β^(23) =(α^− )^(2×11+1) =α^− (α^− −1)^(11) ⇒ α^(23) +β^(23) =α(α−1)^(11) +α^− (α^− −1)^(11) =2Re( α(α−1)^(11) ) but α(α−1)^(11) =((1+i(√3))/2)(((i(√3)−1)/2))^(11) =(1/2^(12) )(1+i(√3))Σ_(k=0) ^(11) C_(11) ^k (i(√3))^k (−1)^(11−k) =(1/2^(12) )(1+i(√3)){ Σ_(p=0) ^5 C_(11) ^(2p) (−1)^p 3^p (−1)^(11−2p) +Σ_(p=1) ^5 C_(11) ^(2p+1) i(−1)^p (√3)×3^p (−1)^(10−2p) } rest to extract Resl of this quantity....
$${x}^{\mathrm{2}} −{x}+\mathrm{1}=\mathrm{0}\:\rightarrow\Delta=\mathrm{1}−\mathrm{4}=−\mathrm{3}\:\Rightarrow\alpha=\frac{\mathrm{1}+{i}\sqrt{\mathrm{3}}}{\mathrm{2}} \\ $$$${and}\:\beta=\frac{\mathrm{1}−{i}\sqrt{\mathrm{3}}}{\mathrm{2}}=\overset{−} {\alpha}\:\:{we}\:{have}\:{x}^{\mathrm{2}} −{x}+\mathrm{1}=\mathrm{0}\:\Rightarrow \\ $$$${x}^{\mathrm{2}} ={x}−\mathrm{1}\:\Rightarrow{x}^{\mathrm{2}{n}} =\left({x}−\mathrm{1}\right)^{{n}} \:\Rightarrow{x}^{\mathrm{2}{n}+\mathrm{1}} ={x}\left({x}−\mathrm{1}\right)^{{n}} \:\Rightarrow \\ $$$$\alpha^{\mathrm{23}} =\alpha^{\mathrm{2}×\mathrm{11}+\mathrm{1}} =\alpha\left(\alpha−\mathrm{1}\right)^{\mathrm{11}} \:{also} \\ $$$$\beta^{\mathrm{23}} =\left(\overset{−} {\alpha}\right)^{\mathrm{2}×\mathrm{11}+\mathrm{1}} =\overset{−} {\alpha}\left(\overset{−} {\alpha}−\mathrm{1}\right)^{\mathrm{11}} \:\Rightarrow \\ $$$$\alpha^{\mathrm{23}} \:+\beta^{\mathrm{23}} =\alpha\left(\alpha−\mathrm{1}\right)^{\mathrm{11}} \:+\overset{−} {\alpha}\left(\overset{−} {\alpha}−\mathrm{1}\right)^{\mathrm{11}} \\ $$$$=\mathrm{2}{Re}\left(\:\alpha\left(\alpha−\mathrm{1}\right)^{\mathrm{11}} \right)\:\:{but}\:\:\alpha\left(\alpha−\mathrm{1}\right)^{\mathrm{11}} \\ $$$$=\frac{\mathrm{1}+{i}\sqrt{\mathrm{3}}}{\mathrm{2}}\left(\frac{{i}\sqrt{\mathrm{3}}−\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{11}} =\frac{\mathrm{1}}{\mathrm{2}^{\mathrm{12}} }\left(\mathrm{1}+{i}\sqrt{\mathrm{3}}\right)\sum_{{k}=\mathrm{0}} ^{\mathrm{11}} {C}_{\mathrm{11}} ^{{k}} \left({i}\sqrt{\mathrm{3}}\right)^{{k}} \left(−\mathrm{1}\right)^{\mathrm{11}−{k}} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}^{\mathrm{12}} }\left(\mathrm{1}+{i}\sqrt{\mathrm{3}}\right)\left\{\:\sum_{{p}=\mathrm{0}} ^{\mathrm{5}} \:{C}_{\mathrm{11}} ^{\mathrm{2}{p}} \:\:\left(−\mathrm{1}\right)^{{p}} \:\:\mathrm{3}^{{p}} \left(−\mathrm{1}\right)^{\mathrm{11}−\mathrm{2}{p}} \right. \\ $$$$\left.+\sum_{{p}=\mathrm{1}} ^{\mathrm{5}} \:{C}_{\mathrm{11}} ^{\mathrm{2}{p}+\mathrm{1}} \:\:{i}\left(−\mathrm{1}\right)^{{p}} \:\:\sqrt{\mathrm{3}}×\mathrm{3}^{{p}} \left(−\mathrm{1}\right)^{\mathrm{10}−\mathrm{2}{p}} \right\} \\ $$$${rest}\:{to}\:{extract}\:{Resl}\:{of}\:{this}\:{quantity}…. \\ $$
Answered by Smail last updated on 30/Nov/19
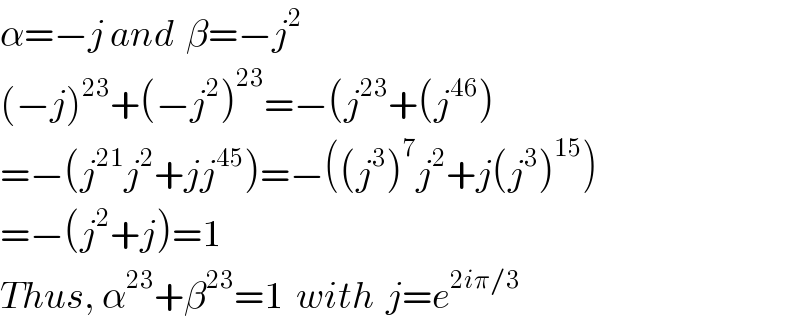
α=−j and β=−j^2 (−j)^(23) +(−j^2 )^(23) =−(j^(23) +(j^(46) ) =−(j^(21) j^2 +jj^(45) )=−((j^3 )^7 j^2 +j(j^3 )^(15) ) =−(j^2 +j)=1 Thus, α^(23) +β^(23) =1 with j=e^(2iπ/3)
$$\alpha=−{j}\:{and}\:\:\beta=−{j}^{\mathrm{2}} \\ $$$$\left(−{j}\right)^{\mathrm{23}} +\left(−{j}^{\mathrm{2}} \right)^{\mathrm{23}} =−\left({j}^{\mathrm{23}} +\left({j}^{\mathrm{46}} \right)\right. \\ $$$$=−\left({j}^{\mathrm{21}} {j}^{\mathrm{2}} +{jj}^{\mathrm{45}} \right)=−\left(\left({j}^{\mathrm{3}} \right)^{\mathrm{7}} {j}^{\mathrm{2}} +{j}\left({j}^{\mathrm{3}} \right)^{\mathrm{15}} \right) \\ $$$$=−\left({j}^{\mathrm{2}} +{j}\right)=\mathrm{1} \\ $$$${Thus},\:\alpha^{\mathrm{23}} +\beta^{\mathrm{23}} =\mathrm{1}\:\:{with}\:\:{j}={e}^{\mathrm{2}{i}\pi/\mathrm{3}} \\ $$
Commented by TawaTawa last updated on 30/Nov/19
God bless you sir
$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir} \\ $$
Commented by TawaTawa last updated on 30/Nov/19
But is it possible without complex number and cube root of unity
$$\mathrm{But}\:\mathrm{is}\:\mathrm{it}\:\mathrm{possible}\:\mathrm{without}\:\mathrm{complex}\:\mathrm{number}\:\mathrm{and} \\ $$$$\mathrm{cube}\:\mathrm{root}\:\mathrm{of}\:\mathrm{unity} \\ $$
Answered by mind is power last updated on 30/Nov/19
(x+1)(x^2 −x+1)=x^3 +1=0 ⇒α^3 =β^3 =−1 ⇒∀k∈N α^3 =β^3 =(−1)^k =1 if k≡0(2),−1 else 23=3.7+2 ⇒α^(3.7+2) +β^(3.7+2) =(−1).α^2 −(β)^2 =−α^2 −β^2 α^2 =−1+α ,β=−1+β =−(α+β)+2 α+β=−((−1)/1)=1,som of root =−1+2=1
$$\left(\mathrm{x}+\mathrm{1}\right)\left(\mathrm{x}^{\mathrm{2}} −\mathrm{x}+\mathrm{1}\right)=\mathrm{x}^{\mathrm{3}} +\mathrm{1}=\mathrm{0} \\ $$$$\Rightarrow\alpha^{\mathrm{3}} =\beta^{\mathrm{3}} =−\mathrm{1} \\ $$$$\Rightarrow\forall\mathrm{k}\in\mathbb{N}\:\:\:\alpha^{\mathrm{3}} =\beta^{\mathrm{3}} =\left(−\mathrm{1}\right)^{\mathrm{k}} =\mathrm{1}\:\mathrm{if}\:\mathrm{k}\equiv\mathrm{0}\left(\mathrm{2}\right),−\mathrm{1}\:\:\mathrm{else} \\ $$$$\mathrm{23}=\mathrm{3}.\mathrm{7}+\mathrm{2} \\ $$$$\Rightarrow\alpha^{\mathrm{3}.\mathrm{7}+\mathrm{2}} +\beta^{\mathrm{3}.\mathrm{7}+\mathrm{2}} \\ $$$$=\left(−\mathrm{1}\right).\alpha^{\mathrm{2}} −\left(\beta\right)^{\mathrm{2}} =−\alpha^{\mathrm{2}} −\beta^{\mathrm{2}} \\ $$$$\alpha^{\mathrm{2}} =−\mathrm{1}+\alpha\:\:,\beta=−\mathrm{1}+\beta \\ $$$$=−\left(\alpha+\beta\right)+\mathrm{2} \\ $$$$\alpha+\beta=−\frac{−\mathrm{1}}{\mathrm{1}}=\mathrm{1},\mathrm{som}\:\mathrm{of}\:\mathrm{root} \\ $$$$=−\mathrm{1}+\mathrm{2}=\mathrm{1} \\ $$$$ \\ $$
Commented by TawaTawa last updated on 30/Nov/19
God bless you sir.
$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir}. \\ $$
Commented by TawaTawa last updated on 30/Nov/19
Can we use it for: x^2 − 2x + 4 = 0 Find α^(23) + β^(23)
$$\mathrm{Can}\:\mathrm{we}\:\mathrm{use}\:\mathrm{it}\:\mathrm{for}: \\ $$$$\:\:\:\:\:\mathrm{x}^{\mathrm{2}} \:−\:\mathrm{2x}\:+\:\mathrm{4}\:\:=\:\:\mathrm{0} \\ $$$$\mathrm{Find}\:\:\:\:\:\alpha^{\mathrm{23}} \:+\:\beta^{\mathrm{23}} \\ $$
Commented by mind is power last updated on 30/Nov/19
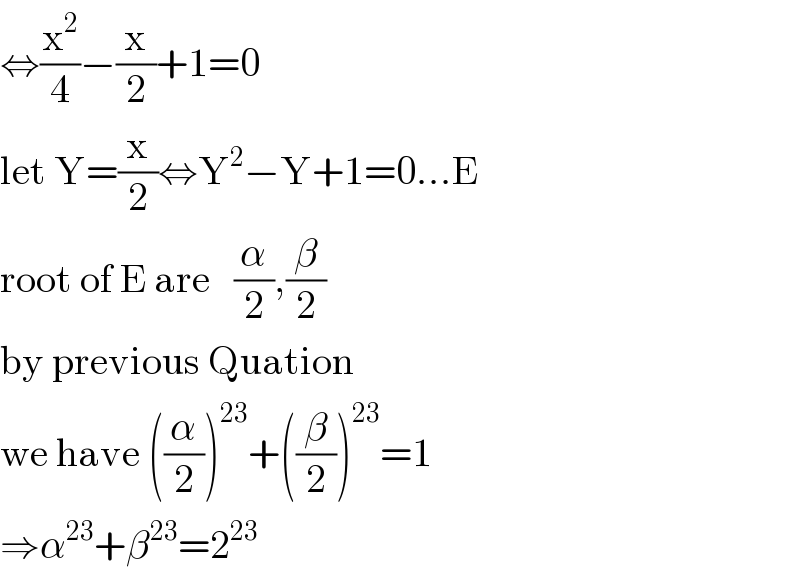
⇔(x^2 /4)−(x/2)+1=0 let Y=(x/2)⇔Y^2 −Y+1=0...E root of E are (α/2),(β/2) by previous Quation we have ((α/2))^(23) +((β/2))^(23) =1 ⇒α^(23) +β^(23) =2^(23)
$$\Leftrightarrow\frac{\mathrm{x}^{\mathrm{2}} }{\mathrm{4}}−\frac{\mathrm{x}}{\mathrm{2}}+\mathrm{1}=\mathrm{0} \\ $$$$\mathrm{let}\:\mathrm{Y}=\frac{\mathrm{x}}{\mathrm{2}}\Leftrightarrow\mathrm{Y}^{\mathrm{2}} −\mathrm{Y}+\mathrm{1}=\mathrm{0}…\mathrm{E} \\ $$$$\mathrm{root}\:\mathrm{of}\:\mathrm{E}\:\mathrm{are}\:\:\:\frac{\alpha}{\mathrm{2}},\frac{\beta}{\mathrm{2}} \\ $$$$\mathrm{by}\:\mathrm{previous}\:\mathrm{Quation} \\ $$$$\mathrm{we}\:\mathrm{have}\:\left(\frac{\alpha}{\mathrm{2}}\right)^{\mathrm{23}} +\left(\frac{\beta}{\mathrm{2}}\right)^{\mathrm{23}} =\mathrm{1} \\ $$$$\Rightarrow\alpha^{\mathrm{23}} +\beta^{\mathrm{23}} =\mathrm{2}^{\mathrm{23}} \\ $$
Commented by TawaTawa last updated on 30/Nov/19
God bless you sir
$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir} \\ $$
Commented by TawaTawa last updated on 30/Nov/19
I appreciate sir.
$$\mathrm{I}\:\mathrm{appreciate}\:\mathrm{sir}. \\ $$
Commented by TawaTawa last updated on 30/Nov/19
Sir, is there any way this method cannot be used appart from complex number ? Or the method can work for any question ??
$$\mathrm{Sir},\:\:\mathrm{is}\:\mathrm{there}\:\mathrm{any}\:\mathrm{way}\:\mathrm{this}\:\mathrm{method}\:\mathrm{cannot}\:\mathrm{be}\:\mathrm{used}\:\mathrm{appart} \\ $$$$\mathrm{from}\:\mathrm{complex}\:\mathrm{number}\:? \\ $$$$\mathrm{Or}\:\mathrm{the}\:\mathrm{method}\:\mathrm{can}\:\mathrm{work}\:\mathrm{for}\:\mathrm{any}\:\mathrm{question}\:?? \\ $$
Commented by TawaTawa last updated on 30/Nov/19
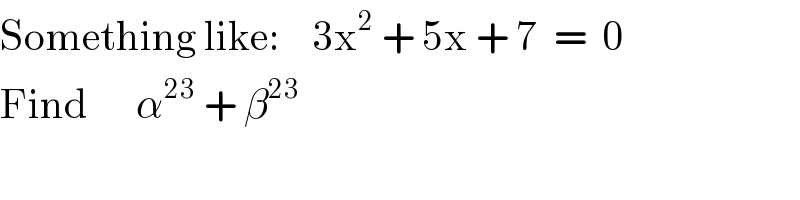
Something like: 3x^2 + 5x + 7 = 0 Find α^(23) + β^(23)
$$\mathrm{Something}\:\mathrm{like}:\:\:\:\:\mathrm{3x}^{\mathrm{2}} \:+\:\mathrm{5x}\:+\:\mathrm{7}\:\:=\:\:\mathrm{0} \\ $$$$\mathrm{Find}\:\:\:\:\:\:\alpha^{\mathrm{23}} \:+\:\beta^{\mathrm{23}} \\ $$
Commented by mind is power last updated on 30/Nov/19
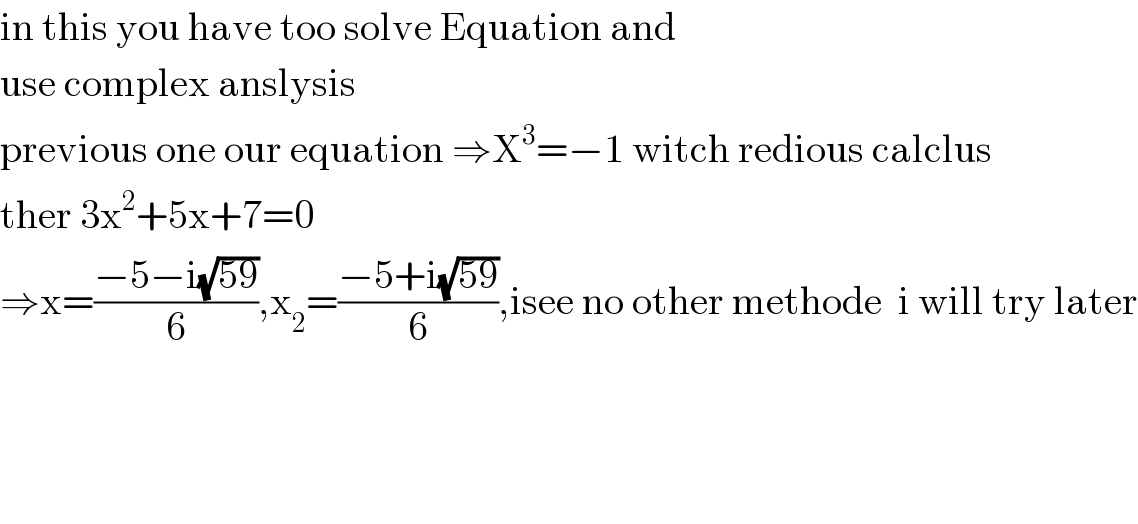
in this you have too solve Equation and use complex anslysis previous one our equation ⇒X^3 =−1 witch redious calclus ther 3x^2 +5x+7=0 ⇒x=((−5−i(√(59)))/6),x_2 =((−5+i(√(59)))/6),isee no other methode i will try later
$$\mathrm{in}\:\mathrm{this}\:\mathrm{you}\:\mathrm{have}\:\mathrm{too}\:\mathrm{solve}\:\mathrm{Equation}\:\mathrm{and}\: \\ $$$$\mathrm{use}\:\mathrm{complex}\:\mathrm{anslysis} \\ $$$$\mathrm{previous}\:\mathrm{one}\:\mathrm{our}\:\mathrm{equation}\:\Rightarrow\mathrm{X}^{\mathrm{3}} =−\mathrm{1}\:\mathrm{witch}\:\mathrm{redious}\:\mathrm{calclus} \\ $$$$\mathrm{ther}\:\mathrm{3x}^{\mathrm{2}} +\mathrm{5x}+\mathrm{7}=\mathrm{0} \\ $$$$\Rightarrow\mathrm{x}=\frac{−\mathrm{5}−\mathrm{i}\sqrt{\mathrm{59}}}{\mathrm{6}},\mathrm{x}_{\mathrm{2}} =\frac{−\mathrm{5}+\mathrm{i}\sqrt{\mathrm{59}}}{\mathrm{6}},\mathrm{isee}\:\mathrm{no}\:\mathrm{other}\:\mathrm{methode}\:\:\mathrm{i}\:\mathrm{will}\:\mathrm{try}\:\mathrm{later} \\ $$$$ \\ $$$$ \\ $$$$ \\ $$
Commented by TawaTawa last updated on 30/Nov/19
I can use complex number Help me find other method sir
$$\mathrm{I}\:\mathrm{can}\:\mathrm{use}\:\mathrm{complex}\:\mathrm{number} \\ $$$$\mathrm{Help}\:\mathrm{me}\:\mathrm{find}\:\mathrm{other}\:\mathrm{method}\:\mathrm{sir} \\ $$
Commented by TawaTawa last updated on 30/Nov/19
God bless you sir
$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *