If-gcd-p-q-1-prove-that-gcd-p-p-q-q-p-q-pq-1-Related-to-Q-69939-

Question Number 70370 by Rasheed.Sindhi last updated on 04/Oct/19

I f g c d (p, q) = 1, p r o v e t h a t

g c d (p (p + q), q (p + q), p q) = 1

You can't use 'macro parameter character #' in math mode

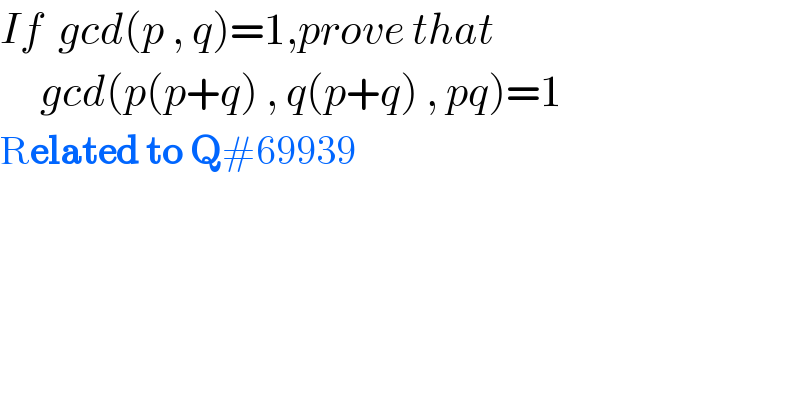
Commented by mind is power last updated on 03/Oct/19

s u p p o s e T h a t a = g c d (p (p + q), q (p + q), p q) \neq 1

\Rightarrow \exists p r i m e n u m b e r x t h a t x ∣ a

\Rightarrow x ∣ p q, x ∣ q (p + q), x ∣ p (p + q)

x ∣ p q + q^{2}, x ∣ p q + p^{2}, x ∣ p q

\Rightarrow x ∣ q^{2} \Rightarrow x ∣ q x i s p r i m e

x ∣ p^{2} \Rightarrow x ∣ p

(x ∣ p \land x ∣ q) \Leftrightarrow x ∣ g c d (p, q) = 1 \Rightarrow x ∣ 1 a b s u r d x i s p r i m e

\Rightarrow t h e r e x i s t n o t p r i m e f a c t o r o f a \in N \Leftrightarrow (a = 1)

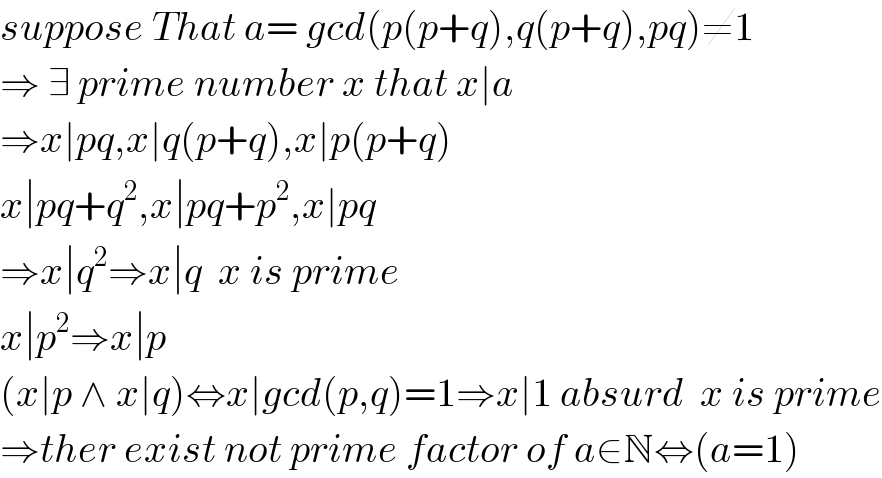
Commented by MJS last updated on 03/Oct/19

good, but I think the last line should be

x ∣ p \land x ∣ q \land \gcd (p, q) = 1 \Rightarrow x = 1

because obviously

\gcd (p, q) = r \land x ∣ p \land x ∣ q \Rightarrow x ⩽ r

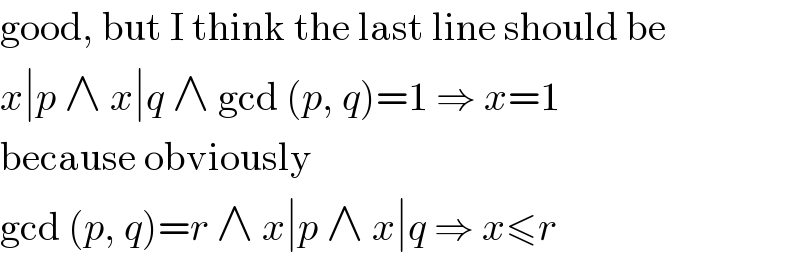
Commented by mind is power last updated on 03/Oct/19

x ∣ a \land x ∣ b \Leftrightarrow x ∣ g c d (a, b)

i f x ∣ g c d (a, b) \Rightarrow x ∣ a \land x ∣ b

i f x ∣ a \land x ∣ b \Rightarrow x ∣ g c d (a, b)

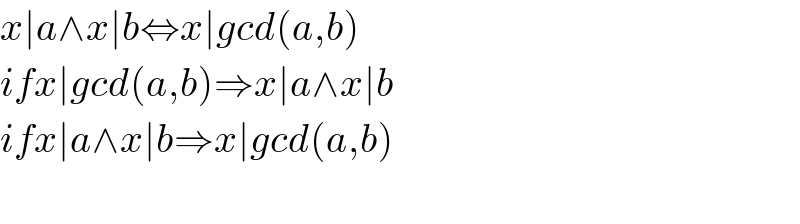
Commented by Rasheed.Sindhi last updated on 04/Oct/19

S ir, T hanks & A ppreciation \to you!

Answered by $@ty@m123 last updated on 04/Oct/19

I f p o s s i b l e l e t u s a s s u m e t h a t

g c d {p (p + q), q (p + q), p q} = a \neq 1

T h e n \exists m, n, r \in N w i t h g c d (m, n, r) = 1

s u c h t h a t

p (p + q) = a . m___(1)

q (p + q) = a . n___(2)

p q = a . r___(3)

F r o m (1) & (3)

p^{2} + a r = a m

p^{2} = a (m - r)___(4)

F r o m (2) & (3)

a r + q^{2} = a n

q^{2} = a (n - r)___(5)

(4) & (5) \Rightarrow p^{2} ∣ a \land q^{2} ∣ a

\Rightarrow p ∣ a \land q ∣ a

\Rightarrow g c d (p, q) = a \neq 1

w h i c h i s a c o n t r a d i c t i o n .

∴ o u r a s s u m p t i o n i s w r o n g .

∴ g c d {p (p + q), q (p + q), p q} = 1

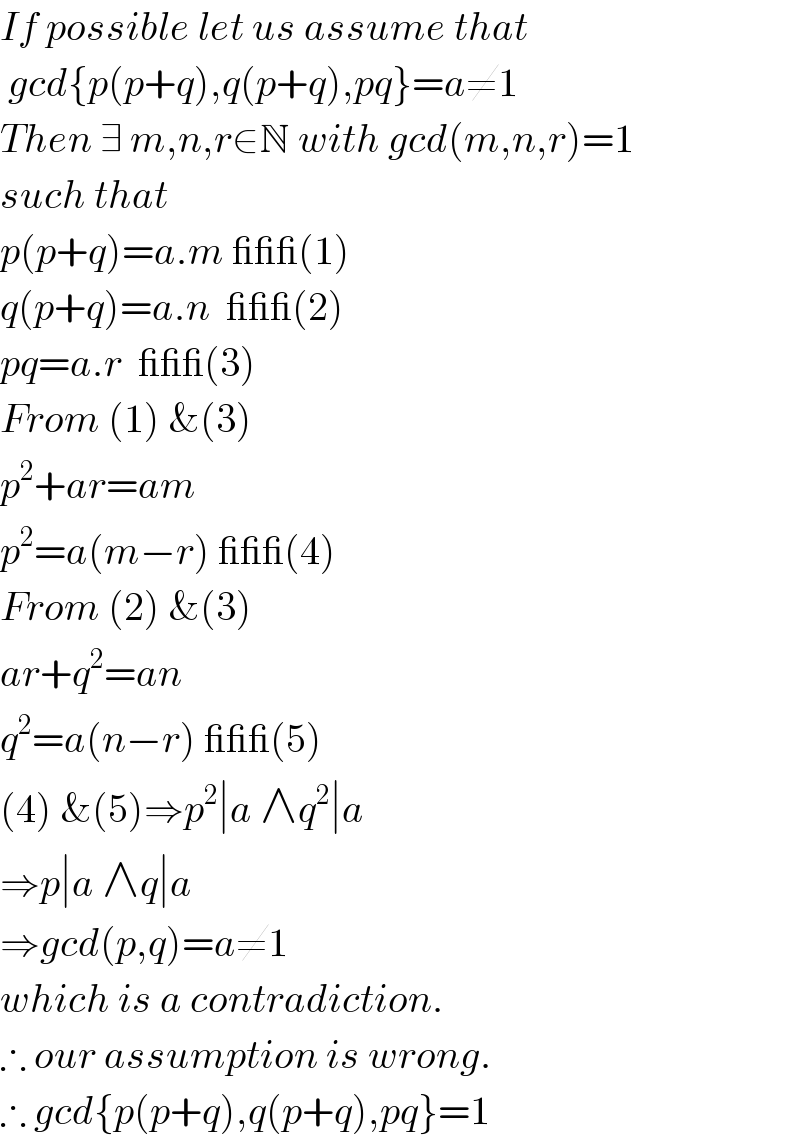
Commented by Rasheed.Sindhi last updated on 04/Oct/19

T h a n k y o u s i r . I a p p r i c i a t e y o u r a p p r o a c h!